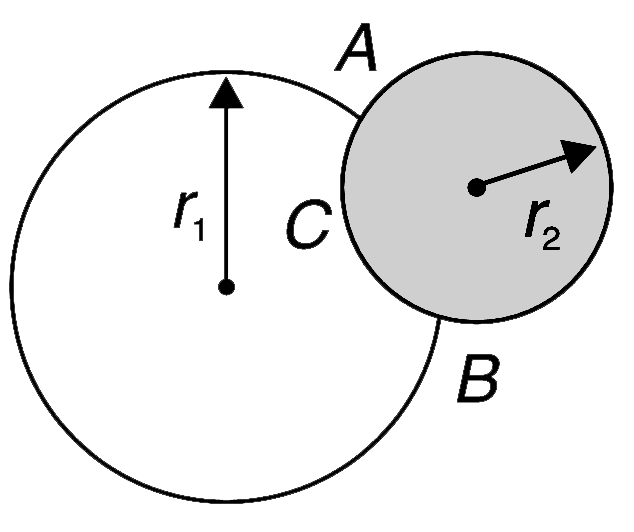
Two soap bubbles are stuck together with an intermediate film separating them. Compute the radius of curvature of this film given that the radii of the bubbles in this arrangement are $r_{1}$ and $r_{2}$ respectively. If $r_{1} > r_{2}$ state clearly which way the intermediate film will bulge.
For the case when $r_{1} = r_{2} = 2 c m$ calculate he radius of the bubble formed by bursting the intermediate film. The volume of a spherical dome of radius $R$ and height $h$ is $π b^{2} (3 R - b) / 3$ .

Video Solution

Text Solution

Verified by Experts

Excess pressure inside a soap bubble is $4 T / r$
If $P$ is the atmospheric pressure
$P_{1} = \frac{4 T}{r_{1}} = P$ , $P_{2} = \frac{4 T}{r_{2}} - P$
$∴ P_{1} - P_{2} = 4 T (\frac{1}{r_{1}} - \frac{1}{r_{2}}) = \frac{4 T}{r_{12}}$
$∴ \frac{1}{r_{12}} = \frac{1}{r_{1}} - \frac{1}{r_{2}} \Rightarrow r_{12} = \frac{r_{1} r_{2}}{| r_{1} - r_{2} |}$
If $r_{1} > r_{2}$ , $P_{2} > P_{1}$ and the intermediate film bulges towards the centre of circle of radius $r_{1}$ .

If $r_{1} = r_{2} = 2 c m$ , the intermediate film is a plane symmetrical positioned. The $3$ films due to the two bubbles and the intermediate film meet at a common circle with diameter $A B$ . These $3$ films produce $3$ forces at every point of this circle.
Since the surface tension $T$ is the same for all the films,
$| F_{1} | = | F_{2} | = | F_{12} |$
Equilibrium at any point such as $A$ is possible only if the three forces are at $120^{\circ}$ to one antoher.
$∴ O_{1} C = \frac{1}{2} r$
$b = r + \frac{1}{2} r = \frac{3}{2} r$
Volume of each bubble before bursting $= \frac{π}{3} {(\frac{3}{2} r)}^{2} (3 t - \frac{3}{2} r) = \frac{9 π r^{4}}{8}$
If $R$ is the radius of the combined bubble after bursting the intermediate film,
$\frac{4 π R^{3}}{3} = 2 \times \frac{9 π r^{3}}{8} = \frac{9 π r^{3}}{4}$

Updated on:21/07/2023