A large vessel is filled with a liquid. Two vertical plates touch the surlace of the liquid (Fig. 25.7). The dimensions of the plates are a and b, the distance between them is d. The plates have been charged by applying a voltage Po and then disconnected from the voltage source. To what height will the liquid rise? Ignore capillary effects.
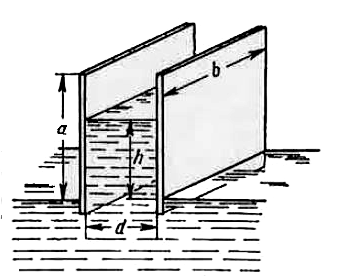
When the liquid flows through a strongly non-uniform electric field close to the edges of the plates, it is polarized and drawn into the space between the plates. Since the charge on the plates remains unchanged in the process, and the capacitance of the capacitor increases, this is accompanied by a decrease in the energy of the field. This decrease is compensated by the increase in the potential energy of the column of líquid held between the plates. From the law of conservation of energy
q22C0=q22C+mgh2
Here C0=ε0abd,C=ε0bd[a+(ε−1)h] (see Problem 25.5) m=ρbhd. Substituting these values into the first formula we obtain, after some simplifications,
(ε−1)q2=ε0ρgab2[a+(ε−1)h]
Express the charge on the plates in terms of the potential,
q=φ0C0=ε0φ0ab/d
After simplifications we obtain a quadratic equation
h2+aε−1h−ε0aφ20ρgd2=0
Solving it we obtain the height the liquid rises.
Similar Questions
Two parallel glass plates are dipped partly in the liquid of density 'd' keeping them vertical. If the distance between the plates is 'x', Surface tension is T and angle of contact is θ then rise of liquid between the plates due to capillary will be
View SolutionA parallel plate capcitor is formed by two discs with a unifrom poorly conducting medium between them. The capacitor was initally charged and then disconnected form a voltage source. Neglecting the edge effects, show that there is no magnetic field between capcitor plates.
View Solution
Knowledge Check
Two parallel glass plates are dipped partly in the liquid of denstiy 'd' keeping them vertical. If the distance between the plates is 'x', Surface tension is T and angle of contact is θ then ries of liquid between the plates due to capillary will be
ATcosθxdB2TcosθxdgC2TxdgcosθDtcosθxdgTwo parallel glass plates separated by a small distance x are dipped partly in a liquid of density " d" keeping them vertical .The surface tension of the liquid is T and angle of contact is θ . What is the rise of the liquid between the plates due to capillarity ?
ATcosθxdB2TcosθxdgC2TxdgcosθDTcosθxdg
A parallel-plate capacitor with vertical plates is connected to a voltage source and then submerged into a liquid dielectric. How does the level of the dielectric between the plates change in the process? Explain the change of the energy stored by the capacitor
View SolutionTwo identical planar capacitor connected in parallel and charged to a voltage U0=12V. When you disconnect them from the voltage source, the distance between the plates of one of the capacitors is reduced to one third of the original distance. What will be the new voltage on the capacitors ?
View SolutionThe space between the rectangular plates (with sides aandb) of a parallel-plato capacitor (the distance between the plates is l) is filled with a solid dielectric whose dielectric constant is c. The capacitor is charged to a certain potential difference and disconnected from the voltage source. After that the dielectric is slowly moved out of the capacitor, which lTIOanS that the section x not filled with the dielectric gradually increases in size. How wi ll the potential difference between the plates and the surface charge densities on both parts of the capacitor (with and without the dielectric) change in the process?
View SolutionTwo conducting plates each having area A are kept at a separation d parallel to each other. The two plates are con- nected to a battery of emf V. The space between the plates is filled with a liquid of dielectric constant K. The height (x) of the liquid between the plates increases at a uniform rate from zero to d in a time interval t0.
(a) Write the capacitance of the system as a function of x as the liquid beings to fill the space between the plates.
(b) write the current through the cell at time t
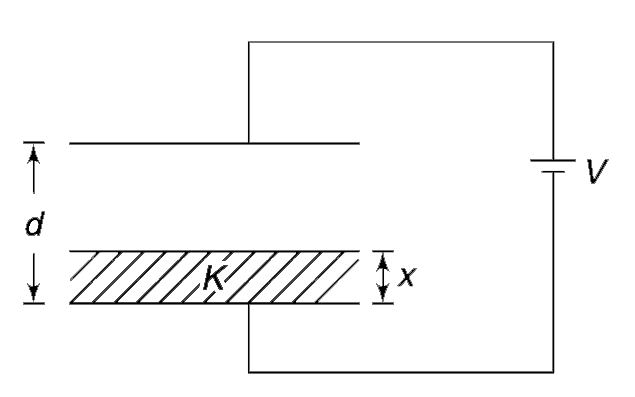
View SolutionThe dielectric filling the space between the plates of a capacitor that has been charged and then disconnected from the voltage source is removed. How should the distance between the plates be changed so that the energy stored in the capacitor remains the same? Explain the origin of the change in energy.
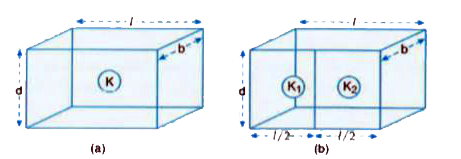
View SolutionTwo identical capacitors of plate dimensions l×b and plate separation 'd' have dielectric slabs filled in between the space of the plates as shown in Fig
Obtain the relation between the dielectric constants K,K1 and K2.
View Solution
