Question

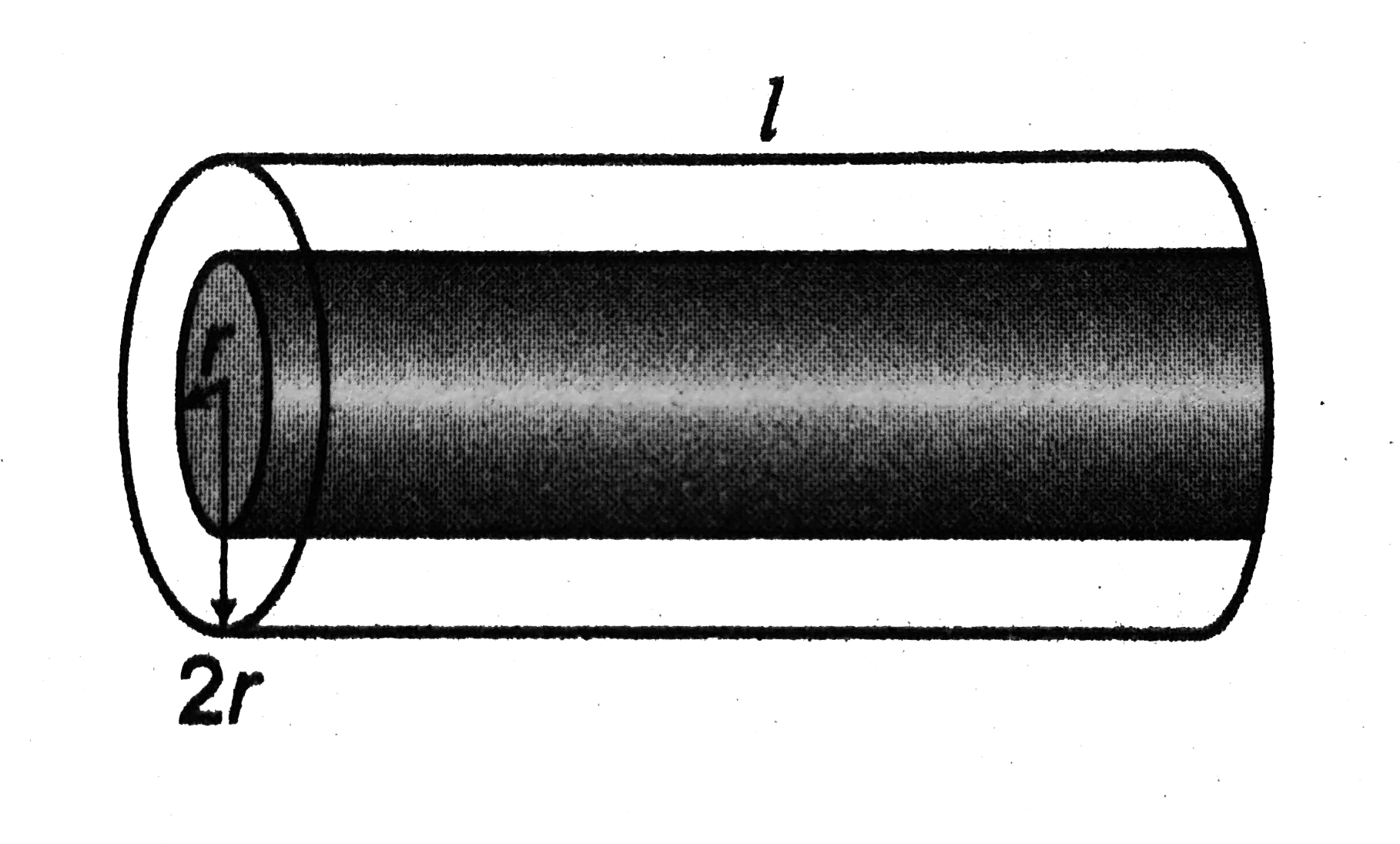
A cylinder of radius r made of a material of thermal conductivity $K_1$ is surrounded by a cylindrical shell of inner radius r and outer radius 2r made of a material of thermal conductivity $K_2$. The two ends of the combined system are maintained at two different temperatures. There is no loss of heat across the cylindrical surface and the system is in steady state. Show that the effective thermal conductivity of the system is $(K_1+3K_2)/4$.

Explanation

To solve the problem, we need to find the effective thermal conductivity of a system consisting of a solid cylinder surrounded by a cylindrical shell. Here’s the step-by-step solution:

Step 1: Understand the System
We have:
- A solid cylinder of radius \( r \) with thermal conductivity \( K_1 \).
- A cylindrical shell surrounding it with inner radius \( r \) and outer radius \( 2r \), made of a material with thermal conductivity \( K_2 \).
- The length of both the cylinder and the shell is \( L \).
- The system is in a steady state with no heat loss across the cylindrical surface.

Step 2: Calculate the Areas
- The cross-sectional area of the inner cylinder (A1) is:
\( A_1 = \pi r^2 \)
- The cross-sectional area of the outer shell (A2) is:
\( A_2 = \pi (2r)^2 - \pi r^2 = \pi (4r^2 - r^2) = 3\pi r^2 \)
- The total cross-sectional area (A) for the entire system is:
\( A = A_2 = 3\pi r^2 \)

Step 3: Calculate the Thermal Resistances
The thermal resistance \( R \) for each part of the system can be calculated using the formula:
\( R = \frac{L}{K \cdot A} \)

- For the inner cylinder (R1):
\( R_1 = \frac{L}{K_1 A_1} = \frac{L}{K_1 \pi r^2} \)

- For the outer shell (R2):
\( R_2 = \frac{L}{K_2 A_2} = \frac{L}{K_2 (3\pi r^2)} = \frac{L}{3K_2 \pi r^2} \)

Step 4: Combine the Resistances
Since the inner cylinder and the outer shell are in parallel, the total resistance \( R \) is given by:
\( \frac{1}{R} = \frac{1}{R_1} + \frac{1}{R_2} \)

Substituting the expressions for \( R_1 \) and \( R_2 \):
\( \frac{1}{R} = \frac{K_1 \pi r^2}{L} + \frac{3K_2 \pi r^2}{L} \)

Step 5: Simplify the Expression
Combining the terms gives:
\( \frac{1}{R} = \frac{(K_1 + 3K_2) \pi r^2}{L} \)

Thus, the total resistance \( R \) becomes:
\( R = \frac{L}{(K_1 + 3K_2) \pi r^2} \)

Step 6: Calculate Effective Thermal Conductivity
The effective thermal conductivity \( K \) can be defined as:
\( K = \frac{L}{R \cdot A} \)

Substituting \( R \) and \( A \):
\( K = \frac{L}{\left(\frac{L}{(K_1 + 3K_2) \pi r^2}\right) \cdot (3\pi r^2)} = \frac{(K_1 + 3K_2) \pi r^2}{3\pi r^2} = \frac{K_1 + 3K_2}{3} \)

Final Result
Thus, the effective thermal conductivity of the system is:
\( K_{\text{effective}} = \frac{K_1 + 3K_2}{4} \)