In variation of specific heat capcity of a substance with temperature is given by C=A+BT2, where A and B are constnts and T is the temperature, Calculate the mean specific heat in a temperature range T = 0 to T =T and the specific heat at the mid-point T/2.
The correct Answer is:A+(BT2/3)
Mean specific heat =(1/T)∫T0(A+BT2)=A+(BT2/3), At midpoint T=T/2
Similar Questions
Knowledge Check
If specific heat of a substance is infinite, it means a) heat is given out b) heat is taken in c) no change in temperature whether heat is taken in or given out d) all of these
Aheat is given outBheat is taken inCno change in temperature takes place whether heat is taken in a or given outDAll the aboveWhich one of the following graphs represents variation of specific heat capacity of water with temperature?
A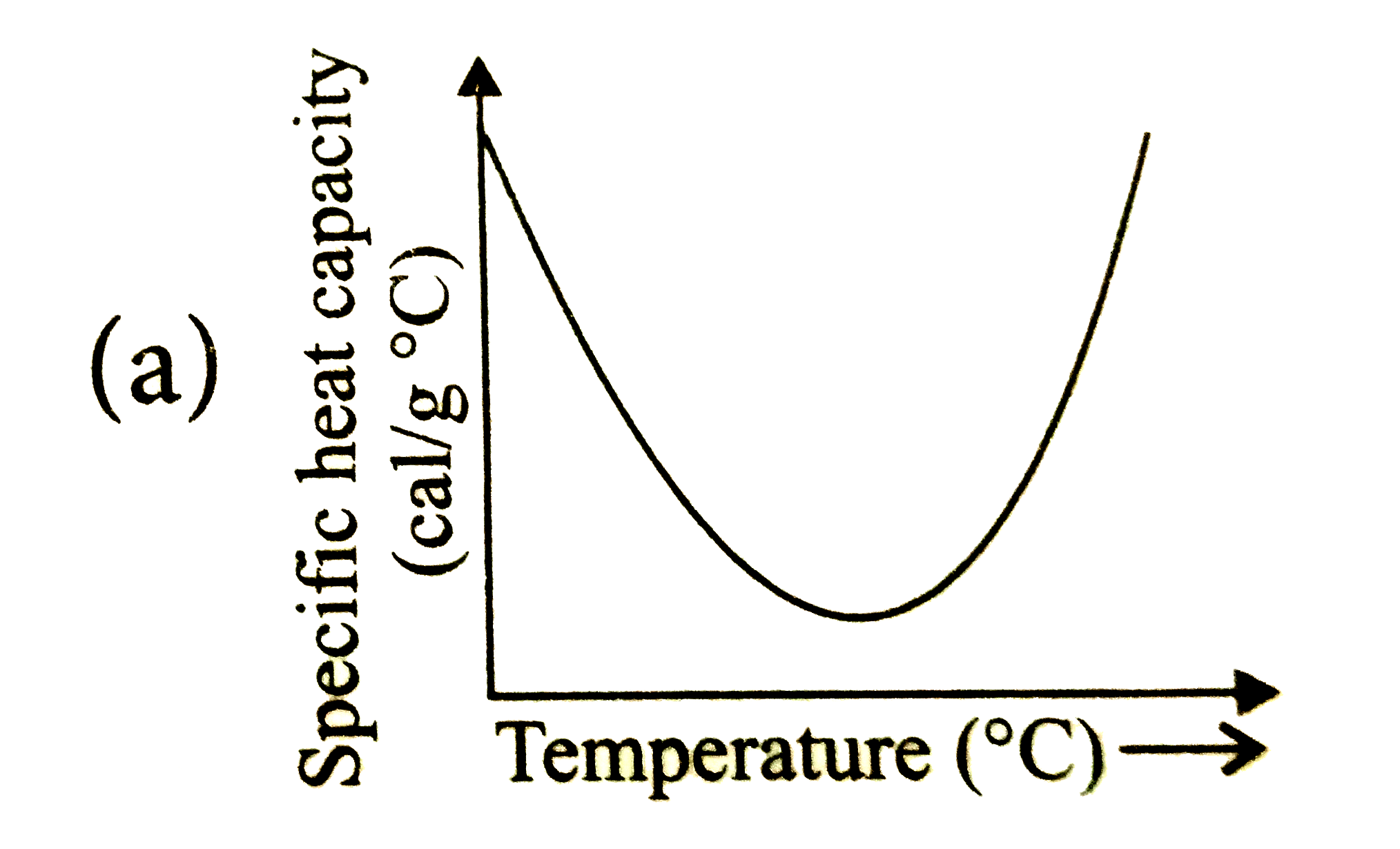 B
B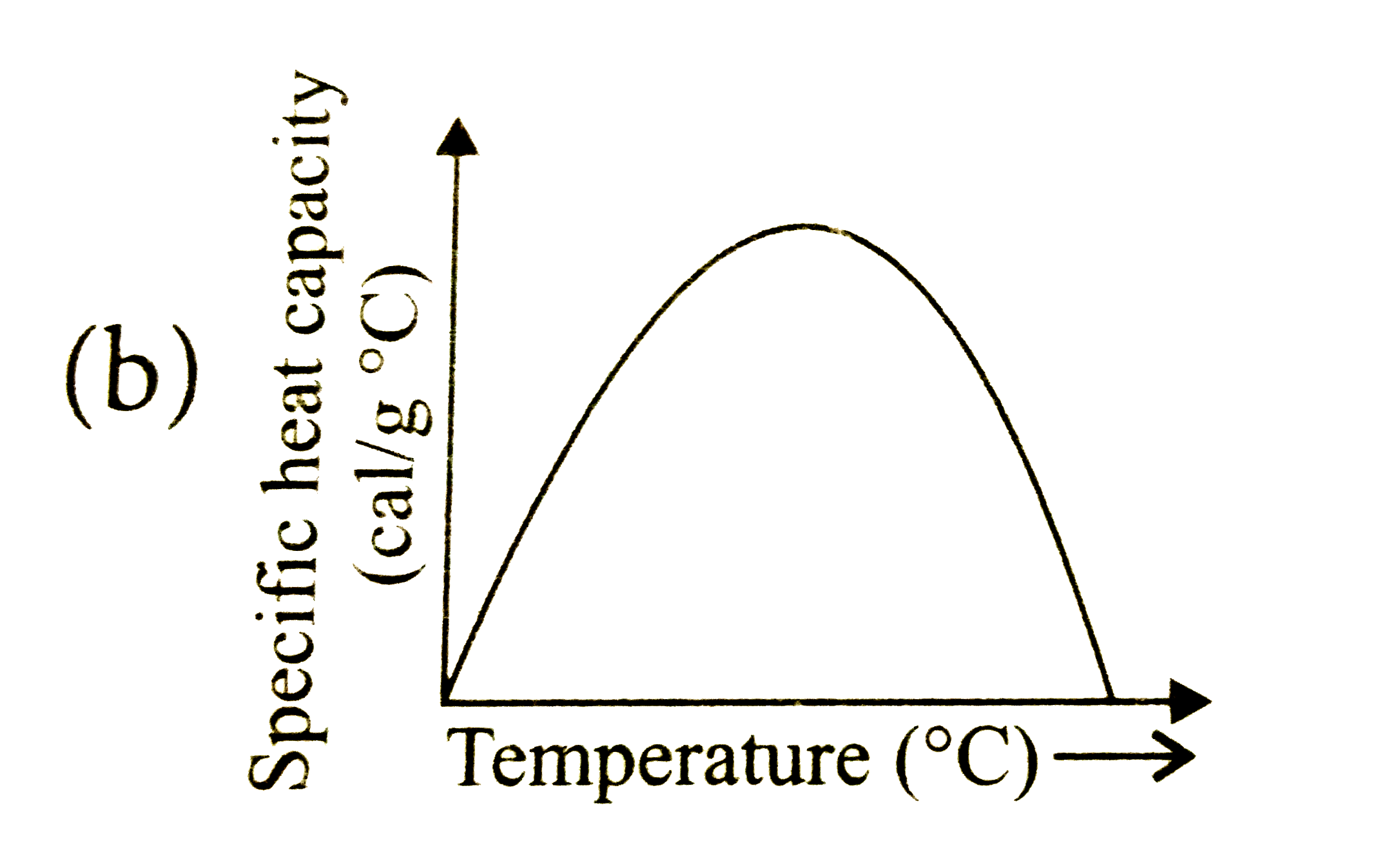 C
C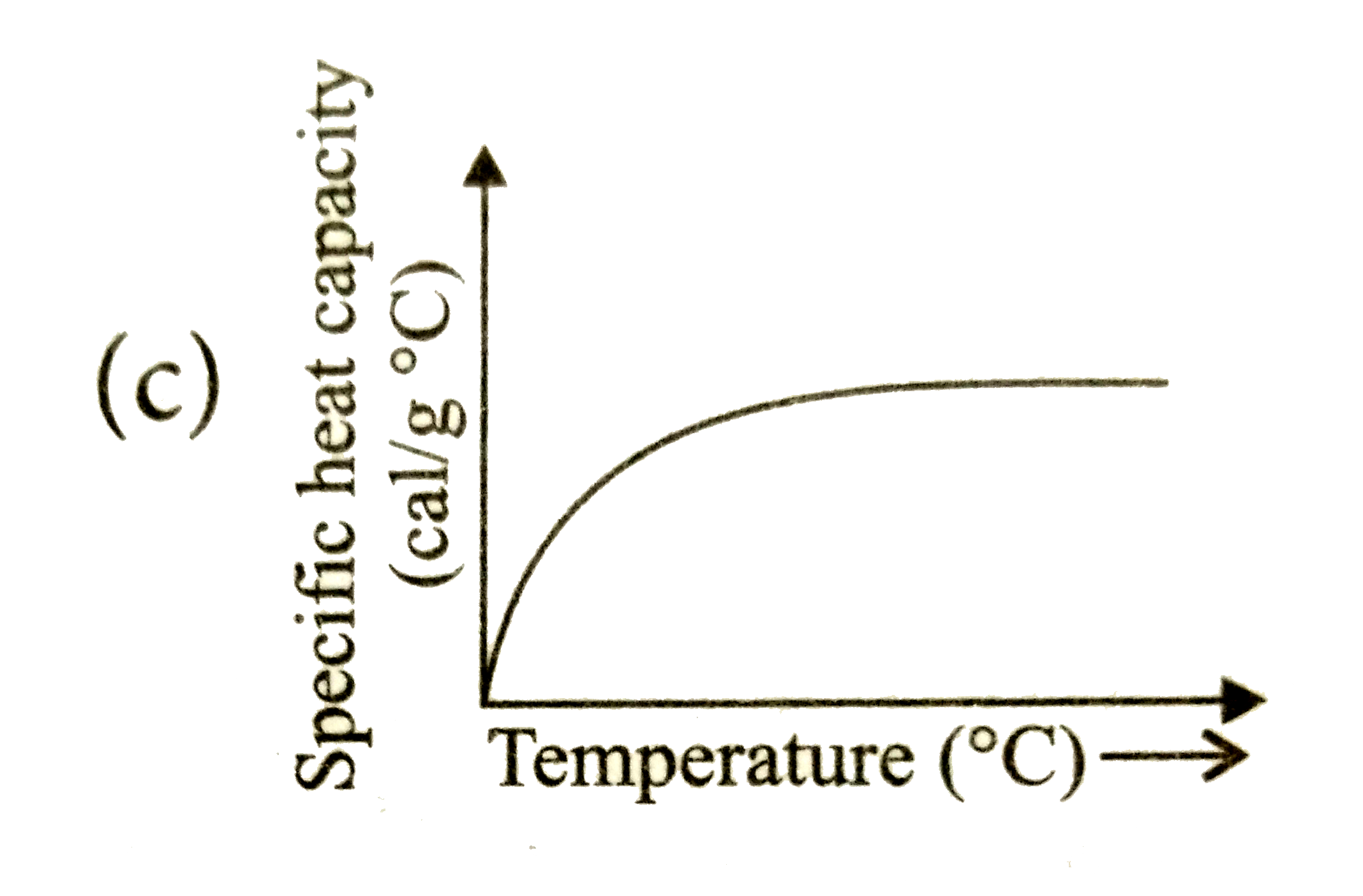 D
D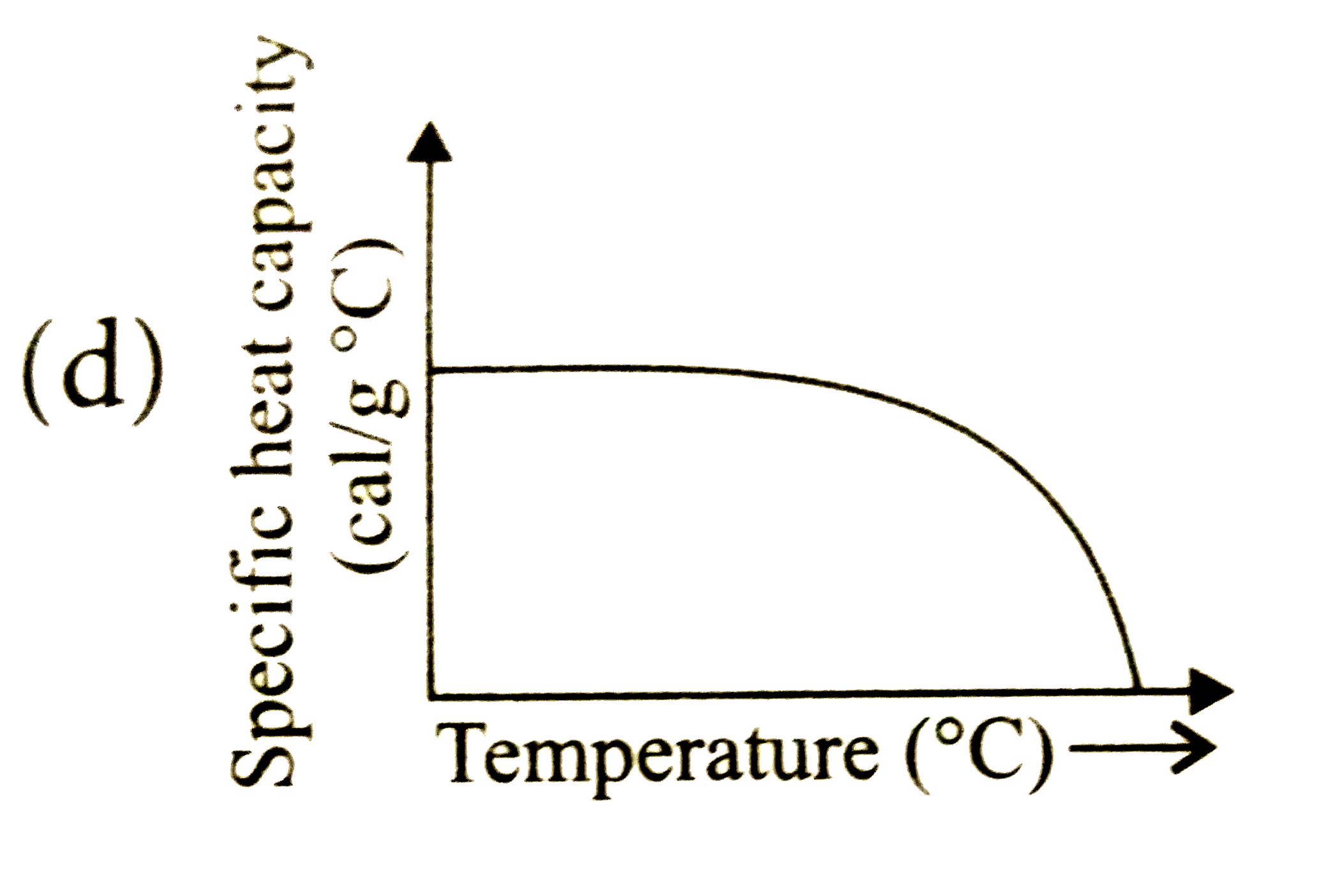
The specific heat of a metal at low temperatures varies according to S=aT3, where a is a constant and T is absolute temperature. The heat energy needed to raise unit mass of the metal from temperature T=1K to T=2K is
View SolutionAt a given temperature, the specific heat of a gas at constant pressure is always greater than its specific heat at constant volume.
View SolutionAssertion : Specific heat of any substance remains constant at all temperatures.
Reason : It is given by s=1m⋅dQdt .
View SolutionResistance of a resistor at temperature t∘C is Rt=R0(1+αt+βt2), where R0 is the resistance at 0∘C. The temperature coefficient of resistance at temperature t∘C is
View SolutionThe specific heat of a metal at low temperature varies according to S=(4/5)T3 where T is the absolute temperature. Find the heat energy needed to raise unit mass of the metal from T = 1 K →T= 2K.
View SolutionThe specific heat of many solids at low temperatures varies with absolute temperature T according to the relation S=AT3, where A is a constant. The heat energy required to raise the temperature of a mass m of such a solid from T = 0 to T = 20 K is
View Solution
