A,B, C and D purchased a cine-multiplex for ₹56 lakhs. The contribution of B,C and D together is 460% that of A,alone . The contribution of A,C and D together is 366.66% that of B's contribution and the contribution of C is 40% that of A,B and D together. The amount contributed by D is
10 lakh
12 lakh
16 lakh
18 lakh
The correct Answer is:D
To solve the problem step by step, we will define the contributions of A, B, C, and D in terms of units and then calculate the amount contributed by each person.
Step 1: Define the contributions
Let:
- A's contribution = A
- B's contribution = B
- C's contribution = C
- D's contribution = D
According to the problem, the total contribution is:
A+B+C+D=56 lakhs
Step 2: Set up the equations based on the given percentages
1. The contribution of B, C, and D together is 460% of A's contribution:
B+C+D=4.6A
2. The contribution of A, C, and D together is 366.66% of B's contribution:
A+C+D=3.6666B(which can be simplified to 113B)
3. The contribution of C is 40% of A, B, and D together:
C=0.4(A+B+D)
Step 3: Express B, C, and D in terms of A
From the first equation:
B+C+D=4.6A⟹C+D=4.6A−B
From the second equation:
A+C+D=113B⟹C+D=113B−A
Setting the two expressions for C+D equal:
4.6A−B=113B−A
Step 4: Solve for B in terms of A
Rearranging gives:
4.6A+A=113B+B
5.6A=143B
Multiplying through by 3:
16.8A=14B⟹B=16.814A=1.2A
Step 5: Substitute B back to find C and D
Now substituting B back into the equations:
Using B=1.2A in C+D=4.6A−B:
C+D=4.6A−1.2A=3.4A
Using C=0.4(A+B+D):
Substituting B:
C=0.4(A+1.2A+D)=0.4(2.2A+D)
Step 6: Solve for C and D
Now we have two equations:
1. C+D=3.4A
2. C=0.4(2.2A+D)
Substituting C from the second equation into the first:
0.4(2.2A+D)+D=3.4A
0.88A+0.4D+D=3.4A
0.88A+1.4D=3.4A
1.4D=3.4A−0.88A
1.4D=2.52A⟹D=2.521.4A=1.8A
Step 7: Substitute back to find C
Now substituting D back into C+D=3.4A:
C+1.8A=3.4A⟹C=3.4A−1.8A=1.6A
Step 8: Find the total contributions
Now we have:
- A = A
- B = 1.2A
- C = 1.6A
- D = 1.8A
Adding these contributions gives:
A+1.2A+1.6A+1.8A=56
5.6A=56⟹A=10 lakhs
Step 9: Calculate contributions
Now we can find the contributions:
- B=1.2×10=12 lakhs
- C=1.6×10=16 lakhs
- D=1.8×10=18 lakhs
Final Answer
The amount contributed by D is:
18 lakhs
Let:
- A's contribution = A
- B's contribution = B
- C's contribution = C
- D's contribution = D
A+B+C+D=56 lakhs
1. The contribution of B, C, and D together is 460% of A's contribution:
B+C+D=4.6A
A+C+D=3.6666B(which can be simplified to 113B)
C=0.4(A+B+D)
From the first equation:
B+C+D=4.6A⟹C+D=4.6A−B
A+C+D=113B⟹C+D=113B−A
4.6A−B=113B−A
Rearranging gives:
4.6A+A=113B+B
5.6A=143B
Multiplying through by 3:
16.8A=14B⟹B=16.814A=1.2A
Now substituting B back into the equations:
Using B=1.2A in C+D=4.6A−B:
C+D=4.6A−1.2A=3.4A
Substituting B:
C=0.4(A+1.2A+D)=0.4(2.2A+D)
Now we have two equations:
1. C+D=3.4A
2. C=0.4(2.2A+D)
0.4(2.2A+D)+D=3.4A
0.88A+0.4D+D=3.4A
0.88A+1.4D=3.4A
1.4D=3.4A−0.88A
1.4D=2.52A⟹D=2.521.4A=1.8A
Now substituting D back into C+D=3.4A:
C+1.8A=3.4A⟹C=3.4A−1.8A=1.6A
Now we have:
- A = A
- B = 1.2A
- C = 1.6A
- D = 1.8A
A+1.2A+1.6A+1.8A=56
5.6A=56⟹A=10 lakhs
Now we can find the contributions:
- B=1.2×10=12 lakhs
- C=1.6×10=16 lakhs
- D=1.8×10=18 lakhs
The amount contributed by D is:
18 lakhs
Topper's Solved these Questions
Similar Questions
For Uttarakhand flood victims two sections A and B of class X contributed Rs 1,500. If the contribution of X-A was Rs 100 less than that of X-B, find graphically the amounts contributed by both the sections.
View SolutionA, B, C, D and E start a partnership firm. Capital contributed by A is three times that contributed by D. E contributes half of A’s contribution, B contributes one-third of E’s contribution and C contributes two-third of A’s contribution. If the difference between the combined shares of A, D and E and the combined shares of B and C in the total profit of the firm is Rs. 13,500, what is the combined share of B, C and E ? (The shares are supposed to be proportional to the contributions)
View Solution
Knowledge Check
A, B, C and D purchased a cine-multiplex for Rs. 56 lakhs. The contribution of B, C and D together is 460% that of A, alone. The contribution of A, C and D together is 366.66% that of B's contribution and the contribution of C is 40% that of A, B and D together. The amount contibuted by D is :
A10 lakhB12 lakhC16 lakhD18 lakhThere are four persons A, B, C and D. The total amount of money with A and B together is equal to the total amount of money with C and D together. But the total amount of money with B and D together is more than the amount of money with A and C together. The amount of money with A is more than that with B. Who has the least amount of money ?
ABBCCDDCannot be determinedSelect the correct option for A, B , C and D given in the figure with respect to the relative contribution of various greenhouse gases to global warming.
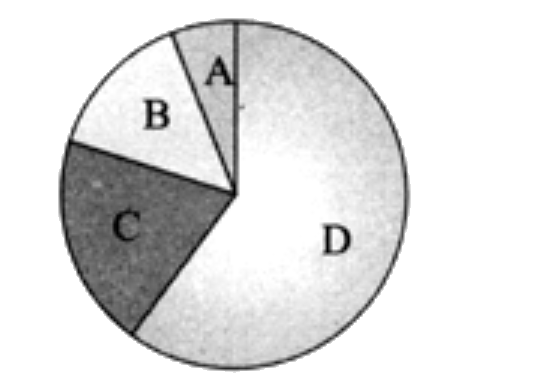 A(A−CO2),(B−CFCs),(C−CH4),(D−NO2)B(A−NO2),(B−CFCs),(C−CH4),(D−CO2)C(A−NO2),(B−CH4),(C−CFCs),(D−CO2)D(A−CH4),(B−CFCs),(C−NO2),(D−CO2)
A(A−CO2),(B−CFCs),(C−CH4),(D−NO2)B(A−NO2),(B−CFCs),(C−CH4),(D−CO2)C(A−NO2),(B−CH4),(C−CFCs),(D−CO2)D(A−CH4),(B−CFCs),(C−NO2),(D−CO2)
Four friends A, B, C, D, contribute money to a pool. A contributes double the amount C contributes and B contributes half the amount A contributes. D contributes half the amount of B. Which two are contributing equal amount?
View SolutionA is 40% less than B, and C is 40% of the sum of A and B. The difference between A and B is what percentage of C?
View SolutionA is 50% as efficient as B. C does half of the work done by A and B together. If C alone does the work in 20 days, then A, B and C together can do the work in
View SolutionIf the amount of A is 45% more than B, C is 60% less than A and B together and D is 20% less than C and the amount difference between B and D is Rs. 21,600 then find the amount of C.
View SolutionThe average marks of the students in four sections A, B, C and D together is 60%. The average marks of the students of A, B, C and D individually are 45%, 50%, 72% and 80% respectively. If the average marks of the students of sections A and B together is 48% and that of the students of B and C together is 60%. What is the ratio of number of students in section A and D?
View Solution
