In a ΔABC,D is a point on BC. AB is the hypotenus of then which relation is correct .
AB2+CD2=BC2+AD2
CD2+BD2=2AD2
AB2+AC2=2AD2
AB2+AD2=BD2
The correct Answer is:(i)
To solve the problem, we need to analyze the triangle ABC with point D on side BC, where AB is the hypotenuse. We will use the Pythagorean theorem to derive the relationships between the sides of the triangles involved.
1. Identify the Right Triangle:
- We have triangle ABC where AB is the hypotenuse.
- By definition, in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
2. Apply the Pythagorean Theorem to Triangle ABC:
- According to the Pythagorean theorem:
AB2=AC2+BC2(1)
3. Consider Triangle ACD:
- Triangle ACD is also a right triangle (assuming D is on BC).
- Applying the Pythagorean theorem here gives:
AD2=AC2+CD2(2)
4. Rearrange Equation (2):
- From equation (2), we can express AC2 in terms of AD2 and CD2:
AC2=AD2−CD2(3)
5. Substitute Equation (3) into Equation (1):
- Now, substitute AC2 from equation (3) into equation (1):
AB2=(AD2−CD2)+BC2
- Rearranging this gives:
AB2+CD2=AD2+BC2(4)
6. Conclusion:
- The correct relation derived from the above steps is:
AB2+CD2=BC2+AD2
- Thus, the correct option is option number 1.
- We have triangle ABC where AB is the hypotenuse.
- By definition, in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
- According to the Pythagorean theorem:
AB2=AC2+BC2(1)
- Triangle ACD is also a right triangle (assuming D is on BC).
- Applying the Pythagorean theorem here gives:
AD2=AC2+CD2(2)
- From equation (2), we can express AC2 in terms of AD2 and CD2:
AC2=AD2−CD2(3)
- Now, substitute AC2 from equation (3) into equation (1):
AB2=(AD2−CD2)+BC2
- Rearranging this gives:
AB2+CD2=AD2+BC2(4)
- The correct relation derived from the above steps is:
AB2+CD2=BC2+AD2
- Thus, the correct option is option number 1.
Topper's Solved these Questions
Similar Questions
Knowledge Check
In an equilateral triangle ABC, the side BC is trisected at D. Then the correct relation is
A9AD2=7AB2B8AD2=9AB2C7AD2=9AB2Dnone of theseIn an equilateral triangle ABC, the side BC is trisected at D. Then the correct relation is
A9AD2=7AB2B8AD2=9AB2C7AD2=9AB2DNone of theseIn a ΔABC right angle at C .P is the length of ⊥ from C to AB. If a, b, c, are the length of BC , CA, AB. Which relation is correct .
A1P2=1a2−1b2B1P2=1b2−1a2C1P2=1a2+1b2D1P2+1a2=1b2=0
In △ABC,AB>AC and D is any point on BC. Prove that, AB > AD.
View SolutionIn isosceles triangle ABC, D is a point on the base BC produced. Prove that AD>AB.

View SolutionIn a ΔABC,AB=AC=17cmD is a point on BC such that AD=15,CD=4cm, find BD=?
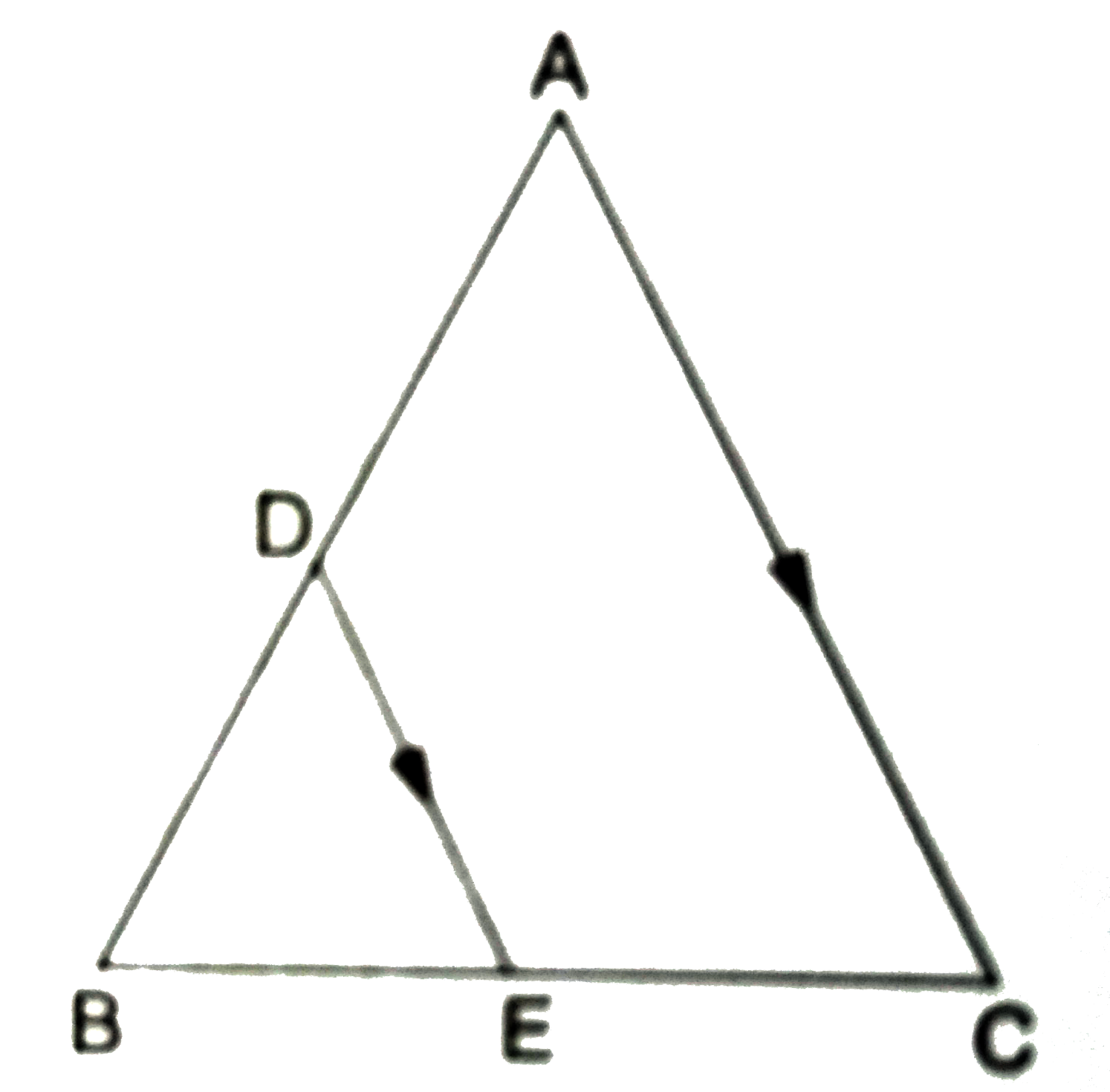
View SolutionIf D is a point on the side AB of ΔABC such that AD:BD=3:2andE is a point on BC such that DE∣∣AC, find the ratio of the areas of ΔABCandΔDBE
View SolutionIn ΔABC, AB=AC and D is a point on side AC such that BD = BC, If AB = 12.5 cm and BC = 5 cm, then what is the measure of DC ?
View Solution
