Define angular velocity and angular acceleration.
Step-by-Step Solution:
1. Definition of Angular Velocity:
- Angular velocity is defined as the rate of change of angular displacement with respect to time.
- Mathematically, it is represented as:
ω=dθdt
- Here, ω is the angular velocity, dθ is the change in angular displacement, and dt is the change in time.
- The SI unit of angular velocity is radians per second (rad/s).
2. Definition of Angular Acceleration:
- Angular acceleration is defined as the rate of change of angular velocity with respect to time.
- It can be expressed mathematically as:
α=dωdt
- In this equation, α is the angular acceleration, dω is the change in angular velocity, and dt is the change in time.
- The SI unit of angular acceleration is radians per second squared (rad/s²).
Summary:
- Angular Velocity (ω): Rate of change of angular displacement with respect to time; units: rad/s.
- Angular Acceleration (α): Rate of change of angular velocity with respect to time; units: rad/s².
- Angular velocity is defined as the rate of change of angular displacement with respect to time.
- Mathematically, it is represented as:
ω=dθdt
- Here, ω is the angular velocity, dθ is the change in angular displacement, and dt is the change in time.
- The SI unit of angular velocity is radians per second (rad/s).
- Angular acceleration is defined as the rate of change of angular velocity with respect to time.
- It can be expressed mathematically as:
α=dωdt
- In this equation, α is the angular acceleration, dω is the change in angular velocity, and dt is the change in time.
- The SI unit of angular acceleration is radians per second squared (rad/s²).
- Angular Velocity (ω): Rate of change of angular displacement with respect to time; units: rad/s.
- Angular Acceleration (α): Rate of change of angular velocity with respect to time; units: rad/s².
Topper's Solved these Questions
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (SHORT ANSWER QUESTIONS)
35 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (VERY SHORT ANSWER QUESTIONS)
14 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (CONCEPTUAL SHORT ANSWERS QUESTIONS WITH ANSWERS)
14 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS
50 Videos
Similar Questions
The angular displacement of a body is given by θ=2t2+5t−3. Find the value of the angular velocity and angular acceleration when t = 2s.
View SolutionA particle starts rotating from rest according to the formula θ=(t2/64)−(t/8) where θ is the angle in radian and t in s . Find the angular velocity and angular acceleration at the end of 4 s .
View SolutionThe linear speed of a particle moving in a circle of radius R varies with time as v=v0−kt, where k is a positive constant. At what time the magnitudes of angular velocity and angular acceleration will be equal ?
View SolutionThe angular velocity and angular acceleration of the pivoted rod are given as ω and α respectively. Fid the x and y components of acceleration of B.
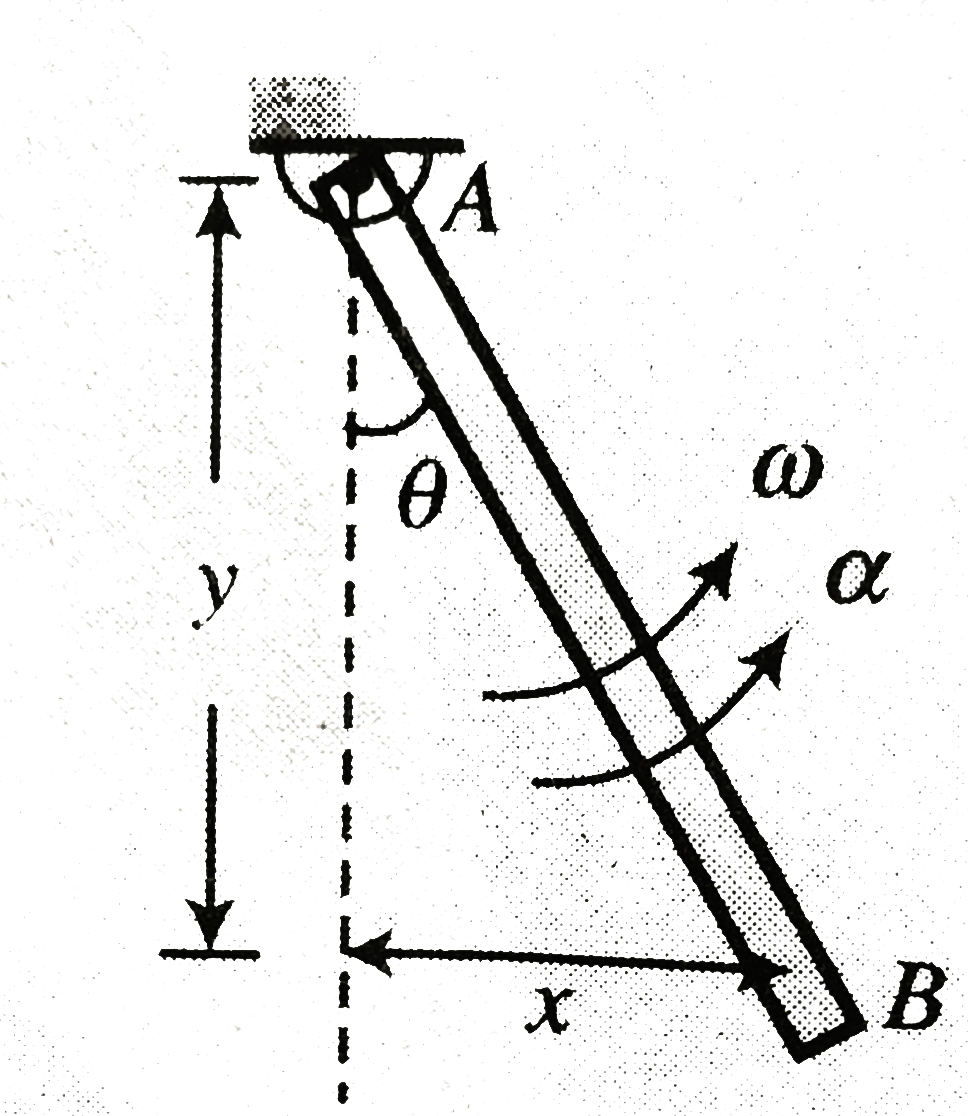
View SolutionA uniiform disc of radius r spins with angular velocity ω and angular acceleration α. If the centre of mass of the disc has linear acceleration a, find the magnitude and direction of aceeleration of the point 1,2, and 3.
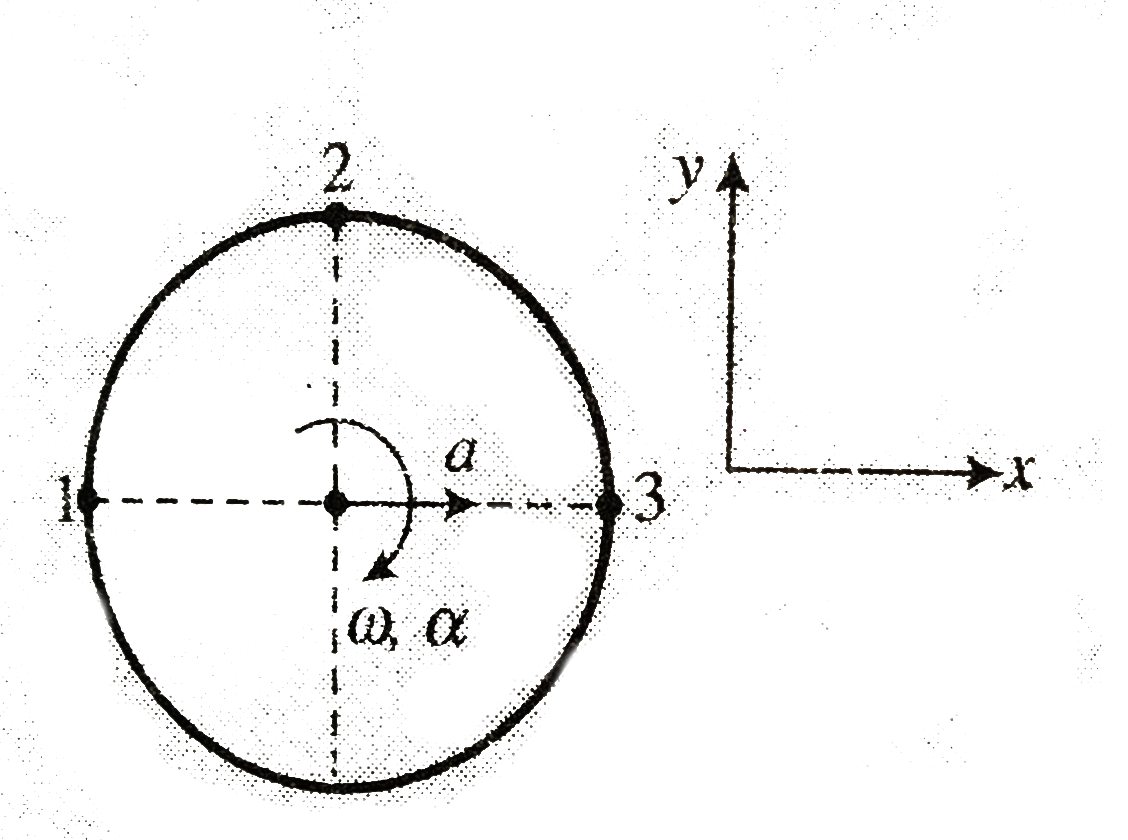
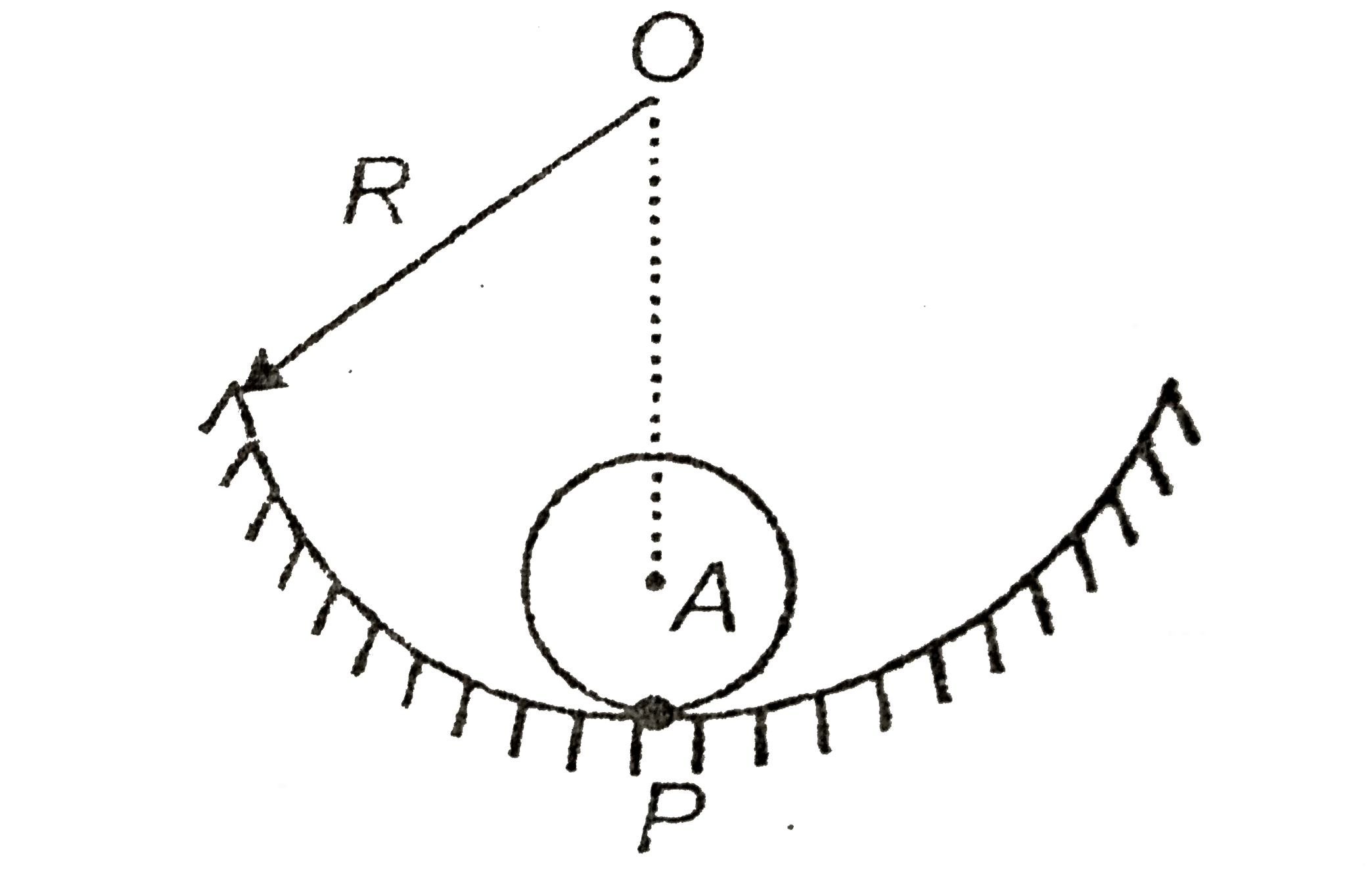
View SolutionA sphere of radius r is rolling without slipping on a hemispherical surface of radius R. Angular velocity and angular acceleration of line OA is ω and α respectively. Find the acceleration of point P on sphere.
View SolutionA particle is moving on a circular path such that at any instant its position vector, linear velocity. Angular velocity, angular acceleration with respect to centre are →r,→v,→w,→α respectively. Net acceleration of the particle is :−
View SolutionA particle is moving along a circular path. The angular velocity, linear velocity, Angular acceleration, and centripetal acceleration of the particle at any instant. Respectively are →ω,→v,→∝,and→ac. Which of the following relations is not correct ?
View SolutionA disc of radius R rolls on a horizontal ground with linear acceleration a and angular acceleration α as shown in figure. The magnitude of acceleration of point P at an instant when its linear velocity is v and angular velocity is ω will be:

View SolutionA particle attached to a string is rotating with a constant angular velocity and its angular momentum is L. If the string is halved and angular velocity is kept constant, the angular momentum will be
View Solution
