A flywheel of mass 400 kg and one metre radius makes 600 rev/minute. Assuming its mass to be concentrated at the rim, calculate the angular velocity, M.I. and energy of the flywheel.
To solve the problem, we will follow these steps:
Step 1: Calculate the Angular Velocity (ω)
The angular velocity in radians per second can be calculated using the formula:
ω=2π×(revolutions per minute)÷60
Given that the flywheel makes 600 revolutions per minute:
ω=2π×600÷60
Calculating this gives:
ω=2π×10=20π rad/s
Now, calculating the numerical value:
ω≈62.83 rad/s
Step 2: Calculate the Moment of Inertia (I)
The moment of inertia for a flywheel with mass concentrated at the rim is given by:
I=M×R2
Where:
- M=400kg (mass of the flywheel)
- R=1m (radius of the flywheel)
Substituting the values:
I=400×(12)=400kg m2
Step 3: Calculate the Energy (E)
The kinetic energy of rotation for the flywheel can be calculated using the formula:
E=12Iω2
Substituting the values we have calculated:
E=12×400×(62.83)2
Calculating (62.83)2:
(62.83)2≈3945.84
Now substituting this back into the energy equation:
E=12×400×3945.84≈789168J
Converting this to kilojoules:
E≈789.17kJ
Final Answers
- Angular Velocity, ω≈62.83rad/s
- Moment of Inertia, I=400kg m2
- Energy, E≈789.17kJ
The angular velocity in radians per second can be calculated using the formula:
ω=2π×(revolutions per minute)÷60
Given that the flywheel makes 600 revolutions per minute:
ω=2π×600÷60
Calculating this gives:
ω=2π×10=20π rad/s
Now, calculating the numerical value:
ω≈62.83 rad/s
The moment of inertia for a flywheel with mass concentrated at the rim is given by:
I=M×R2
Where:
- M=400kg (mass of the flywheel)
- R=1m (radius of the flywheel)
I=400×(12)=400kg m2
The kinetic energy of rotation for the flywheel can be calculated using the formula:
E=12Iω2
Substituting the values we have calculated:
E=12×400×(62.83)2
Calculating (62.83)2:
(62.83)2≈3945.84
Now substituting this back into the energy equation:
E=12×400×3945.84≈789168J
Converting this to kilojoules:
E≈789.17kJ
- Angular Velocity, ω≈62.83rad/s
- Moment of Inertia, I=400kg m2
- Energy, E≈789.17kJ
Topper's Solved these Questions
Similar Questions
A ring of mass m and radius r rotates about an axis passing through its centre and perpendicular to its plane with angular velocity ω. Its kinetic energy is
View Solution"The mass of a flywheel is concentrated on the rim ". Why ?
View Solutionthe flywheel is so constructed that the entire mass of it is concentrated at its rim, because
View SolutionA car of mass 300 kg is travelling on a circular track of radius 100 m with a constant speed of 60 m/s
Calculate the angular momentum ?
View SolutionA car of mass 300 kg is travelling on a circular track of radius 100 m with a constant speed of 60 m/s
Calculate the angular momentum ?
View SolutionA flywheel of radiiis 10 cm mounted so as to rotate about a horizontal axis through its centre. A string of negligible mass wrapped round its' circumference carries' a mass of 200 gm attached to its free end, when let fall the mass descends through 100 cm is 5 s. Calculate the angular acceleration and the moment of inertia of the flywheel.
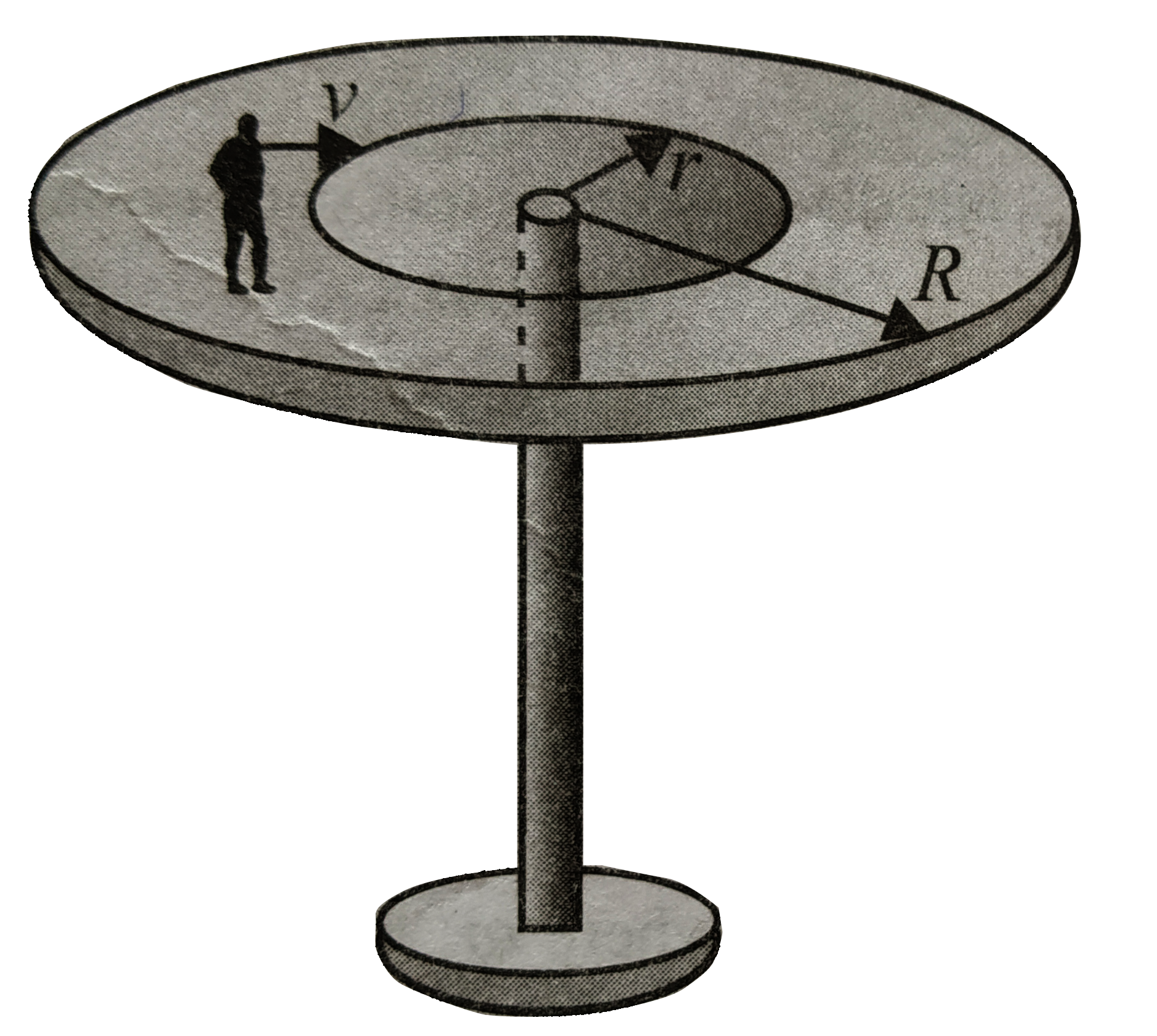
View SolutionA man of mass m stands on a horizontal platform in the shape of a disc of mass m and radius R, pivoted on a vertical axis thorugh its centre about which it can freely rotate. The man starts to move aroung the centre of the disc in a circle of radius r with a velocity v relative to the disc. Calculate the angular velocity of the disc.
View SolutionA flywheel of mass 0.2 kg and radius 10 cm is rotating with 5πrevs about an axis perpendicular to its plane passing through its centre. Calculate angular momentum and kinetic energy of flywheel.
View SolutionA solid sphere of radius r and mass m rotates about an axis passing through its centre with angular velocity ω. Its K.E. Is
View SolutionA flywheel of mass 100 kg and radius 1 m is rotating at the rate of 420 rev/min. Find the constant retarding torque to stop the wheel in 14 revolutions, the mass is concentrated at the rim. M.I. of the flywheel about its axis of rotation I=mr2.
View Solution
