A body floats in a liquid A of density ρ1 with a part of it submerged inside liquid while in liquid B of density ρ2 totally submerged inside liquid. The densities ρ1 and ρ2 are related as :
ρ1=ρ2
ρ1<ρ2
ρ1>ρ2
nothing can be said
The correct Answer is:C
To solve the problem, we need to analyze the conditions under which a body floats in two different liquids, A and B, with given densities ρ1 and ρ2.
1. Understanding the Scenario:
- In liquid A (density ρ1), the body is partially submerged, which means it floats.
- In liquid B (density ρ2), the body is completely submerged.
2. Applying Archimedes' Principle:
- According to Archimedes' principle, a body will float if the weight of the liquid displaced by the submerged part of the body is equal to the weight of the body itself.
- For the body to float in liquid A, the density of the body must be less than the density of liquid A. Therefore, we can express this as:
Density of body<ρ1
3. Analyzing Liquid B:
- In liquid B, since the body is completely submerged, it means that the buoyant force acting on the body is equal to the weight of the body. However, for the body to be fully submerged, the density of the body must be greater than the density of liquid B. Thus, we can express this as:
Density of body>ρ2
4. Combining the Inequalities:
- From the two inequalities derived from the conditions in liquids A and B, we have:
ρ2<Density of body<ρ1
- This implies that the density of the body lies between the densities of the two liquids.
5. Establishing the Relationship:
- Since the density of the body is greater than ρ2 and less than ρ1, we can conclude that:
ρ1>ρ2
Final Conclusion:
The relationship between the densities of the two liquids is:
ρ1>ρ2
- In liquid A (density ρ1), the body is partially submerged, which means it floats.
- In liquid B (density ρ2), the body is completely submerged.
- According to Archimedes' principle, a body will float if the weight of the liquid displaced by the submerged part of the body is equal to the weight of the body itself.
- For the body to float in liquid A, the density of the body must be less than the density of liquid A. Therefore, we can express this as:
Density of body<ρ1
- In liquid B, since the body is completely submerged, it means that the buoyant force acting on the body is equal to the weight of the body. However, for the body to be fully submerged, the density of the body must be greater than the density of liquid B. Thus, we can express this as:
Density of body>ρ2
- From the two inequalities derived from the conditions in liquids A and B, we have:
ρ2<Density of body<ρ1
- This implies that the density of the body lies between the densities of the two liquids.
- Since the density of the body is greater than ρ2 and less than ρ1, we can conclude that:
ρ1>ρ2
The relationship between the densities of the two liquids is:
ρ1>ρ2
Topper's Solved these Questions
- UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONBOOK - ICSECHAPTER - UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONEXERCISE - EXERCISE 5(c) (Numerical)
9 Videos
- UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONBOOK - ICSECHAPTER - UPTHRUST IN FLUIDS, ARCHIMEDES' PRINCIPLE AND FLOATATIONEXERCISE - EXERCISE 5(c)
26 Videos
- SOUNDBOOK - ICSECHAPTER - SOUNDEXERCISE - TOPIC 2 Infrasonic and Ultrasonic Waves ( 3 Marks Questions)
3 Videos
Similar Questions
Knowledge Check
The pressure inside a liquid of density ρ at a depth h is :
AhρgBhρgChρgDhρ
A body weighs W1 in a liquid of density ρ1 and W2 in a liquid of density ρ2. What is the weight of body in a liquid of density ρ3?
View SolutionA ball of density ρ is released from deep inside of a liquid of density 2ρ. It will move up
View SolutionA body of density ρ floats with volume V1 of its total volume V immersed in a liquid of density ρ1 and with the remainder of volume V2 immersed in another liquid of density ρ2 where ρ1>ρ2. Find the volume immersed in two liquids. (V1 and V2).
View Solution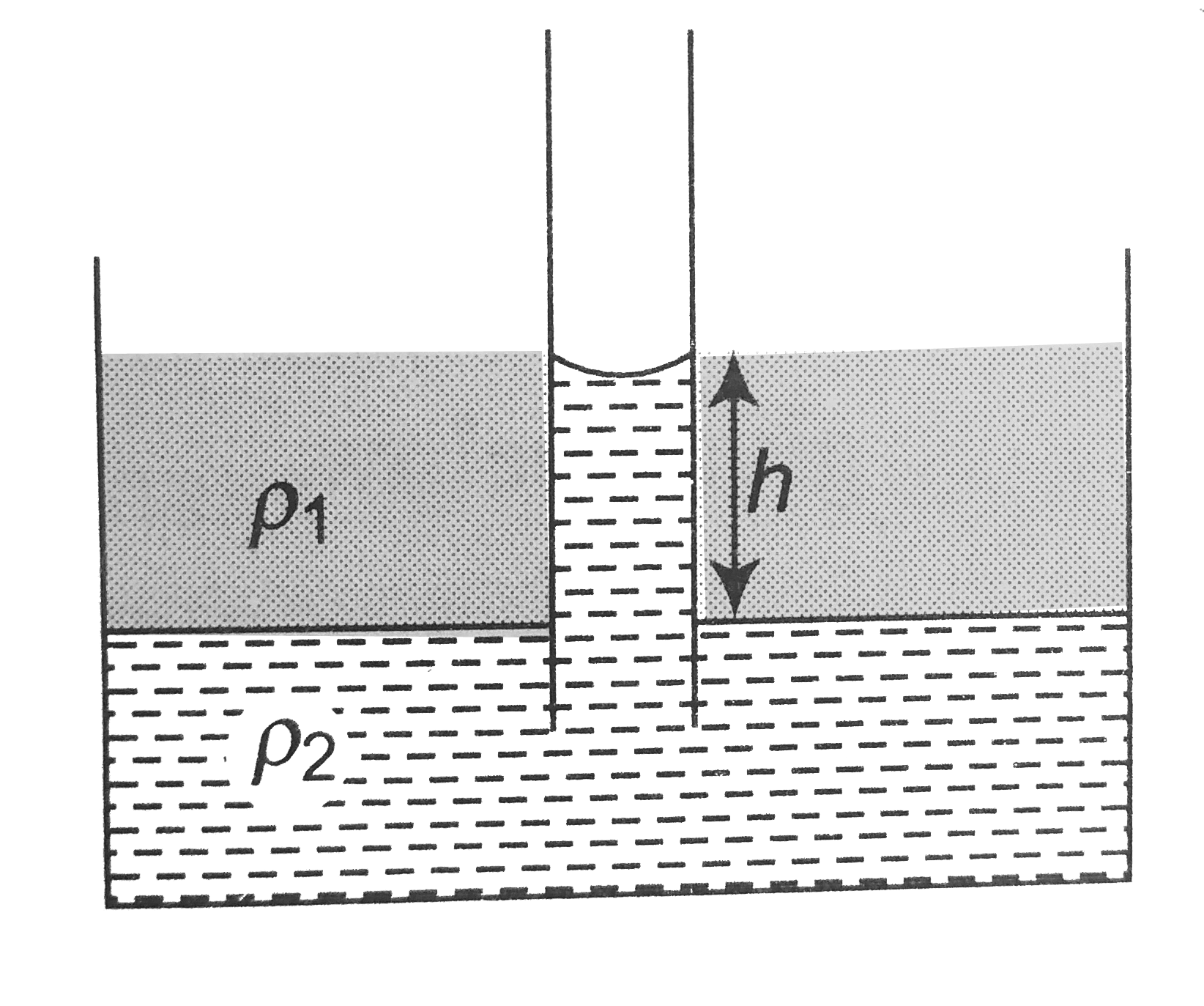
A container is partially filled with a liquid of density ρ2 A capillary tube of radius r is vertically inserted in this liquid. Now another liquid of density ρ1(ρ1<ρ2) is slowly poured in the container to a height h as shown. There is only denser liquid in the capillary tube. The rise of denser liquid in the capillary tube is also h. Assuming zero contact angle, the surface tension of heavier liquid is
View SolutionA block of density ρ floats in a liquid with its one third volume immersed. The density of the liquid is
View SolutionA block of material has a density ρ1 and floats three-fourth submerged in a liquid of unknown density. Show tht the density ρ2 of the unknown liquid is given by ρ2=43ρ1.
View SolutionA ball of volume V and density ρ1 is moved downwards by a distance 'd' in liquid of density ρ2. Find total change in potential energy of the system.

View Solution
