Question

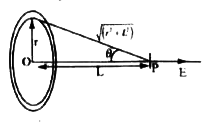
The largest magnitude the electric field on the axis of a uniformly charged ring of radius 3m is at a distance h from its centre. What is the value of h? (Take $\frac{1}{\sqrt{2}}=0.7$)

Answer

2.1

Explanation

To find the distance $$h$$ from the center of a uniformly charged ring where the electric field is maximized, we can follow these steps:

Step 1: Understand the Electric Field on the Axis of a Ring
The electric field \( E \) at a distance \( h \) from the center of a uniformly charged ring of radius \( r \) is given by the formula:
\( E = \frac{kQh}{(h^2 + r^2)^{3/2}} \)
where:
- \( k \) is the Coulomb's constant,
- \( Q \) is the total charge on the ring,
- \( r \) is the radius of the ring,
- \( h \) is the distance from the center along the axis.

Step 2: Differentiate the Electric Field with Respect to \( h \)
To find the maximum electric field, we need to differentiate \( E \) with respect to \( h \) and set the derivative equal to zero:
\( \frac{dE}{dh} = 0 \)

Step 3: Apply the Condition for Maximum Electric Field
From the differentiation, we find that the condition for maximum electric field occurs when:
\( h = \frac{r}{\sqrt{2}} \)

Step 4: Substitute the Given Radius
In this problem, the radius \( r \) is given as 3 meters. Substituting this value into the equation:
\( h = \frac{3}{\sqrt{2}} \)

Step 5: Simplify the Expression
Using the approximation \( \frac{1}{\sqrt{2}} \approx 0.7 \):
\( h \approx 3 \times 0.7 = 2.1 \text{ meters} \)

Final Answer
Thus, the value of \( h \) is:
\( \boxed{2.1 \text{ meters}} \)

---