PQRS is a parallelogram with Find PS.
The correct Answer is:
To find the length of PS in the parallelogram PQRS, we can use the properties of the area of a parallelogram. Here’s a step-by-step solution:
Step 1: Understand the given information
We know:
- PQ = 15 cm (one side of the parallelogram)
- ST = 10 cm (the height corresponding to base PQ)
- QV = 12 cm (the height corresponding to base PS)
Step 2: Calculate the area using base PQ and height ST
The area of a parallelogram can be calculated using the formula:
Area=Base×Height
Using PQ as the base:
Area=PQ×ST=15cm×10cm=150cm2
Step 3: Set up the area equation using base PS and height QV
Now, we can also express the area using PS as the base and QV as the height:
Area=PS×QV
Substituting the value of QV:
Area=PS×12cm
Step 4: Equate the two area expressions
Since both expressions represent the area of the same parallelogram, we can set them equal to each other:
150cm2=PS×12cm
Step 5: Solve for PS
To find PS, we rearrange the equation:
PS=150cm212cm
Calculating this gives:
PS=12.5cm
Final Answer
Thus, the length of PS is 12.5cm.
---
We know:
- PQ = 15 cm (one side of the parallelogram)
- ST = 10 cm (the height corresponding to base PQ)
- QV = 12 cm (the height corresponding to base PS)
The area of a parallelogram can be calculated using the formula:
Area=Base×Height
Area=PQ×ST=15cm×10cm=150cm2
Now, we can also express the area using PS as the base and QV as the height:
Area=PS×QV
Area=PS×12cm
Since both expressions represent the area of the same parallelogram, we can set them equal to each other:
150cm2=PS×12cm
To find PS, we rearrange the equation:
PS=150cm212cm
PS=12.5cm
Thus, the length of PS is 12.5cm.
Topper's Solved these Questions
- PERIMETER AND AREA BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - UNIT PRACTICE PAPER V
10 Videos
- PERIMETER AND AREA BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - TRY THIS
41 Videos
- PERIMETER AND AREA BOOK - ICSECHAPTER - PERIMETER AND AREA EXERCISE - EXERCISE 17.3
16 Videos
- PERCENTAGE, PROFIT AND LOSSBOOK - ICSECHAPTER - PERCENTAGE, PROFIT AND LOSSEXERCISE - REVISION EXERCISE
26 Videos
- PROBABILITYBOOK - ICSECHAPTER - PROBABILITYEXERCISE - TRY THIS
14 Videos
Similar Questions
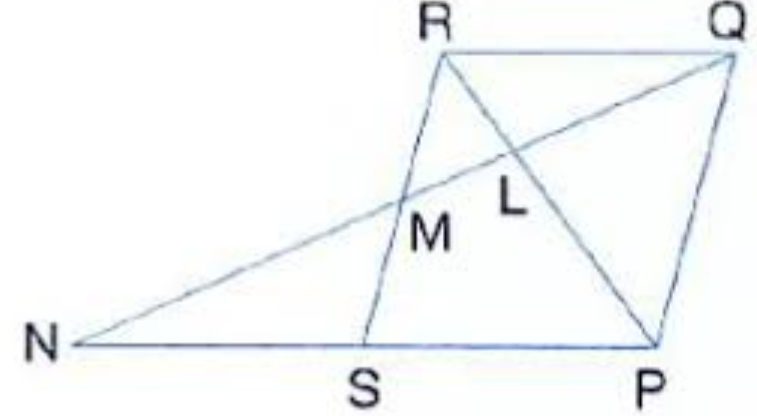
In the figure, PQRS is a parallelogram with PQ = 16 cm and QR = 10 cm. L is a point on PR such that RL:LP=2:3. QL produced meets RS at M and PS produced at N.
Find the lengths of PN and RM.
View SolutionA circle is inscribed in a triangle PQR with PQ = 10 cm, QR = 8 cm and PR = 12 cm. Find the lengths QM, RN and PL.
View SolutionA circle is inscribed in a triangle PQR with PQ = 10 cm, QR = 8 cm and PR = 12 cm. Find the lengths QM, RN and PL.
View SolutionPQRS is a parallegoram whose adjecent sides PQ = 20 cm and QR = 21 cm one of its diagonals QS = 13 cm , find :
the area of the parallelogram PQRS.
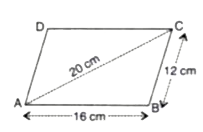
View SolutionIn parallelogram ABCD, AB = 16 cm, BC = 12 cm and diagonal AC = 20 cm. Find the area of the parallelogram.
View SolutionPQRS is a parallegoram whose adjecent sides PQ = 20 cm and QR = 21 cm one of its diagonals PR = 13 cm , find :
the distance between the shorter sides.
View SolutionPQRS is a parallegoram whose adjecent sides PQ = 20 cm and QR = 21 cm one of its diagonals PR = 13 cm , find :
the distance between the longer sides.
View SolutionThe area of a parallelogram PQRS is 162cm2 This parallelogram has adjacent sides PQ = 27 cm and QR = 12 cm . Find the distance between its longer sides and that between its shorter sides .
View SolutionFind the area of a parallelogram ABCD in which AB = 8 cm, BC = 15 cm and diagonal AC = 17 cm.
View SolutionPQRS is a parallelogram with ST perpendicular to PQ. Given area of Δ PST = 54 sq.cm, QS = 13 cm, and TQ = 5 cm, find the perimeter of the parallelogram.
View Solution
