In the following figure, CE is drawn parallel to diagonal DB of the quadrilateral ABCD which meets AB produced ar point E.
Prove that ΔADE and quadrilateral ABCD are equal in area.
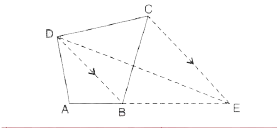
Step by step video & image solution for In the following figure, CE is drawn parallel to diagonal DB of the quadrilateral ABCD which meets AB produced ar point E. Prove that DeltaADE and quadrilateral ABCD are equal in area. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
- AREA THEOREMSBOOK - ICSECHAPTER - AREA THEOREMSEXERCISE - Exercies 16(B)
16 Videos
- AREA THEOREMSBOOK - ICSECHAPTER - AREA THEOREMSEXERCISE - Exercies 16(C )
22 Videos
- AREA THEOREMSBOOK - ICSECHAPTER - AREA THEOREMSEXERCISE - Exercies 16(C )
22 Videos
- AREA AND PERIMETER OF PLANE FIGURESBOOK - ICSECHAPTER - AREA AND PERIMETER OF PLANE FIGURESEXERCISE - EXERCISE 20(D)
12 Videos
- CHAPTER REVISION (STAGE 2) BOOK - ICSECHAPTER - CHAPTER REVISION (STAGE 2) EXERCISE - DISTANCE FORMULA
12 Videos
Similar Questions
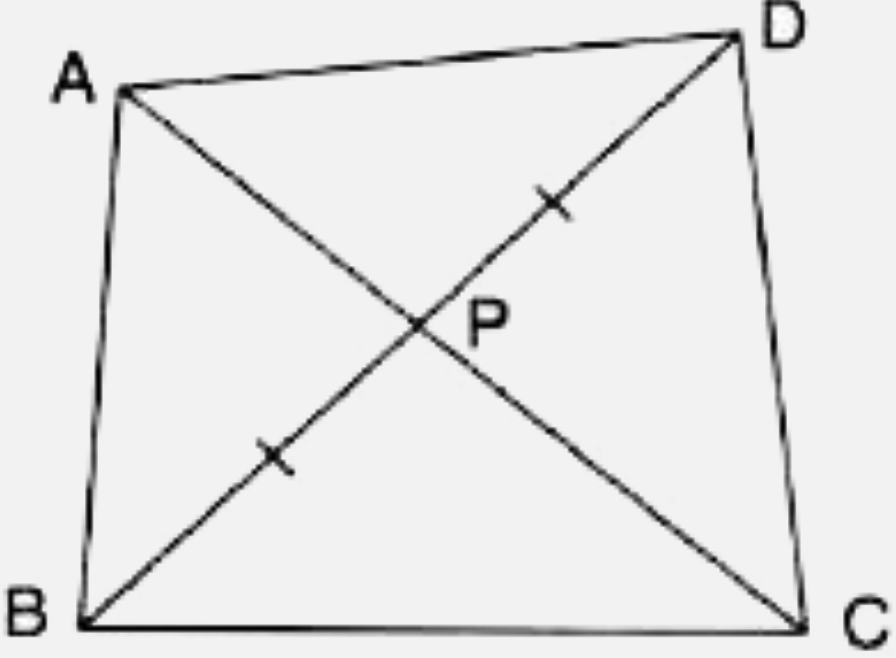
In quadrilateral ABCD, diagonal BD is bisected by the diagonal AC. Prove that : ΔABCandΔADC are equal in area.
View SolutionFind the perimeter and area of the quadrilateral ABCD in which AB=21cm
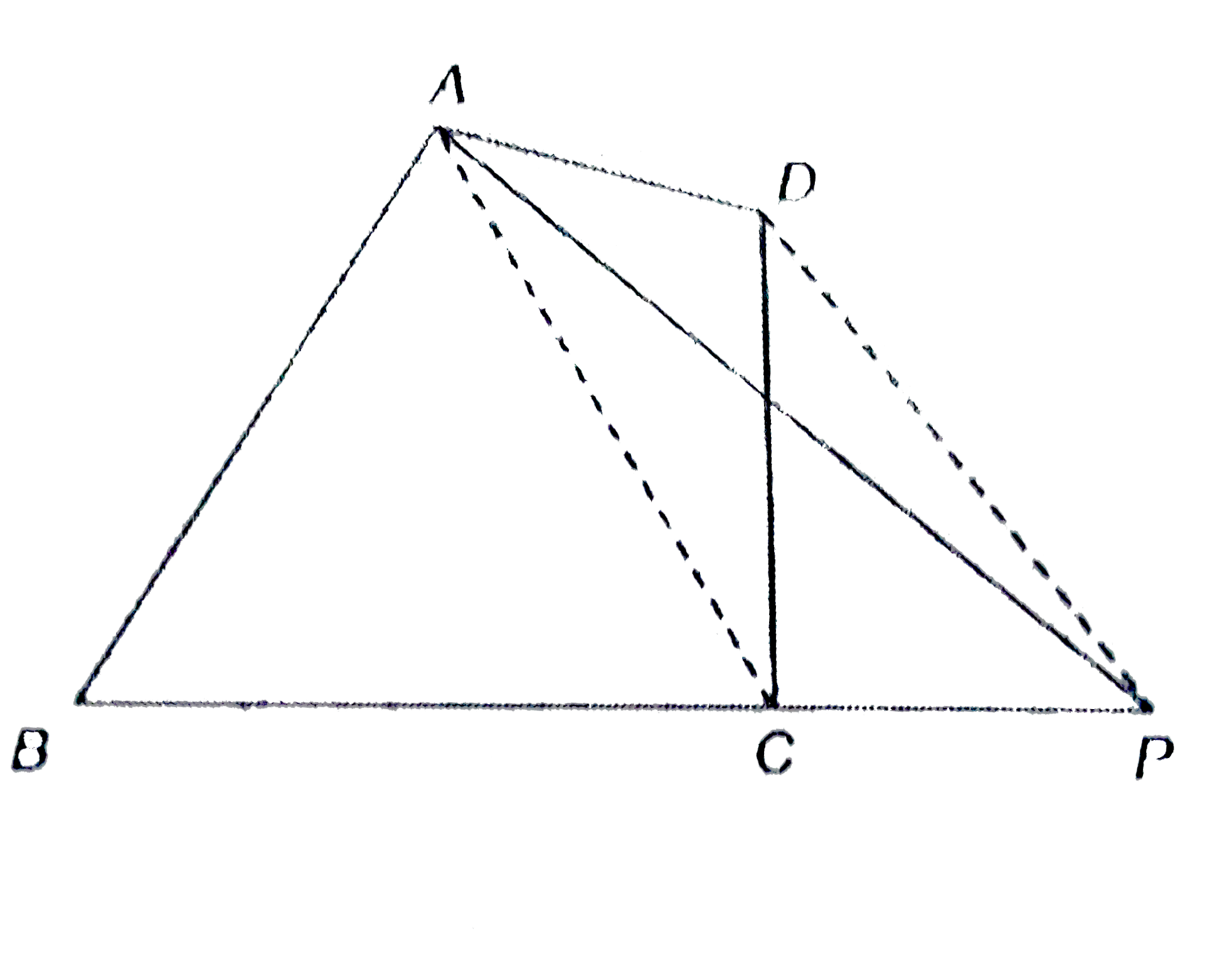
View SolutionIn the figure, ABCD is a quadrilateral. A line DP drawn parallel to diagonal AC from point D, meet BC produced at P. Prove that:
are of ΔABP= area of □ABCD
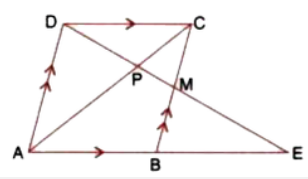
View SolutionIn the following figure, M is mid-point of BC of a parallelogram ABCD. DM intersects the diagonal AC at P and AB produced at E. Prove that : PE = 2 PD .
View SolutionA quadrilateral ABCD is drawn to circumscribe a circle. Prove that AB+CD=AD+BC
View SolutionA quadrilateral ABCD is drawn to circumscribe a circle. Prove that AB+CD=AD+BC
View SolutionA quadrilateral ABCD is drawn to circumscribe a circle. Prove that AB+CD=AD+BC
View SolutionP is the mid-point of diagonal AC of quadrilateral ABCD. Prove that the quadrilaterals ABPD and CBPD are equal in area.
View SolutionIn the adjoining figure, the diagonals AC and BD of a quadrilateral ABCD intersect point O. Prove that :
AB+BC+CD+DA<2(AC+BD)
View SolutionIn the following figure AC=10&BD=14, then area of quadrilateral ABCD-
View Solution
