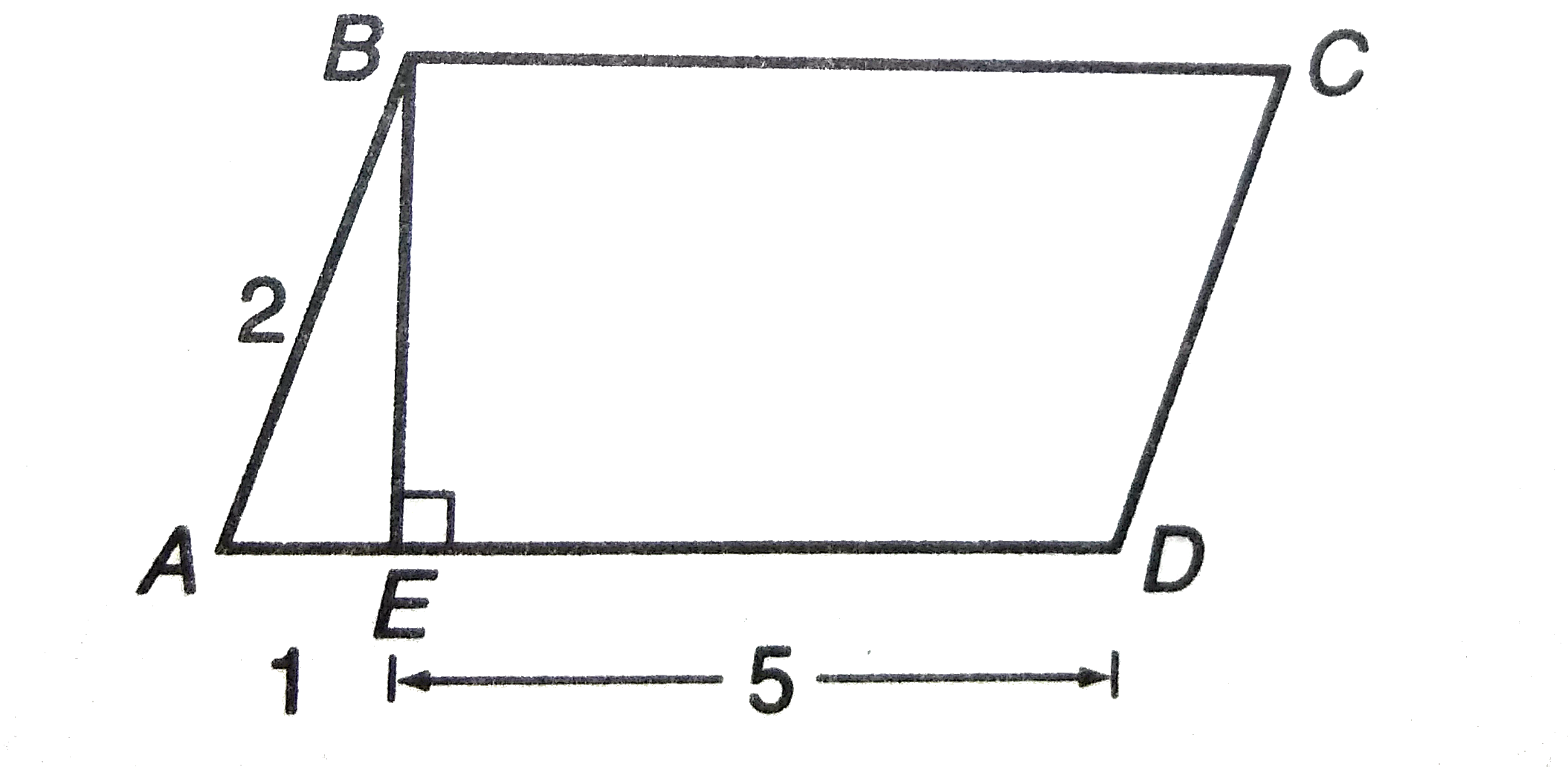
ABCD is a parallelogram. P and Q are the mid-points of sides AB and AD reapectively.
Prove that area of triangle APQ= of the area of parallelogram ABCD.
To prove that the area of triangle APQ is 18 of the area of parallelogram ABCD, we can follow these steps:
Step 1: Identify the midpoints
Let P and Q be the midpoints of sides AB and AD respectively.
Step 2: Draw the diagonals
Draw the diagonal BD of parallelogram ABCD. This diagonal divides the parallelogram into two triangles: ABD and BCD.
Step 3: Analyze triangle APD
Since Q is the midpoint of AD, triangle APD can be analyzed. The area of triangle APD can be expressed as:
Area of △APD=12×Base (AD)×Height from P to AD
Step 4: Use the midpoint theorem
Since P is the midpoint of AB, line segment PQ will be parallel to BD and will also be half the length of BD (by the midpoint theorem). Therefore, triangle APQ is similar to triangle ABD.
Step 5: Relate areas of triangles
The area of triangle APQ can be expressed in terms of triangle APD:
Area of △APQ=12×Area of △APD
Step 6: Relate triangle APD to triangle ABD
Since Q is the midpoint of AD, triangle APD is half of triangle ABD:
Area of △APD=12×Area of △ABD
Step 7: Relate triangle ABD to parallelogram ABCD
The area of triangle ABD is half of the area of parallelogram ABCD:
Area of △ABD=12×Area of ABCD
Step 8: Substitute and simplify
Now substituting the area of triangle ABD into the area of triangle APD:
Area of △APD=12×(12×Area of ABCD)=14×Area of ABCD
Now substituting this into the area of triangle APQ:
Area of △APQ=12×(14×Area of ABCD)=18×Area of ABCD
Conclusion
Thus, we have shown that:
Area of △APQ=18×Area of ABCD
Let P and Q be the midpoints of sides AB and AD respectively.
Draw the diagonal BD of parallelogram ABCD. This diagonal divides the parallelogram into two triangles: ABD and BCD.
Since Q is the midpoint of AD, triangle APD can be analyzed. The area of triangle APD can be expressed as:
Area of △APD=12×Base (AD)×Height from P to AD
Since P is the midpoint of AB, line segment PQ will be parallel to BD and will also be half the length of BD (by the midpoint theorem). Therefore, triangle APQ is similar to triangle ABD.
The area of triangle APQ can be expressed in terms of triangle APD:
Area of △APQ=12×Area of △APD
Since Q is the midpoint of AD, triangle APD is half of triangle ABD:
Area of △APD=12×Area of △ABD
The area of triangle ABD is half of the area of parallelogram ABCD:
Area of △ABD=12×Area of ABCD
Now substituting the area of triangle ABD into the area of triangle APD:
Area of △APD=12×(12×Area of ABCD)=14×Area of ABCD
Area of △APQ=12×(14×Area of ABCD)=18×Area of ABCD
Thus, we have shown that:
Area of △APQ=18×Area of ABCD
Topper's Solved these Questions
- AREA THEOREMSBOOK - ICSECHAPTER - AREA THEOREMSEXERCISE - Exercies 16(C )
22 Videos
- AREA THEOREMSBOOK - ICSECHAPTER - AREA THEOREMSEXERCISE - Exercies 16(A)
36 Videos
- AREA AND PERIMETER OF PLANE FIGURESBOOK - ICSECHAPTER - AREA AND PERIMETER OF PLANE FIGURESEXERCISE - EXERCISE 20(D)
12 Videos
- CHAPTER REVISION (STAGE 2) BOOK - ICSECHAPTER - CHAPTER REVISION (STAGE 2) EXERCISE - DISTANCE FORMULA
12 Videos
Similar Questions
Knowledge Check
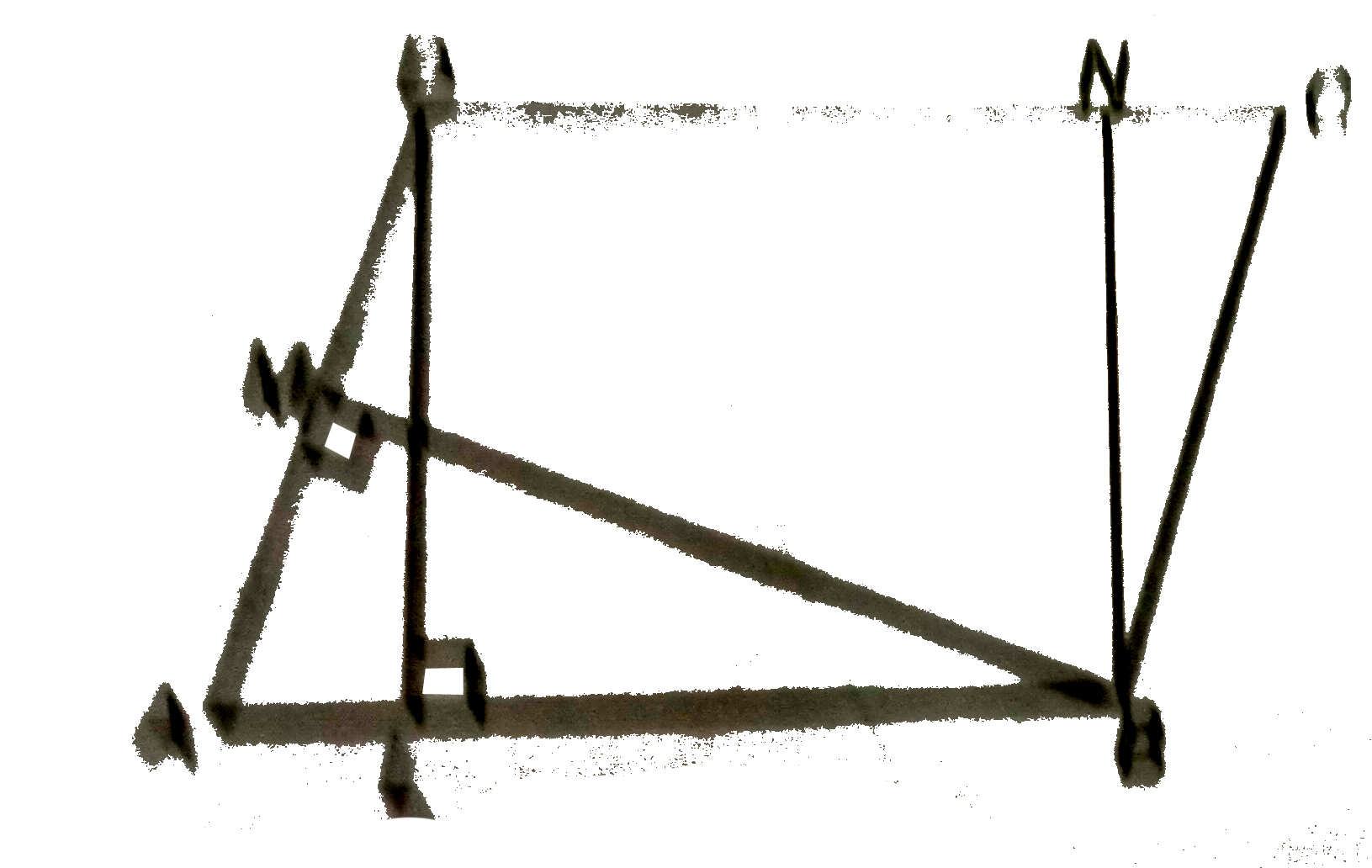
In the figure above, what is the area of parallelogram ABCD?A4√2B4√3C6√2D6√3
E, F, G and H are the mid-points of the sides of a parallelogram ABCD. Show that area of quadrilateral EFGH is half of the area of parallelogram ABCD.
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
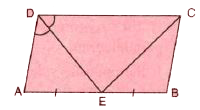
∠DEC=90∘
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
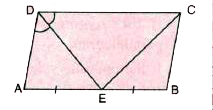
CE bisects angle C
View SolutionABCD is a parallelogram. Two points P and Q are taken on sides AD and BC respectively such that AP 13ADandCQ=13BC. Prove that □AQCP is a parallelogram.
View SolutionD, E and F are the mid-points of the sides BC, CA and AB respectively of triangle ABC. Prove that:
BDEF is a parallelogram.
View SolutionIn parallelogram ABCD, X and Y are mid-points of opposite sides AB and DC respectively. Prove that:
(i) AX=YC.
(ii) AX is parallel to YC
(iii) AXCY is a parallelogram.
View SolutionIn the given figure, M and N are the mid-points of the sides DC and AB respectively of the parallelogram ABCD.
If the area of parallelogram ABCD is 48cm2,
(i) State the area of the triangle BEC.
(ii) name the parallelofram which is equal in area to the triangle BEC.
View Solution