Starting with an expression for refraction at a single spherical surface, obtain Lens Maker.s formula.
To derive the Lens Maker's formula starting from the expression for refraction at a single spherical surface, we will follow these steps:
Step 1: Understand Refraction at a Spherical Surface
Consider a spherical surface separating two media with refractive indices μ1 and μ2. Let R1 be the radius of curvature of the first surface (convex surface) and R2 be the radius of curvature of the second surface (concave surface).
Step 2: Set Up the Refraction Equation
Using the refraction formula at a spherical surface, we have:
μ1−u+μ2v=μ2−μ1R1
Where:
- u is the object distance,
- v is the image distance.
Step 3: Apply the Second Surface Refraction
For the second surface, we can write a similar equation. Since the light is passing from medium 2 to medium 1, we have:
μ2v+μ1−v′=μ1−μ2R2
Where v′ is the image distance for the second surface.
Step 4: Combine the Equations
For a thin lens, the distances v and v′ can be considered equal, and we can combine the two equations.
Adding both equations gives:
μ1−u+μ2v+μ2v+μ1−v′=μ2−μ1R1+μ1−μ2R2
Step 5: Simplify the Equation
Since v=−v′ for a thin lens, we can simplify the equation to:
μ1−u+2μ2v=μ2−μ1R1+μ1−μ2R2
Step 6: Rearranging to Get Lens Maker's Formula
Rearranging the equation leads to:
1f=μ2−μ1μ1(1R1−1R2)
Where f is the focal length of the lens.
Step 7: Final Form of Lens Maker's Formula
Thus, we arrive at the Lens Maker's formula:
1f=(μ−1μ1)(1R1−1R2)
Where μ=μ2μ1.
Consider a spherical surface separating two media with refractive indices μ1 and μ2. Let R1 be the radius of curvature of the first surface (convex surface) and R2 be the radius of curvature of the second surface (concave surface).
Using the refraction formula at a spherical surface, we have:
- u is the object distance,
- v is the image distance.
For the second surface, we can write a similar equation. Since the light is passing from medium 2 to medium 1, we have:
For a thin lens, the distances v and v′ can be considered equal, and we can combine the two equations.
Since v=−v′ for a thin lens, we can simplify the equation to:
Rearranging the equation leads to:
Thus, we arrive at the Lens Maker's formula:
Similar Questions
Obtain an expression for refraction at a single convex spherical surface separating the two media having refractive indices μ1(rarer medium) and μ2(denser medium) i.e., a relation between u,v,μ1,μ2 and R.
View SolutionDerive an expression for refraction at a single (convex) spherical surface, i.e., a relation between u, v, R, n1 (rarer medium) and n2 (denser medium), where the terms have their usual meaning.
View SolutionIn Figure, a point object O is placed in air. A spherical boundary separates two media. Ab is the principall axis. The refractive index above AB is 1.6 and below AB is 2.0. The separation between the images formed due to refraction at a the spherical surface is (assume the radius of curvature (R) to be 1 unit)
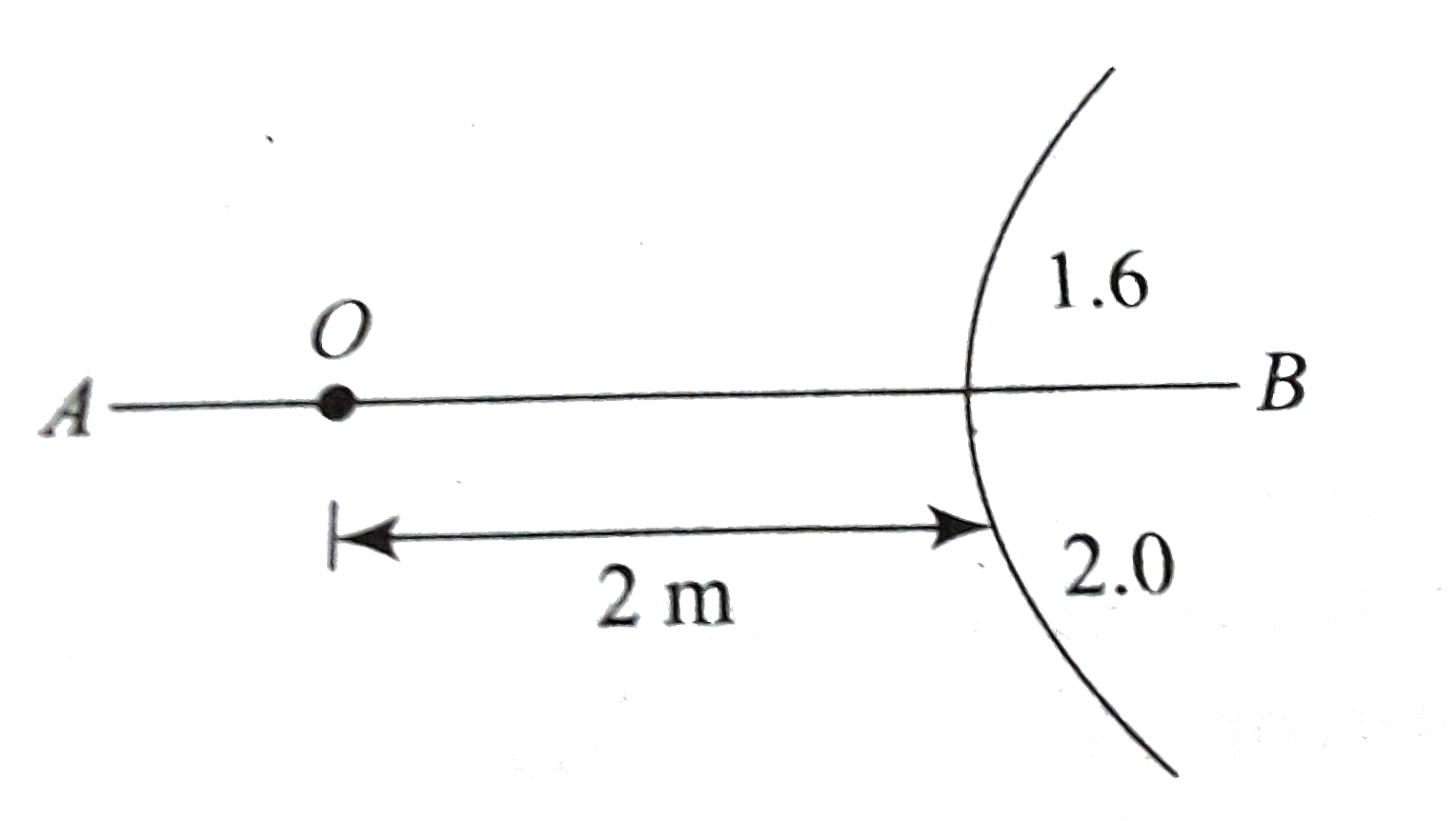 View Solution
View SolutionShow that for refraction at a concave spherical surface (separating glass-air medium), the distance of the object should be greater than three times the radius of curvature of the refracting surface for the image to be real.
View SolutionA person starts with a speed of √(0.5gr) at the top of a large frictionless spherical surface, and slides into the water below (see the drawing). Then , the person
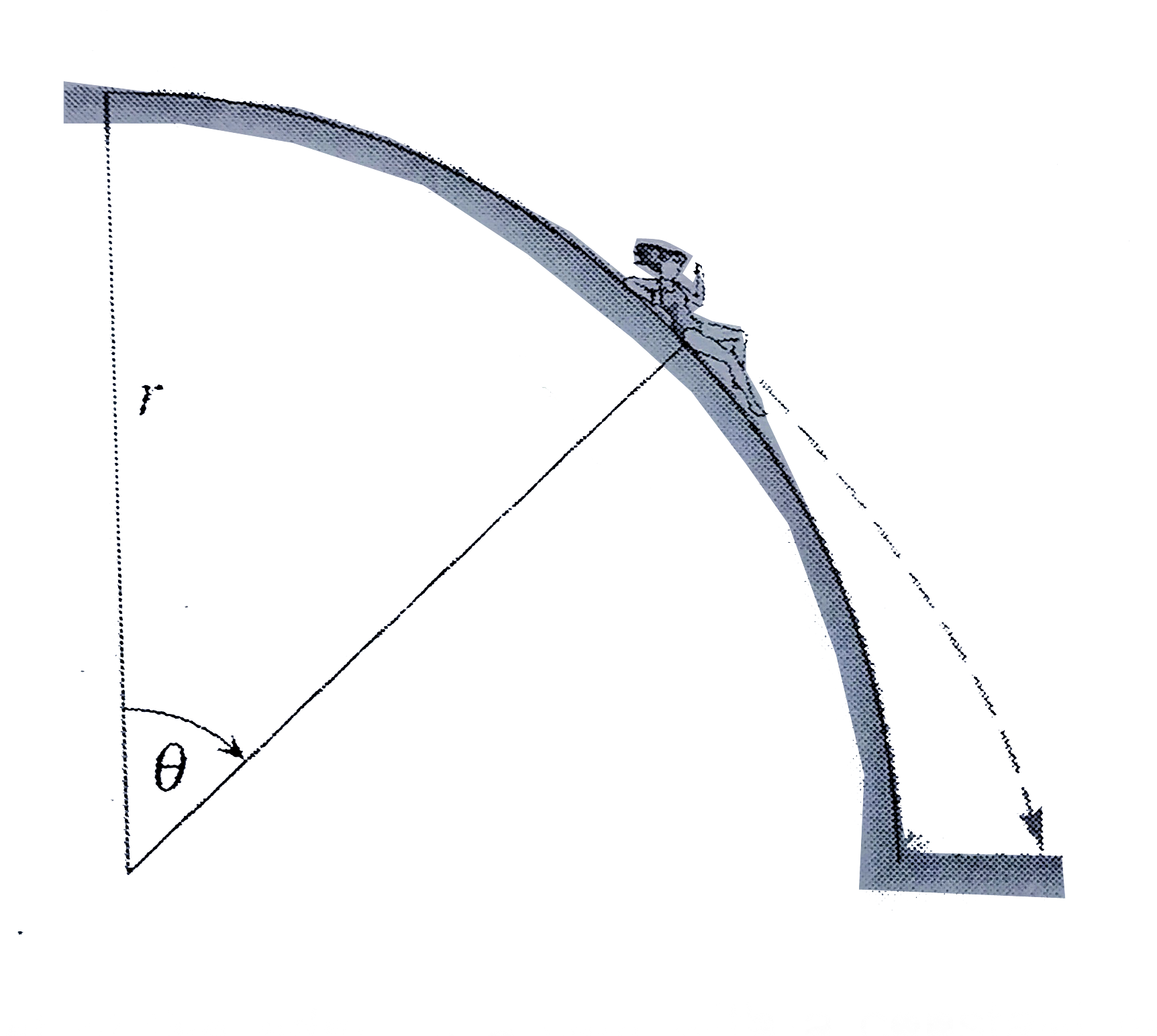 View Solution
View SolutionFigure below shows a parallel beam of monochromatic light incident on a convex spherical surface, radius of curvature R = 30 cm, which separates glass (refractive index - 1 6) from air. Find the position of the image formed due to refraction of light at this single spherical surface.
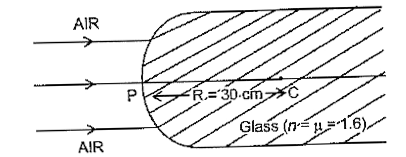 View Solution
View SolutionA parallel narrow beam of light is incident on the surface of a transparent hemisphere of radius R and refractive index μ=1.5 as shown. The position of the image formed by refraction at the image formed by refraction at the spherical surface only as
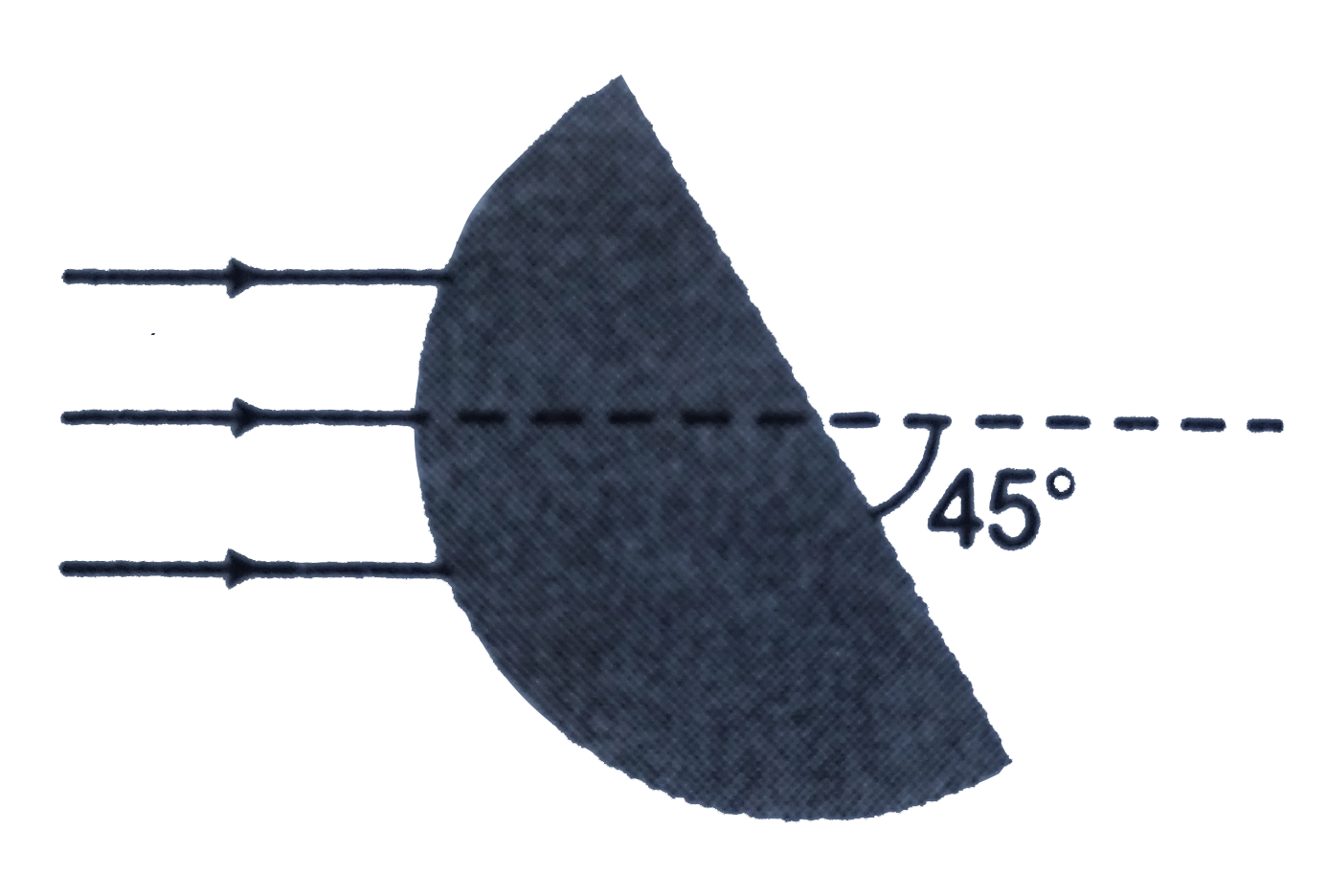 View Solution
View SolutionHow is the refractive index of a medium related to the speed of light ? Obtain an expression for refractive index of a medium with respect to another in terms of speed of light in these two media ?
View SolutionTrace the rays of light showing the formation of an image due to a point object placed on the axis of a spherical surface separating the two media of refractive indices n1andn2. Establish the relation between the distance of the object, the image and the radius of curvature from the central point of the spherical surface.
Hence derive the expression of the lens maker's formulaView SolutionWhen an object is placed 60cm in front of a diverging lens, a virtual image is formed 20cm from the lens. The lens is made of a refractive index μ=1.65 and its two spherical surfaces have the same radius of curvature. What is the value of this radius?
View Solution

