A ray of light is incident on a prism whose refractive index is 1.52 at an angle of 40∘. If the angle of emergence is 60∘, calculate the angle of the prism.
To solve the problem, we will use Snell's law and the relationship between the angles of incidence, refraction, and the prism's angles.
1. Identify Given Values:
- Refractive index of the prism, n=1.52
- Angle of incidence, i=40∘
- Angle of emergence, e=60∘
2. Apply Snell's Law at the First Surface:
Snell's law states that:
n=sinisinr1
Rearranging gives:
sinr1=sinin
3. Calculate sinr1:
Substitute the values:
sinr1=sin40∘1.52
Using sin40∘≈0.643:
sinr1=0.6431.52≈0.423
4. Find r1:
Now, calculate r1:
r1=sin−1(0.423)≈25∘
5. Apply Snell's Law at the Second Surface:
Again using Snell's law:
n=sinesinr2
Rearranging gives:
sinr2=sinen
6. Calculate sinr2:
Substitute the values:
sinr2=sin60∘1.52
Using sin60∘≈0.866:
sinr2=0.8661.52≈0.57
7. Find r2:
Now, calculate r2:
r2=sin−1(0.57)≈35∘
8. Calculate the Angle of the Prism A:
The angle of the prism A is given by:
A=r1+r2=25∘+35∘=60∘
Final Answer:
The angle of the prism A is 60∘.
---
- Refractive index of the prism, n=1.52
- Angle of incidence, i=40∘
- Angle of emergence, e=60∘
Snell's law states that:
n=sinisinr1
Rearranging gives:
sinr1=sinin
Substitute the values:
sinr1=sin40∘1.52
Using sin40∘≈0.643:
sinr1=0.6431.52≈0.423
Now, calculate r1:
r1=sin−1(0.423)≈25∘
Again using Snell's law:
n=sinesinr2
Rearranging gives:
sinr2=sinen
Substitute the values:
sinr2=sin60∘1.52
Using sin60∘≈0.866:
sinr2=0.8661.52≈0.57
Now, calculate r2:
r2=sin−1(0.57)≈35∘
The angle of the prism A is given by:
A=r1+r2=25∘+35∘=60∘
The angle of the prism A is 60∘.
Similar Questions
A prism is made of glass of refractive index 1.5. If the angle of minimum deviation is equal to the refracting angle of the prism, calculate the angle of the prism.
View SolutionA prism of refractive index 1.53 is placed in water of refractive index 1.33. If the angle of prism is 60∘, calculate the angle of minimum deviation in water.
View Solution
Knowledge Check
A ray of light is incident at 60∘ on one face of a prism of angle 30∘ and the emergent ray makes 30∘ with the incident ray. The refractive index of the prism is
A1.732B1.414C1.5D1.33
A ray of light is incident on one face of a prism (m=√3) at an angle of 60∘. The refracting angle of the prism is also 60∘. Find the angle of emergence .
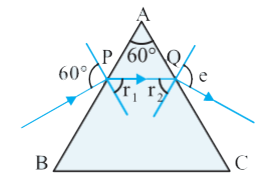
View SolutionA ray of light is incident on one of the faces of the angle prism with an angle 40∘ with the surface. The angle of the prism is 60∘. The emergent ray is deviated through an angle 38∘. The angle of emergence is
View SolutionA ray of light passing through a prism having refractive index √2 suffers minimum deviation. It is found that the angle of incidence is double the angle of refraction within the prism. What is the angle of prism?
View SolutionA ray of light is incident at 60∘ on one face of a prism of angle 30∘ and the emergent ray makes 30∘ with the incident ray. The refractive index of the prism is
View SolutionA ray EF of monochromatic light is incident on the refracting surface AB of a regular glass prism ( refractive index=1.5) at angle of incidence i=55∘ (Figure). If it emerges the adjacent face AC, calculate the angle of emergence .e..
View SolutionA ray of light is incident on a glass prism of refractive index μ and refractive angle A. If it just suffers total internal reflection at the other face, obtain an expression relating the angle of incidence , angle of prism and critical angle.
View SolutionA ray of light is incident at 65∘ on one face of a prism of angle of 30∘ and the emergent ray makes 35∘ with the incident ray. The refractive index of the prism is:
View Solution
