A ray of light is incident on a glass prism of refractive index μ and refractive angle A. If it just suffers total internal reflection at the other face, obtain an expression relating the angle of incidence , angle of prism and critical angle.
To solve the problem, we need to derive an expression relating the angle of incidence (I), the angle of the prism (A), and the critical angle (C) when a ray of light undergoes total internal reflection at the second face of a glass prism.
1. Understanding the Geometry of the Prism:
- Consider a glass prism with a refractive index μ and a refracting angle A.
- A ray of light is incident on the first face of the prism at an angle I.
2. Refraction at the First Face:
- According to Snell's law at the first face of the prism:
n1sinI=n2sinR
where n1=1 (the refractive index of air) and n2=μ (the refractive index of the prism).
- Thus, we have:
sinI=μsinR(1)
3. Understanding Total Internal Reflection:
- At the second face of the prism, the ray of light undergoes total internal reflection. For this to happen, the angle of incidence at this face must be greater than the critical angle C.
- The angle of refraction at the second face is 90∘ when total internal reflection occurs, which means:
R+C=A(2)
- Rearranging gives:
R=A−C
4. Substituting for R:
- Substitute R from equation (2) into equation (1):
sinI=μsin(A−C)(3)
5. Finding the Critical Angle:
- The critical angle C is defined by the relationship:
μsinC=1(4)
- Therefore, we can express μ as:
μ=1sinC(5)
6. Substituting μ into Equation (3):
- Substitute equation (5) into equation (3):
sinI=1sinCsin(A−C)
7. Final Expression for Angle of Incidence:
- Rearranging gives:
sinI=sin(A−C)sinC
- Therefore, we can express the angle of incidence I as:
I=sin−1(sin(A−C)sinC)
Final Result:
The expression relating the angle of incidence I, the angle of the prism A, and the critical angle C is:
I=sin−1(sin(A−C)sinC)
- Consider a glass prism with a refractive index μ and a refracting angle A.
- A ray of light is incident on the first face of the prism at an angle I.
- According to Snell's law at the first face of the prism:
n1sinI=n2sinR
where n1=1 (the refractive index of air) and n2=μ (the refractive index of the prism).
- Thus, we have:
sinI=μsinR(1)
- At the second face of the prism, the ray of light undergoes total internal reflection. For this to happen, the angle of incidence at this face must be greater than the critical angle C.
- The angle of refraction at the second face is 90∘ when total internal reflection occurs, which means:
R+C=A(2)
- Rearranging gives:
R=A−C
- Substitute R from equation (2) into equation (1):
sinI=μsin(A−C)(3)
- The critical angle C is defined by the relationship:
μsinC=1(4)
- Therefore, we can express μ as:
μ=1sinC(5)
- Substitute equation (5) into equation (3):
sinI=1sinCsin(A−C)
- Rearranging gives:
sinI=sin(A−C)sinC
- Therefore, we can express the angle of incidence I as:
I=sin−1(sin(A−C)sinC)
The expression relating the angle of incidence I, the angle of the prism A, and the critical angle C is:
I=sin−1(sin(A−C)sinC)
Topper's Solved these Questions
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTION-C50videos
XII BOARDS
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - PHYSICS (Theory) DELHI BOARD -2016 [SET -I][COMPTT.]11videos
XII BOARDS
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SECTION-A28videos
XII BOARDS
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D6videos
SAMPLE PAPER 2019
Similar Questions
At what angle should a ray of light be incident on the face of a prism of refracting angle 60∘ so that it just suffers total internal reflection at the other face ? The refractive index of the prism is 1.524.
View SolutionA prism of refractive index sqrt2 has refractive angle 60∘. In the order that a ray suffers minimum deviation it should be incident at an angle of
View SolutionA ray of light is incident on the surface of a glass plate of refractive index √3 at the polarising angle . The angle of incidence and angle of refraction of the ray is
View SolutionA ray of light is incident on a prism whose refractive index is 1.52 at an angle of 40∘. If the angle of emergence is 60∘, calculate the angle of the prism.
View SolutionA ray of light falls on a glass plate of refractive index μ=1.5.
What is the angle of incidence of the ray if the angle between the reflected and
refracted rays is 90∘?View SolutionA ray of light falls on a glass plate of refractive index μ=1.5.
What is the angle of incidence of the ray if the angle between the reflected and
refracted rays is 90∘?View SolutionUnpolarized light is incident on a plane surface of glass of refractive index μ at angle i. If the reflected light gets totally polarized, write the relation between the angle i and refractive index μ.
View SolutionA ray of light is incident on a transparent glass slab of refractive index √3. If the reflected and refracted rays are mutually perpendicular, what is the angle of incidence?
 View Solution
View SolutionA ray of light falls normally on one face of a right angled prims of refracting angle A. It suffers two total internal reflections before coming out of prism parallel to incident ray as shown. Refractive index to prism material will be:
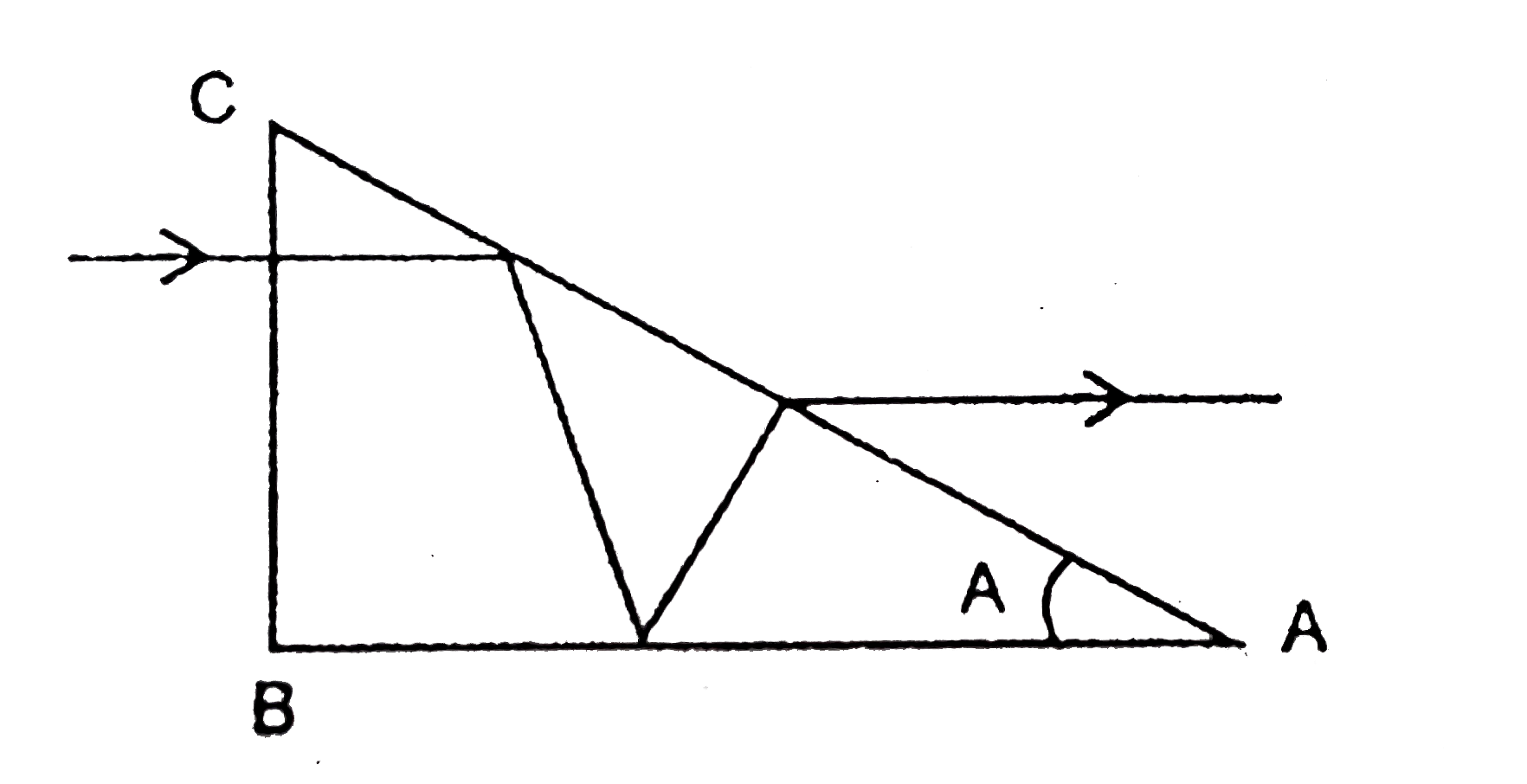 View Solution
View SolutionA ray of light suffers a minimum deviation when incident on equilateral prism of refractive index √2 The angle of deviation is
View Solution

