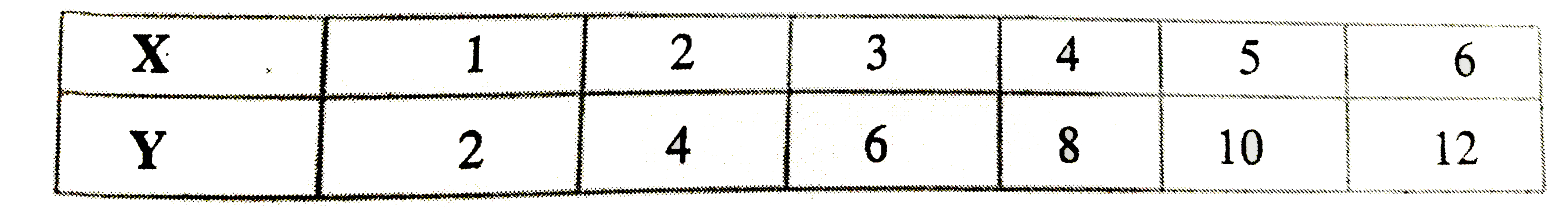
The coefficient of correlation is independent of
change of scale only
change of origin only
both change of scale and change of origin
neither change of scale nor change or origin
The correct Answer is:C
To solve the question regarding the coefficient of correlation and its independence from certain changes, let's break it down step by step.
1. Understanding the Coefficient of Correlation:
The coefficient of correlation (denoted as r) measures the strength and direction of a linear relationship between two variables. It ranges from -1 to 1.
Hint: Remember that r indicates how closely two variables are related.
2. Identifying Changes:
- Change of Scale: This refers to multiplying or dividing all values of a variable by a constant. For example, if we multiply all values of X by a factor k, the relationship between X and Y remains unchanged in terms of correlation.
- Change of Origin: This involves adding or subtracting a constant from all values of a variable. For example, if we add a constant c to all values of X, the correlation remains the same.
Hint: Think of how scaling (multiplying/dividing) and shifting (adding/subtracting) affects the data.
3. Independence of Correlation:
The coefficient of correlation is independent of both change of scale and change of origin. This means that regardless of whether we scale or shift the data, the value of the correlation coefficient r will not change.
Hint: Consider how the relative positions of points in a scatter plot remain the same under these transformations.
4. Conclusion:
Since the coefficient of correlation does not change with either transformation, the correct answer to the question is that it is independent of both change of scale and change of origin.
Final Answer: The coefficient of correlation is independent of both change of scale and change of origin.
The coefficient of correlation (denoted as r) measures the strength and direction of a linear relationship between two variables. It ranges from -1 to 1.
- Change of Scale: This refers to multiplying or dividing all values of a variable by a constant. For example, if we multiply all values of X by a factor k, the relationship between X and Y remains unchanged in terms of correlation.
- Change of Origin: This involves adding or subtracting a constant from all values of a variable. For example, if we add a constant c to all values of X, the correlation remains the same.
The coefficient of correlation is independent of both change of scale and change of origin. This means that regardless of whether we scale or shift the data, the value of the correlation coefficient r will not change.
Since the coefficient of correlation does not change with either transformation, the correct answer to the question is that it is independent of both change of scale and change of origin.
Topper's Solved these Questions
Similar Questions
Knowledge Check
Coefficient of correlation is the measure of
Acentral tendencyBdispresionCboth central tendency and dispersionDneither central tendency nor dispersionBOD is measure of
ACentral tendencyBDispersionCBoth central tendency and dispersionDNeither central tendency nor dispersion.Consider the following statements:
1. Two independent variables are always uncorrelated.
2. The coefficient of correlation between two variables X and Y is positive when X decreases then Y decreases. Which of the above statements is/are correct?A1 onlyB2 onlyCBoth 1 and 2DNeither 1 nor 2
Calculate coefficient of correlation between demand for a good and its price.
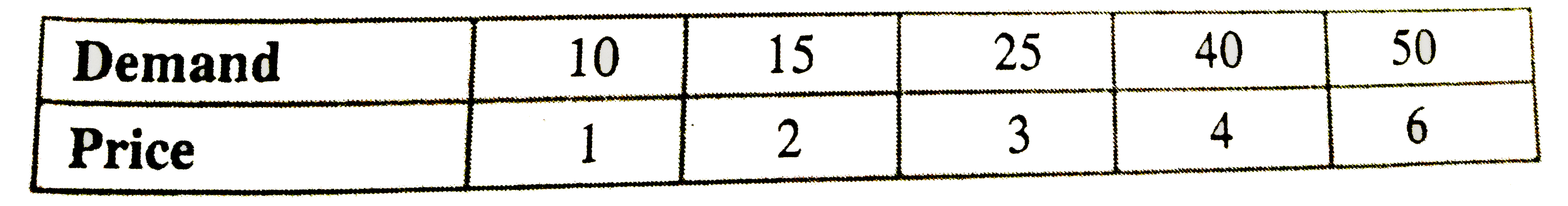
View SolutionThe regression lines will be perpendicular to each other if the coefficient of correlation r is equal to
View SolutionCalculate the coefficient of rank correlation.
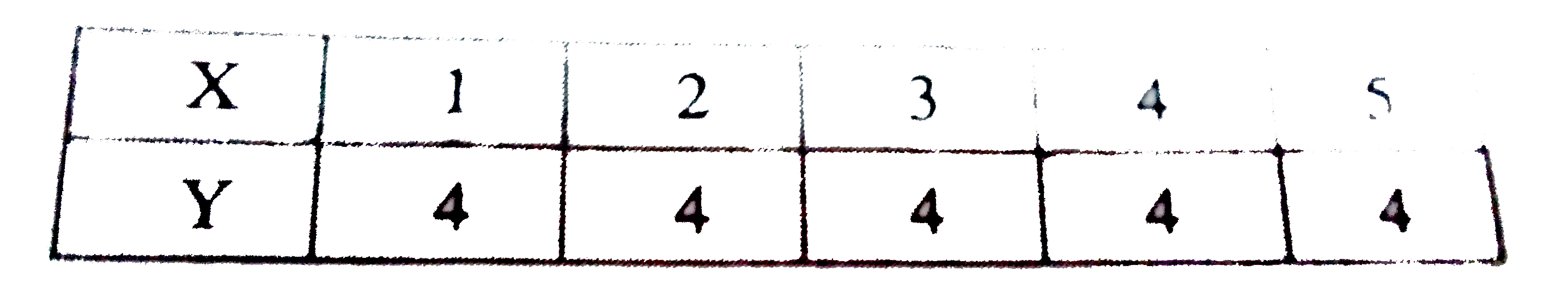
View SolutionThe coefficient of correlation when coefficients of regression are 0.2 and 1.8 is
View SolutionThe coefficient of correlation when coefficients of regression are 0.2 and 1.8 is:
View Solution