What is law of equipartion energy? Show that energy per molecule per degrees of freedom is 12KT.Solution in Assamese
Step by step video & image solution for What is law of equipartion energy? Show that energy per molecule per degrees of freedom is frac(1)(2) KT. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Define gravitational potential.
View SolutionThe moment of inertia of a body depends upon
View SolutionLaw Of Equipartion Of Energy
View SolutionSTATEMENT-1 : The average translational kinetic energy per molecule of the gas per degree of freedom is 1/2 KT.
STATEMENT-2 : For every molecule there are three rotational degree of freedom.
View SolutionFor a gas molecule with 6 degrees of freedom,the law of equipartion of energy gives the following relation between the molecular specific heat (CV) and gas constant (R)
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
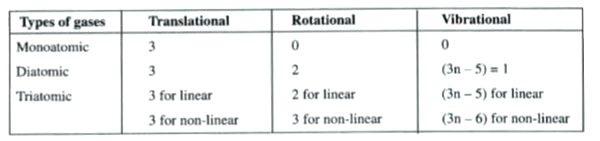
Where 'n' is atomicity of gas.
The vibrational kinetic energy of CO2 molecule is
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are E_(K_(x)), E_(K_(y)), E_(K_(z)) Total K.e =E_(K_(x)) + E_(K_(y)) + E_(K_(z)) Since the motion of molecule is equally probable in all the three directions, therefore E_(K_(x)) = E_(K_(y)) = E_(K_(z)) =1/3 E_(K) =1/3 xx 3/2 kT = 1/2 kT , where k =R/N_(A) = Botzman constant. K.E. = 1/2 kT per molecule or =1/2 RT per mole. In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom. Vibration energy =2 xx 1/2kT =2 xx 1/2RT [ therefore two degrees of freedom per mole] If the gas molecules have n_(1) translational degrees of freedom, n_2 rotational degrees of freedom and n_(3) vibrational degrees of freedom, that total energy = n_(1)[(kT)/2] + n_(2) [(kT)/2] + n_(3) [(kT)/2] xx 2 Where 'n' is atomicity of gas. The rotational kinetic energy of H20 molecule is equal to
View SolutionSTATEMENT-1 : The average translational kinetic energy per molecule of the gas per degree of freedom is 1/2 KT.
STATEMENT-2 : For every molecule there are three rotational degree of freedom.
View SolutionMean kinetic energy per degree of freedom for gas molecule is
View SolutionWhat is the mean energy per molecule for a polyatomic gas with n degrees of freedom?
View SolutionWhat is the knietic energy per degrees of freedom?
View SolutionAt a given temperature what is the mean energy per degree of freedom of any gas ?
View SolutionState law of equiparation of energy. Express the interpretation KE per molecule per degree of freedom.
View SolutionA polyatomic gas with n degrees of freedom has energy per molecule given by :
View SolutionFor a gas molecule with 6 degree of freedom , the law of equipartion of energy gives the following relations between the molecular speecific heat (C_V) and gas constant ( R )
View Solution
