STATEMENT-1 : The average translational kinetic energy per molecule of the gas per degree of freedom is 1/2 KT.
STATEMENT-2 : For every molecule there are three rotational degree of freedom.Solution in Punjabi
If both the statement are TRUE and STATEMENT-2 is the correct explanation of STATEMENT-1
If both the statement are TRUE but STATEMENT-2 is NOT the correct explanation of STATEMENT-1
If STATEMENT-1 is TRUE and STATEMENT-2 is FALSE
If STATEMENT-1 is FALSE and STATEMENT-2 is TRUE
The correct Answer is:C
Step by step video & image solution for STATEMENT-1 : The average translational kinetic energy per molecule of the gas per degree of freedom is 1/2 KT. STATEMENT-2 : For every molecule there are three rotational degree of freedom. by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- GASEOUS STATEBOOK - NARENDRA AWASTHICHAPTER - GASEOUS STATEEXERCISE - Level 1 (Q.151 To Q.176)
1 Videos
- GASEOUS STATEBOOK - NARENDRA AWASTHICHAPTER - GASEOUS STATEEXERCISE - Level 2
1 Videos
- GASEOUS STATEBOOK - NARENDRA AWASTHICHAPTER - GASEOUS STATEEXERCISE - Level 1 (Q.121 To Q.150)
1 Videos
- ELECTROCHEMISTRYBOOK - NARENDRA AWASTHICHAPTER - ELECTROCHEMISTRYEXERCISE - Level 3 - Subjective Problems
1 Videos
- IONIC EEQUILIBRIUMBOOK - NARENDRA AWASTHICHAPTER - IONIC EEQUILIBRIUMEXERCISE - Assertin-Reason Type Questions
1 Videos
Similar Questions
The temperature at which real gases obey the ideal gas laws over a wide range of low pressure is called:
View SolutionThe following organic compound are popularly known by their common names. write their structural formula and iupac name : hydroquinol
View SolutionDimethyl ether decomposes as
CH3OCH3(g)→CH4(g)+CO(g)+H2(g)
When CH3OCH3(g) decomposes to 20% extent under certain conditions, what is the ratio of diffusion of pure CH3OCH3(g) with methane?
View SolutionCalculate the oxidation state of nitrogen ( N ) in NO2
View SolutionSTATEMENT-1: All strong monoprotic acid with same concentration in dilute solution show same pH.
STATEMENT-2: Water shows levelling effect.
View SolutionAssertion : The number of degrees of freedom of triatomic molecules is 6 Reason : Triatomic molecules have three translational degrees of freedom and three rotational degrees of freedom.
View SolutionSTATEMENT-1 : The average translational kinetic energy per molecule of the gas per degree of freedom is 1/2 KT.
STATEMENT-2 : For every molecule there are three rotational degree of freedom.
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
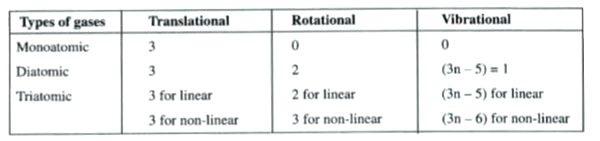
Where 'n' is atomicity of gas.
The vibrational kinetic energy of CO2 molecule is
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are E_(K_(x)), E_(K_(y)), E_(K_(z)) Total K.e =E_(K_(x)) + E_(K_(y)) + E_(K_(z)) Since the motion of molecule is equally probable in all the three directions, therefore E_(K_(x)) = E_(K_(y)) = E_(K_(z)) =1/3 E_(K) =1/3 xx 3/2 kT = 1/2 kT , where k =R/N_(A) = Botzman constant. K.E. = 1/2 kT per molecule or =1/2 RT per mole. In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom. Vibration energy =2 xx 1/2kT =2 xx 1/2RT [ therefore two degrees of freedom per mole] If the gas molecules have n_(1) translational degrees of freedom, n_2 rotational degrees of freedom and n_(3) vibrational degrees of freedom, that total energy = n_(1)[(kT)/2] + n_(2) [(kT)/2] + n_(3) [(kT)/2] xx 2 Where 'n' is atomicity of gas. The rotational kinetic energy of H20 molecule is equal to
View SolutionSTATEMENT-1 : The average translational kinetic energy per molecule of the gas per degree of freedom is 1/2 KT.
STATEMENT-2 : For every molecule there are three rotational degree of freedom.
View Solution(A): Vibrational energy of diatomic molecule corresponding to cach degree of freedom is k, (R): For every molecule, vibrational degree of freedom is 2.
View SolutionThe molecules of an ideal gas have 6 degrees of freedom. The temperature of the gas is T. The average translational kinetic energy of its molecules is
View SolutionMean kinetic energy per degree of freedom for gas molecule is
View SolutionWhat is the mean energy per molecule for a polyatomic gas with n degrees of freedom?
View SolutionThe mean kinetic energy of 1 mole of gas per degree of freedom is
View Solution
