Consider the wall of a dam to be straight with height H and length L. It holds a lake of water of height h(h < H) on one side. Let the density of water be ρw. Denote the torque about the axis along the bottom length of the wall by T1. Denote also a similar torque due to the water up to height h/2 and wall length L/2 by T2. Then T1/T2 (ignore atmospheric pressure) is
2
4
8
16
Step by step video & image solution for Consider the wall of a dam to be straight with height H and length L. It holds a lake of water of height h(h < H) on one side. Let the density of water be rho_w. Denote the torque about the axis along the bottom length of the wall by T_1. Denote also a similar torque due to the water up to height h/2 and wall length L/2 by T_2. Then T_1/T_2 (ignore atmospheric pressure) is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
A wooden cylinder of diameter 4 r, height H and density ρ/3 is kept on a hole of diameter 2 r of a tank, filled with water of density ρ as shown in the
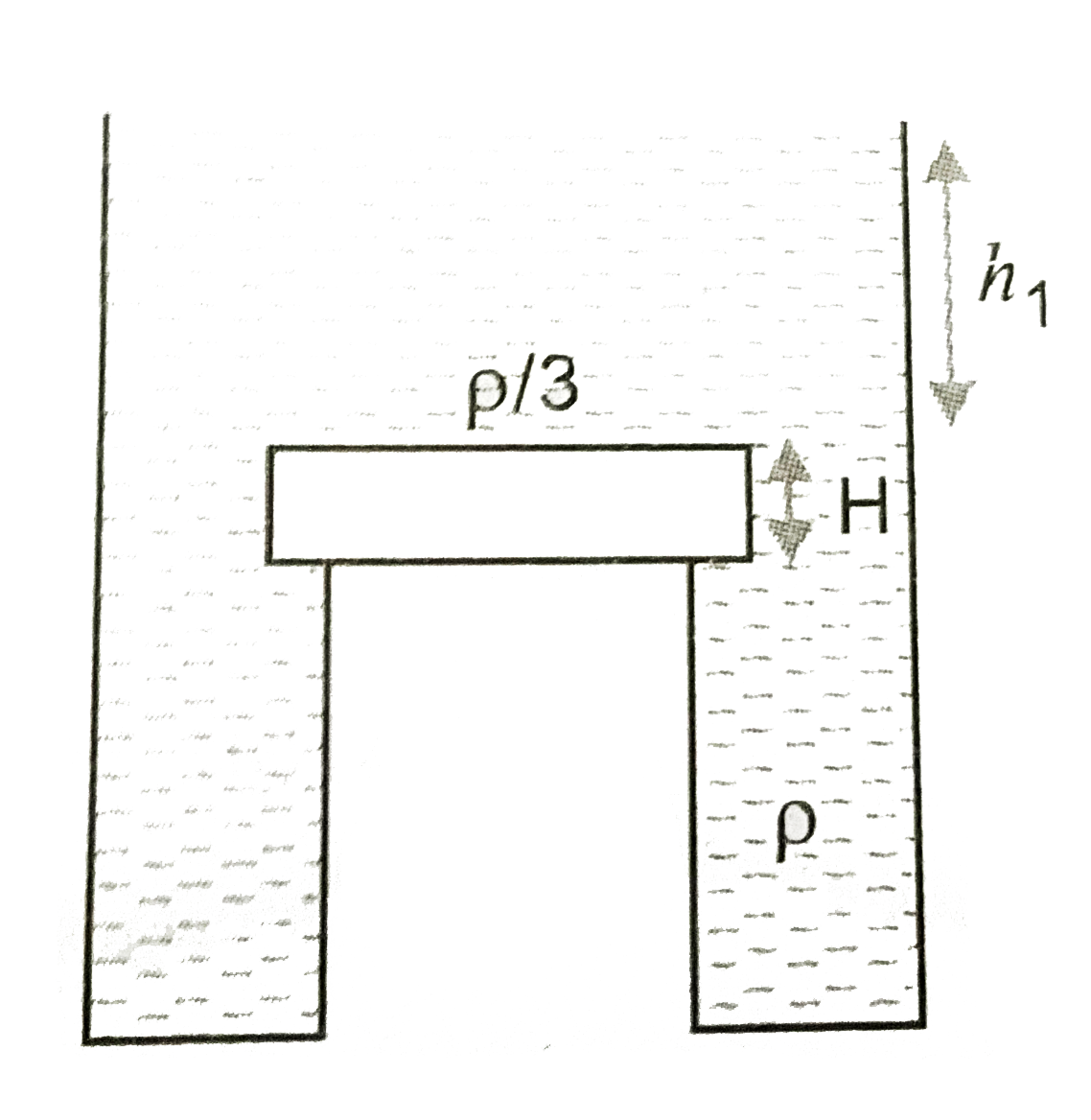
If height h2 of water level is further decreased, thenAcylinder will not move up and remains at its original positionBfor h2=H/3 , cylinder again starts moving upCfor h2=H/4 , cylinder again starts moving upDfor h2=H/5 , cylinder again starts moving upAn air bubble doubles in radius on string from the bottom of a lake to its surface. If the atmospheric pressure is equal to that of a column of water of height H, the depth of the lake is
AHB2HC7HD8HWhen a large bubble rises from the bottom of a lake to the surface, its radius doubles. The atmospheric pressure is equal to that of a column of water of height H. The depth of the lake is
AHB2 HC7 HD8 H
When a large bubble rises from the bottom of a lake to the surface, its radius doubles. The atmospheric pressure is equal to that of a column of water of height H. The depth of the lake is
View SolutionA breaker contains water, up to a height h1 and kerosene of height h2 above water, so that the total height of ( water + kerosene ) is (h1+h2). Refractive index of water is μ1 and that of kerosene is μ2. The apparent shift in the position of the bottom of the beaker when viewed from above is
View SolutionA cylindrical vessel of area of cross-section A is filled with water to a height H. It has capillary tube of length l and radius r fitted horizontally at its bottom. If the coeffiecient of viscosity of water is η, then time required in which level will fall to a height H2 is (density of water is ρ)
View SolutionA cylindrical tank has a hole of diameter 2r in its bottom. The hole is covered wooden cylindrical block of diameter 4r, height h and density ρ/3.

Situation I: Initially, the tank is filled with water of density ρ to a height such that the height of water above the top of the block is h1 (measured from the top of the block).
Situation II: The water is removed from the tank to a height h2 (measured from the bottom of the block), as shown in the figure. The height h2 is smaller than h (height of the block) and thus the block is exposed to the atmosphere.
Find the minimum value of height h1 (in situation 1), for which the block just starts to move up?
View SolutionA cylindrical tank has a hole of diameter 2r in its bottom. The hole is covered wooden cylindrical block of diameter 4r, height h and density ρ/3.

Situation I: Initially, the tank is filled with water of density ρ to a height such that the height of water above the top of the block is h1 (measured from the top of the block).
Situation II: The water is removed from the tank to a height h2 (measured from the bottom of the block), as shown in the figure. The height h2 is smaller than h (height of the block) and thus the block is exposed to the atmosphere.
In situation 2, if h2 is further decreased, then
View Solution
