A particle of mass m oscillates with simple harmonic motion between points x1 and x2, the equilibrium position being O. Its potential energy is plotted. It will be as given below in the graph




The correct Answer is:C
To solve the problem of determining the potential energy graph of a particle of mass m oscillating in simple harmonic motion (SHM) between points x1 and x2 with the equilibrium position at O, we can follow these steps:
Step 1: Understand the potential energy in SHM
The potential energy U of a particle in simple harmonic motion is given by the formula:
U=12kx2
where k is the spring constant and x is the displacement from the equilibrium position O.
Step 2: Identify the displacement
In this case, the particle oscillates between points x1 and x2. The equilibrium position O is the midpoint between these two points. Thus, the maximum displacement from the equilibrium position is:
A=x2−x12
Step 3: Analyze the potential energy at maximum displacement
At maximum displacement (either at x1 or x2), the potential energy will be:
Umax=12kA2
At the equilibrium position O, the potential energy is:
U(O)=0
Step 4: Determine the shape of the graph
Since the potential energy is proportional to the square of the displacement, the graph of potential energy U versus displacement x will be a parabola opening upwards. The vertex of this parabola (where U=0) is at the equilibrium position O.
Step 5: Identify the correct graph
From the options provided, we need to find a graph that:
- Is a parabola opening upwards.
- Has its vertex at the origin (equilibrium position O).
- Passes through the points corresponding to maximum displacements x1 and x2 where the potential energy is at its maximum.
After analyzing the options, we find that option C fits these criteria.
Final Answer
Thus, the correct option representing the potential energy of the particle oscillating in simple harmonic motion is Option C.
---
The potential energy U of a particle in simple harmonic motion is given by the formula:
U=12kx2
where k is the spring constant and x is the displacement from the equilibrium position O.
In this case, the particle oscillates between points x1 and x2. The equilibrium position O is the midpoint between these two points. Thus, the maximum displacement from the equilibrium position is:
A=x2−x12
At maximum displacement (either at x1 or x2), the potential energy will be:
Umax=12kA2
At the equilibrium position O, the potential energy is:
U(O)=0
Since the potential energy is proportional to the square of the displacement, the graph of potential energy U versus displacement x will be a parabola opening upwards. The vertex of this parabola (where U=0) is at the equilibrium position O.
From the options provided, we need to find a graph that:
- Is a parabola opening upwards.
- Has its vertex at the origin (equilibrium position O).
- Passes through the points corresponding to maximum displacements x1 and x2 where the potential energy is at its maximum.
Thus, the correct option representing the potential energy of the particle oscillating in simple harmonic motion is Option C.
Similar Questions
A particle oscillates simple harmonic motion with a period of 16s. Two second after crossing the equilibrium position its velocity becomes 1m/s. The amplitude is
View SolutionA particle is vibrating in a simple harmonic motion with an amplitude of 4 cm . At what displacement from the equilibrium position, is its energy half potential and half kinetic
View SolutionA particle executes simple harmonic motion with an amplitude of 10 cm. At what distance from the mean position are the kinetic and potential energies equal?
View SolutionA particle starts oscillating simple harmonically from its equilibrium position then, the ratio of kinetic energy and potential energy of the particle at the time T/12 is: (T=time period)
View SolutionA particle executing harmonic motion is having velocities v1 and v2 at distances is x1 and x2 from the equilibrium position. The amplitude of the motion is
View SolutionA particle of mass 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is

View SolutionA particle of mass 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is

View SolutionThe total energy of a particle, executing simple harmonic motion is.
where x is the displacement from the mean position, hence total energy is independent of x.
View SolutionA particle of mass = 2kg moves in simple harmonic motion and its potential energy U varies with position x as shown. The period of oscillation of the particle is nπ5 second. Find value of n.
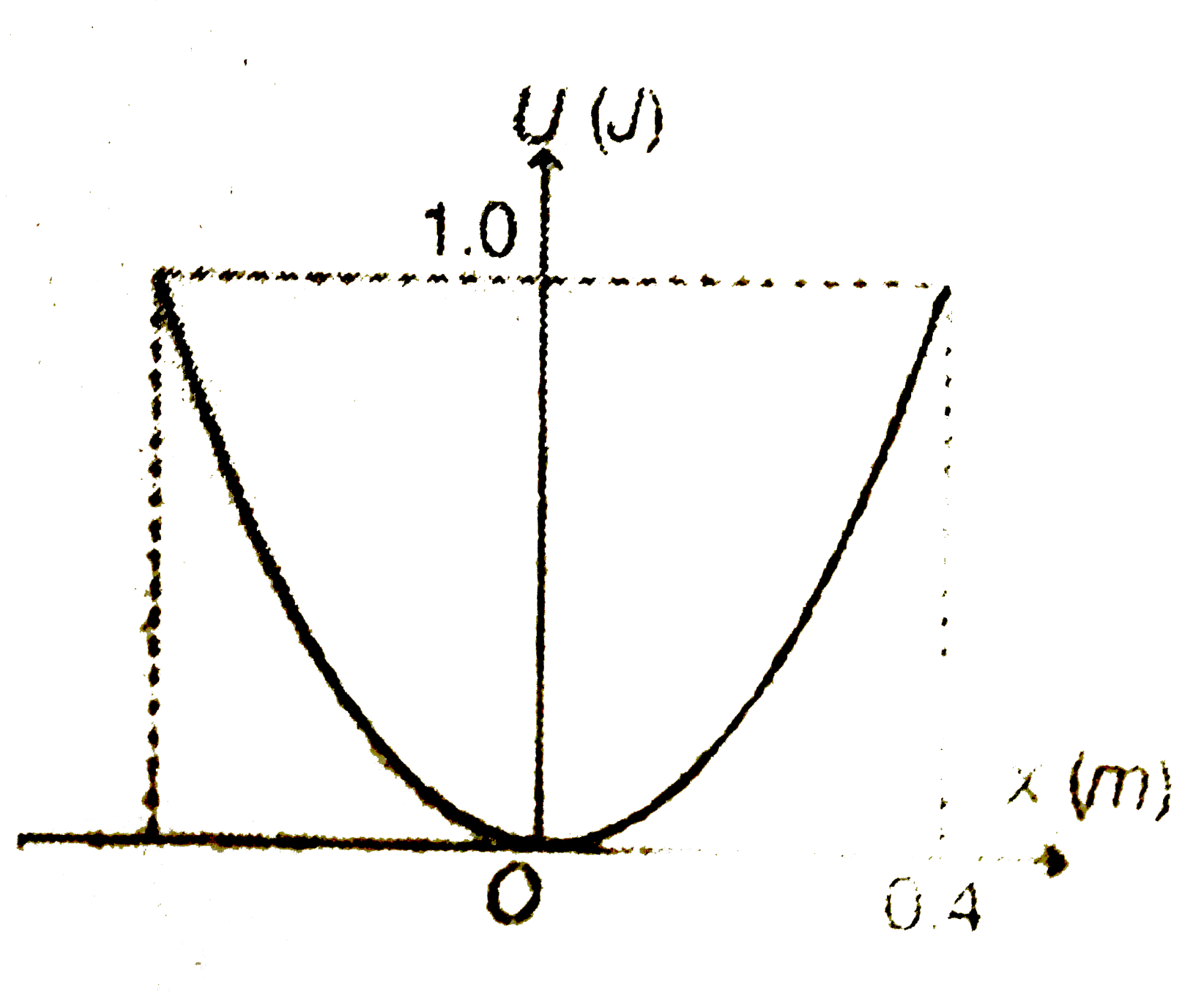
View SolutionA particle of mass 4kg moves simple harmonically such that its PE (U) varies with position x, as shown. The period of oscillations is :-
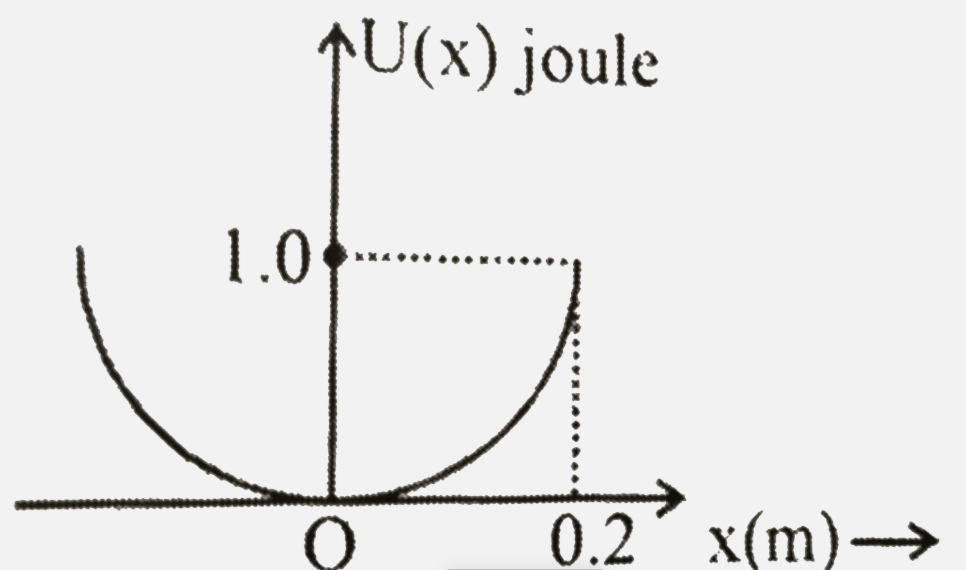
View Solution
