Starting from rest , a body slides down at 45∘ inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is
1. 0.8
2. 0.75
3. 0.25
4. 0.33
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Define the variables
Let:
- d = distance slid down the incline
- t1 = time taken to slide down the incline without friction
- t2=2t1 = time taken to slide down the incline with friction
- g = acceleration due to gravity
- θ=45∘ = angle of the incline
- μ = coefficient of friction
Step 2: Write the equations for distance
In the absence of friction, the distance d can be expressed as:
d=12a1t21
where a1=gsinθ.
With friction, the distance can be expressed as:
d=12a2(2t1)2=2a2t21
where a2=gsinθ−μgcosθ.
Step 3: Set the equations equal to each other
Since both expressions represent the same distance d, we can set them equal:
12a1t21=2a2t21
Step 4: Cancel t21 and simplify
Cancelling t21 from both sides gives:
12a1=2a2
This simplifies to:
a1=4a2
Step 5: Substitute for a1 and a2
Substituting the expressions for a1 and a2:
gsinθ=4(gsinθ−μgcosθ)
Step 6: Factor out g and simplify
Dividing through by g (assuming g≠0):
sinθ=4(sinθ−μcosθ)
Expanding this gives:
sinθ=4sinθ−4μcosθ
Rearranging yields:
4μcosθ=3sinθ
Step 7: Solve for μ
Dividing both sides by 4cosθ:
μ=34tanθ
Step 8: Substitute θ=45∘
Since tan45∘=1:
μ=34⋅1=0.75
Final Answer
The coefficient of friction μ between the body and the inclined plane is:
μ=0.75
---
Let:
- d = distance slid down the incline
- t1 = time taken to slide down the incline without friction
- t2=2t1 = time taken to slide down the incline with friction
- g = acceleration due to gravity
- θ=45∘ = angle of the incline
- μ = coefficient of friction
In the absence of friction, the distance d can be expressed as:
d=12a1t21
where a1=gsinθ.
d=12a2(2t1)2=2a2t21
where a2=gsinθ−μgcosθ.
Since both expressions represent the same distance d, we can set them equal:
12a1t21=2a2t21
Cancelling t21 from both sides gives:
12a1=2a2
This simplifies to:
a1=4a2
Substituting the expressions for a1 and a2:
gsinθ=4(gsinθ−μgcosθ)
Dividing through by g (assuming g≠0):
sinθ=4(sinθ−μcosθ)
Expanding this gives:
sinθ=4sinθ−4μcosθ
Rearranging yields:
4μcosθ=3sinθ
Dividing both sides by 4cosθ:
μ=34tanθ
Since tan45∘=1:
μ=34⋅1=0.75
The coefficient of friction μ between the body and the inclined plane is:
μ=0.75
Similar Questions
Starting from rest a body slides down a 45∘ inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of the body and the inclined plane is :
View SolutionA piece of ice slides down a 45∘ incline in twice the time it takes to slide down a frictionless 45∘ incline . What is the coefficient of friction between the ice and the incline ? .
View Solution
Knowledge Check
Starting from rest, a body slides down a 45∘ inclined plane in twice the time it itakes to slide the same distance in the absence of friction.
They the coefficient of friction between the body and the inclined plane isA1/4B2/3C3/4D√3/2When a body slides down from rest along a smooth inclined plane making an angle of 30∘ with the horizontal, it takes time 20s. When the same body slides down from rest along a rough inclined plane making the same angle and through the same distance, it takes time 20p is, where p is some number greater than 1. The coefficient of friction between the body and the rough plane is
Aμ=(1−1p2)1√3Bμ=(1−19p2)Cμ=(1−p2)1√3Dμ=√1−9p2
The time taken by a body to slide down a rough 45∘ inclined plane is twice that required to slide down a smooth 45∘ inclined plane. The coefficient of kinetic friction between the object and rough plane is given by
View SolutionTwo identical blocks A and B of equal masses are placed on rough inclined plane as shown in the figure. Initially the block A is √2m behind the block B. Coefficient of kinetic friction for the blocks A and B are 0.2 and 0.3 respectively (g=10m/s2). By the time the two blocks come on the same line on the inclined plane if they are released simultaneously, the distance moved by B is βm Find the value of β
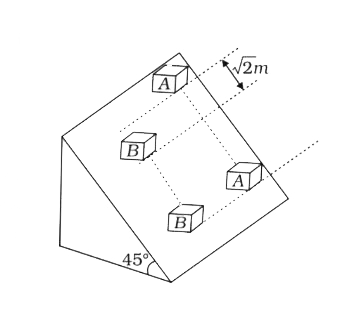
View SolutionThe minimum force required to move a body up an inclined plane is three times the minimum force required to prevent it from sliding down the plane. If the coefficient of friction between the body and the inclined plane is 12√3, the angle of the inclined plane is
View SolutionA given object taken n time more time to slide down 45∘ rough inclined plane as it taken to slide down a perfectly smooth 45∘ incline The coefficient of kintic friction between the object and the incline is .
View SolutionIf the angle of inclination of the inclined plane is sin−1(12) when the body just starts sliding find the angle of repose and coefficient of static friction between the body and the inclined plane.
View SolutionA block of mass m slides down an inclined plane which makes an angle θ with the horizontal. The coefficient of friction between the block and the plane is μ. The force exerted by the block on the plane is
View Solution
