A given object taken n time more time to slide down 45∘ rough inclined plane as it taken to slide down a perfectly smooth 45∘ incline The coefficient of kintic friction between the object and the incline is .
1−1n2
√11−n2
√1−1n2
12−n2
The correct Answer is:A
To solve the problem, we need to analyze the motion of an object sliding down two different inclined planes: one smooth and one rough. We'll derive the coefficient of kinetic friction based on the given information.
1. Define Variables:
- Let the length of the incline be s.
- Let the time taken to slide down the smooth incline be t.
- The time taken to slide down the rough incline is n⋅t.
- The angle of inclination θ=45∘.
2. Acceleration on Smooth Incline:
- For the smooth incline, the only force acting along the incline is the component of gravitational force.
- The acceleration a on the smooth incline is given by:
a=gsinθ=gsin45∘=g√2
- Using the second equation of motion s=ut+12at2 (where u=0):
s=0+12(g√2)t2
s=g2√2t2(Equation 1)
3. Acceleration on Rough Incline:
- For the rough incline, the forces acting on the object include the gravitational component and the frictional force.
- The net acceleration a′ is given by:
a′=gsinθ−μgcosθ=gsin45∘−μgcos45∘=g√2−μg√2=g(1−μ)√2
- The time taken to slide down the rough incline is n⋅t:
s=0+12a′(nt)2
s=12(g(1−μ)√2)(nt)2
s=g(1−μ)2√2n2t2(Equation 2)
4. Equating the Two Equations:
- Since both expressions represent the same distance s, we can set Equation 1 equal to Equation 2:
g2√2t2=g(1−μ)2√2n2t2
- We can cancel g2√2t2 from both sides (assuming t≠0):
1=(1−μ)n2
- Rearranging gives:
1−μ=1n2
μ=1−1n2
5. Final Result:
- The coefficient of kinetic friction μ is given by:
μ=1−1n2
- Let the length of the incline be s.
- Let the time taken to slide down the smooth incline be t.
- The time taken to slide down the rough incline is n⋅t.
- The angle of inclination θ=45∘.
- For the smooth incline, the only force acting along the incline is the component of gravitational force.
- The acceleration a on the smooth incline is given by:
a=gsinθ=gsin45∘=g√2
- Using the second equation of motion s=ut+12at2 (where u=0):
s=0+12(g√2)t2
s=g2√2t2(Equation 1)
- For the rough incline, the forces acting on the object include the gravitational component and the frictional force.
- The net acceleration a′ is given by:
a′=gsinθ−μgcosθ=gsin45∘−μgcos45∘=g√2−μg√2=g(1−μ)√2
- The time taken to slide down the rough incline is n⋅t:
s=0+12a′(nt)2
s=12(g(1−μ)√2)(nt)2
s=g(1−μ)2√2n2t2(Equation 2)
- Since both expressions represent the same distance s, we can set Equation 1 equal to Equation 2:
g2√2t2=g(1−μ)2√2n2t2
- We can cancel g2√2t2 from both sides (assuming t≠0):
1=(1−μ)n2
- Rearranging gives:
1−μ=1n2
μ=1−1n2
- The coefficient of kinetic friction μ is given by:
μ=1−1n2
Topper's Solved these Questions
Similar Questions
The time taken by a body to slide down a rough 45∘ inclined plane is twice that required to slide down a smooth 45∘ inclined plane. The coefficient of kinetic friction between the object and rough plane is given by
View SolutionA piece of ice slides down a 45∘ incline in twice the time it takes to slide down a frictionless 45∘ incline . What is the coefficient of friction between the ice and the incline ? .
View Solution
Knowledge Check
Starting from rest, a body slides down a 45∘ inclined plane in twice the time it itakes to slide the same distance in the absence of friction.
They the coefficient of friction between the body and the inclined plane isA1/4B2/3C3/4D√3/2
A body takes 1(13) times as much time to slide down a rough inclined plane as it takes to slide down an identical bust smooth inclined plane. If the angle of inclination is 45°, find the coefficient of friction.
View SolutionTwo identical blocks A and B of equal masses are placed on rough inclined plane as shown in the figure. Initially the block A is √2m behind the block B. Coefficient of kinetic friction for the blocks A and B are 0.2 and 0.3 respectively (g=10m/s2). By the time the two blocks come on the same line on the inclined plane if they are released simultaneously, the distance moved by B is βm Find the value of β
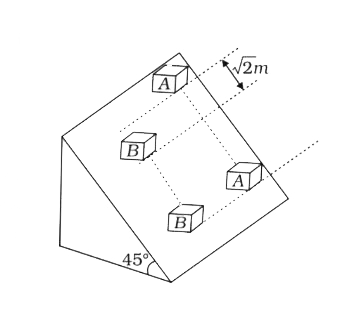
View SolutionA body takes ''n'' times as much time to slide down a rough inclined plane as it takes to slide down an identical but smooth inclined plane. If the angle of inclination of the inclined plane is ''θ''. What is the coefficient of friction between the body and the rough plane ?
View SolutionStarting from rest , a body slides down at 45∘ inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of friction between the body and the inclined plane is
View SolutionAn object takes 1 second to slide down a rough 45∘ inclined plane. The time taken to slide down a smooth 30∘ inclined plane having the same slope length is (μ=0.5)
View SolutionA block of mass m slides down a rough inclined plane with an acceleration g2

View SolutionStarting from rest a body slides down a 45∘ inclined plane in twice the time it takes to slide down the same distance in the absence of friction. The coefficient of the body and the inclined plane is :
View Solution
