The quantities of heat required to raise the temperature of two solid copper spheres of radii r1 and r2 (r1=1.5r2) through 1K are in ratio
27/8
44078
43892
43954
To solve the problem of finding the ratio of the quantities of heat required to raise the temperature of two solid copper spheres of radii r1 and r2 (where r1=1.5r2) through 1K, we can follow these steps:
1. Understand the Heat Required Formula:
The heat Q required to raise the temperature of an object is given by the formula:
Q=mcΔT
where m is the mass, c is the specific heat capacity, and ΔT is the change in temperature.
2. Identify the Variables:
Since both spheres are made of copper, they have the same specific heat capacity c and the same change in temperature ΔT=1K. Therefore, the heat required for each sphere will depend only on their masses.
3. Calculate the Mass of Each Sphere:
The mass m of a sphere can be expressed in terms of its volume and density:
m=density×volume
The volume V of a sphere is given by:
V=43πr3
Thus, the mass of the spheres can be expressed as:
m1=ρ⋅V1=ρ⋅43πr31
m2=ρ⋅V2=ρ⋅43πr32
where ρ is the density of copper.
4. Express the Heat for Each Sphere:
The heat required for each sphere can now be written as:
Q1=m1cΔT=(ρ⋅43πr31)cΔT
Q2=m2cΔT=(ρ⋅43πr32)cΔT
5. Find the Ratio of Heat Quantities:
The ratio of the heat quantities Q1 and Q2 is:
Q1Q2=m1m2=ρ⋅43πr31ρ⋅43πr32
The constants cancel out, leading to:
Q1Q2=r31r32
6. Substitute the Given Ratio:
Since r1=1.5r2:
Q1Q2=(1.5r2)3r32=1.53⋅r32r32
The r32 cancels out, giving:
Q1Q2=1.53
7. Calculate 1.53:
1.53=278
8. Final Ratio:
Thus, the ratio of the quantities of heat required to raise the temperature of the two spheres is:
Q1Q2=278
Conclusion:
The final answer is 278.
The heat Q required to raise the temperature of an object is given by the formula:
Q=mcΔT
where m is the mass, c is the specific heat capacity, and ΔT is the change in temperature.
Since both spheres are made of copper, they have the same specific heat capacity c and the same change in temperature ΔT=1K. Therefore, the heat required for each sphere will depend only on their masses.
The mass m of a sphere can be expressed in terms of its volume and density:
m=density×volume
The volume V of a sphere is given by:
V=43πr3
Thus, the mass of the spheres can be expressed as:
m1=ρ⋅V1=ρ⋅43πr31
m2=ρ⋅V2=ρ⋅43πr32
where ρ is the density of copper.
The heat required for each sphere can now be written as:
Q1=m1cΔT=(ρ⋅43πr31)cΔT
Q2=m2cΔT=(ρ⋅43πr32)cΔT
The ratio of the heat quantities Q1 and Q2 is:
Q1Q2=m1m2=ρ⋅43πr31ρ⋅43πr32
The constants cancel out, leading to:
Q1Q2=r31r32
Since r1=1.5r2:
Q1Q2=(1.5r2)3r32=1.53⋅r32r32
The r32 cancels out, giving:
Q1Q2=1.53
1.53=278
Thus, the ratio of the quantities of heat required to raise the temperature of the two spheres is:
Q1Q2=278
The final answer is 278.
Similar Questions
The capacitance of two concentric spherical shells of radii R1 and R2(R2>R1) is
View SolutionAmount of heat energy required to raise the temperature of 2 g neon gas by 54°F at constant volume is (R= 2 cal mol−1K−1)
View Solution50 cal of heat is required to raise the temperature of 1 mole of an ideal gas from 20∘C to 25∘C, while the pressure of the gas is kept constant. The amount of heat required to raise the temperature of the same has through same temperature range at constant volume is ( R = 2 cal/mol/K)
View SolutionThe figure shows a system of two concentric spheres of radii r1andr2 are kept at temperature T1andT2, respectively. The radial rate of flow of heat in a substance between the two concentric spheres is proportional to
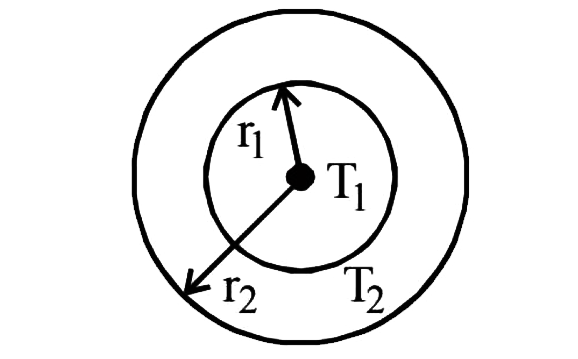
View SolutionThe length of the common chord of two circles of radii r1 and r2 which intersect at right angles is
View SolutionThe figure shows a system of two concentric spheres of radii r 1 and r 2 and kept at temperature T 1 and T 2 , respectively. The radial rate of flow of heat in a substance between the two concentric spheres, is proportional to:
View SolutionA semicircular metallic disc of radius R has two small cavities of radii r1and r2 as shown in the figure. On heating the disc

View SolutionIf the sum of the areas of two circles with radii R1 and R2 is equal to the area of a circle of radius R, then
View SolutionThe radii of the bases of two right circular solid cones of same height are r1 and r2 respectively. The cones are melted and recast into a solid sphere of radius R . Show that the height of each cone is given by h=4R3r12+r22
View SolutionA solid copper sphere of density ρ, specific heat c and radius r is at temperature T1. It is suspended inside a chamber whose walls are at temperature 0K. What is the time required for the temperature of sphere to drop to T2? Take the emmissivity of the sphere to be equal to e.
View Solution
