If critical angle for TIR from medium to vacuum is 45 deg then velocity of light in medium
1.5x108ms
3sq2√ x 108ms
2√x108ms
3x108ms
The correct Answer is:B
To solve the problem, we need to find the velocity of light in a medium given that the critical angle for total internal reflection (TIR) from the medium to vacuum is 45 degrees.
1. Understanding the Critical Angle:
The critical angle (θ_c) is the angle of incidence above which total internal reflection occurs. The relationship between the critical angle and the refractive index (μ) of the medium is given by:
sin(θc)=1μ
2. Substituting the Given Value:
We know that the critical angle θ_c is 45 degrees. Thus, we can substitute this value into the equation:
sin(45∘)=1μ
3. Calculating the Sine of 45 Degrees:
The sine of 45 degrees is:
sin(45∘)=1√2
Therefore, we can rewrite the equation as:
1√2=1μ
4. Finding the Refractive Index (μ):
Rearranging the equation gives us:
μ=√2
5. Relating Refractive Index to Velocity:
The refractive index (μ) is also related to the speed of light in vacuum (C) and the speed of light in the medium (V) by the formula:
μ=CV
Rearranging this gives:
V=Cμ
6. Substituting the Values:
We know that the speed of light in vacuum (C) is approximately 3×108 m/s. Substituting the value of μ:
V=3×108 m/s√2
7. Final Calculation:
Thus, the speed of light in the medium is:
V=3√2×108 m/s
Final Answer:
The velocity of light in the medium is:
V=3√2×108 m/s
---
The critical angle (θ_c) is the angle of incidence above which total internal reflection occurs. The relationship between the critical angle and the refractive index (μ) of the medium is given by:
sin(θc)=1μ
We know that the critical angle θ_c is 45 degrees. Thus, we can substitute this value into the equation:
sin(45∘)=1μ
The sine of 45 degrees is:
sin(45∘)=1√2
Therefore, we can rewrite the equation as:
1√2=1μ
Rearranging the equation gives us:
μ=√2
The refractive index (μ) is also related to the speed of light in vacuum (C) and the speed of light in the medium (V) by the formula:
μ=CV
Rearranging this gives:
V=Cμ
We know that the speed of light in vacuum (C) is approximately 3×108 m/s. Substituting the value of μ:
V=3×108 m/s√2
Thus, the speed of light in the medium is:
V=3√2×108 m/s
The velocity of light in the medium is:
V=3√2×108 m/s
Similar Questions
Knowledge Check
Critical angle for light going from medium (i) to (ii) is θ . The speed of light in medium (i) is v then speed in medium (ii) is
Av(1−cosθ)BvsinθCvcosθDv(1−sinθ)
The critical angle of light from medium A to medium B is θ. The speed of light in medium A is v. the speed of light in medium B is
View SolutionWave length of light in denser mediumis 4000Å, it is grazing into a rarer medium. If critical angle for the pairof mediam is sin−1(23) then the wave length of light in rarer medium is
View SolutionThe critical angle for light going from medium X into medium Y is θ . The speed of light in medium X is v. The speed of light in medium Y is
View SolutionHow is the refractive index of a material related to:
Velocity of light in vacuum or air and the velocity of light in a given medium?
View SolutionIf c is velocity of light in vacuum and v is the velocity of light in a medium of refractive index μ, then
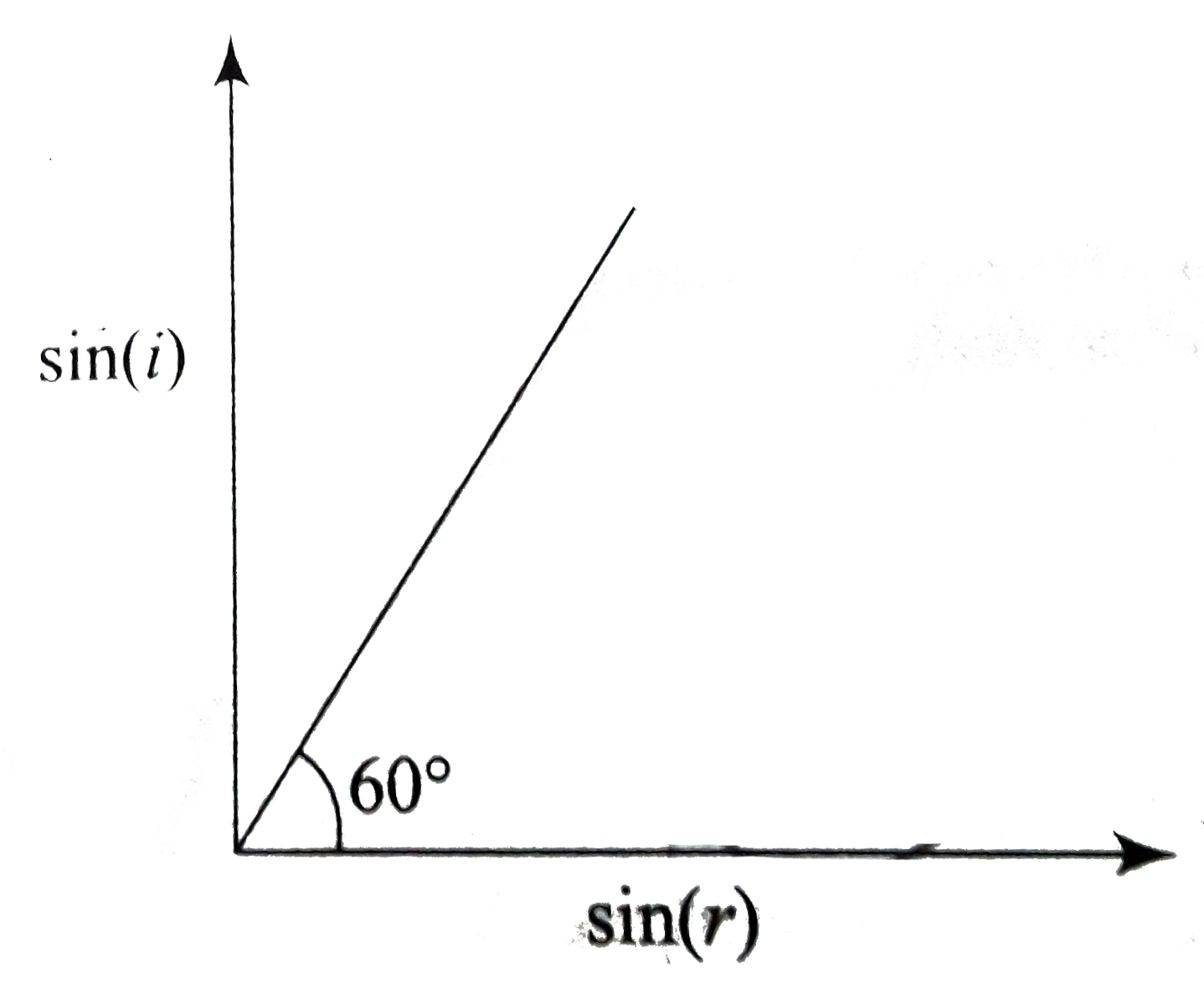
View SolutionA ray of light is incident on a medium with angle of incidence I and refracted into a second medium with angle of refraction r. The graph of sin(i)vssin(R) is as shown in figure. Then the velocity of light in the first medium in n times the velocity of light in the second medium. What shoul be th evalue of n?
View SolutionA ray of light is incident on the surface of seperation of a medium at an angle 45∘ and is refracted in the medium at an angle 30∘. What will be the velocity of light in the medium?
View Solution
