Let OABCD be a pentagon in which the sides OA and CB are parallel and the sides OD and AB are parallel as shown in figure. Also, OA:CB=2:1 and OD:AB=1:3. if the diagonals OC and AD meet at x, find OX:XC.
The correct Answer is:2:5
To solve the problem, we will use the properties of vectors and the given ratios to find the ratio OX:XC.
1. Understanding the Problem:
We have a pentagon OABCD where OA∥CB and OD∥AB. The ratios given are OA:CB=2:1 and OD:AB=1:3. We need to find the ratio OX:XC where X is the intersection of the diagonals OC and AD.
2. Setting Up the Vectors:
Let O be the origin, so we can denote the position vectors of the points as follows:
- →O=→0
- Let →A=→a
- Let →B=→b
- Let →C=→c
- Let →D=→d
3. Using the Given Ratios:
From the ratio OA:CB=2:1, we can express this as:
OA=2kandCB=k
Therefore, we can write:
→A=2→band→C=→b+k
From the ratio OD:AB=1:3:
OD=mandAB=3m
Thus, we can express:
→D=13(→b+2→a)
4. Finding the Position Vectors:
We can express the position vectors of points C and D in terms of →A and →B:
→C=→b+kand→D=13(→b+2→a)
5. Finding the Intersection Point X:
The point X divides OC and AD in some ratios. Let:
OX:XC=λ:1andAX:XD=μ:1
The position vector of X can be expressed in two ways:
→X=λ→Cλ+1and→X=μ→D+→Aμ+1
6. Setting the Equations Equal:
Setting the two expressions for →X equal gives:
λ→Cλ+1=μ→D+→Aμ+1
7. Substituting Known Values:
Substitute the values of →C and →D into the equation and simplify.
8. Solving for λ and μ:
By equating coefficients and solving the resulting equations, we can find the values of λ and μ.
9. Finding the Ratio OX:XC:
Once we have λ, we can find the desired ratio:
OX:XC=λ:1
Final Answer:
After solving, we find that:
OX:XC=2:5
We have a pentagon OABCD where OA∥CB and OD∥AB. The ratios given are OA:CB=2:1 and OD:AB=1:3. We need to find the ratio OX:XC where X is the intersection of the diagonals OC and AD.
Let O be the origin, so we can denote the position vectors of the points as follows:
- →O=→0
- Let →A=→a
- Let →B=→b
- Let →C=→c
- Let →D=→d
From the ratio OA:CB=2:1, we can express this as:
OA=2kandCB=k
Therefore, we can write:
→A=2→band→C=→b+k
OD=mandAB=3m
Thus, we can express:
→D=13(→b+2→a)
We can express the position vectors of points C and D in terms of →A and →B:
→C=→b+kand→D=13(→b+2→a)
The point X divides OC and AD in some ratios. Let:
OX:XC=λ:1andAX:XD=μ:1
→X=λ→Cλ+1and→X=μ→D+→Aμ+1
Setting the two expressions for →X equal gives:
λ→Cλ+1=μ→D+→Aμ+1
Substitute the values of →C and →D into the equation and simplify.
By equating coefficients and solving the resulting equations, we can find the values of λ and μ.
Once we have λ, we can find the desired ratio:
OX:XC=λ:1
After solving, we find that:
OX:XC=2:5
Topper's Solved these Questions
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)9videos
VECTOR ALGEBRA
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - Exercise (Single Integer Answer Type Questions)7videos
VECTOR ALGEBRA
- BOOK - ARIHANT MATHS ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONS AND IDENTITIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)19videos
TRIGONOMETRIC FUNCTIONS AND IDENTITIES
Similar Questions
Let OABCD be a pentagon in which the sides OA and CB are parallel and the sides OD and AB are parallel as shown in figure. Also, OA:CB=2:1 and OD:AB=1:3. if the diagonals OC and AD meet at x, find OX:OC.
View SolutionThe side AB of a square ABCD is parallel to the x-axis. Find the slopes of all its sides.

Also, find :
the slope of the diagonal AC.View SolutionThe side AB of a square ABCD is parallel to the x-axis. Find the slopes of all its sides.

Also, find :
the slope of the diagonal BD.View SolutionTwo sides of a Rhombus ABCD are parallel to the lines x−y=5 and 7x−y=3. The diagonals intersect at (2,1) then the equations of the diagonals are
View SolutionThe sides AB and AD of a parallelogram ABCD are 2x−y+1=0andx+3y−10=0 respectively and C is the point (−1,−2). Find the equation of the diagonals AC.
View SolutionThrough A, B and C lines RQ, PR and QP have been drawn, respectively parallel to sides BC, CA and AB of a ΔABC as shown in figure. Show that BC=12QR.
 View Solution
View SolutionIn the given figure, AB and EC are parallel to each other. Sides AD and BC are 2 cm each and are perpendicular to AB.
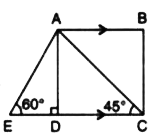
Given that ∠AED=60∘and∠ACD=45∘, calculate :
(i) ABView SolutionIn the given figure, AB and EC are parallel to each other. Sides AD and BC are 2 cm each and are perpendicular to AB.
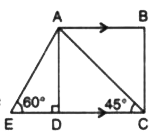
Given that ∠AED=60∘and∠ACD=45∘, calculate :
(iii) AEView SolutionIn the given figure, AB and EC are parallel to each other. Sides AD and BC are 2 cm each and are perpendicular to AB.
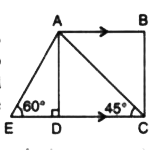
Given that ∠AED=60∘and∠ACD=45∘, calculate :
(ii) ACView Solution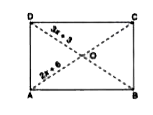
In the given figure, the diagonals AC and BD of a rectangle ABCD intersect each other at point O.
If OA=2x+6 and OD=3x+3, find the value of x.View Solution

