If n∑i=1a2i=λ,∀ai≥0 and if greatest and least values of (n∑i=1ai)2 are λ1 and λ2 respectively, then (λ1−λ2) is
nλ
(n−1)λ
(n+2)λ
(n+1)λ
The correct Answer is:B
To solve the problem, we need to find the difference between the greatest and least values of (n∑i=1ai)2 given that n∑i=1a2i=λ and ai≥0.
1. Understanding the Problem:
We know that n∑i=1a2i=λ. We want to find the maximum and minimum values of (n∑i=1ai)2.
2. Applying Cauchy-Schwarz Inequality:
By the Cauchy-Schwarz inequality, we have:
(n∑i=1ai)2≤nn∑i=1a2i
Substituting n∑i=1a2i=λ, we get:
(n∑i=1ai)2≤nλ
This gives us the upper bound for (n∑i=1ai)2.
3. Finding the Maximum Value:
The maximum value of (n∑i=1ai)2 occurs when all ai are equal. If a1=a2=…=an=k, then:
nk2=λ⟹k2=λn⟹k=√λn
Therefore,
n∑i=1ai=n⋅√λn=√nλ
Thus, the maximum value is:
λ1=nλ
4. Finding the Minimum Value:
The minimum value of (n∑i=1ai)2 occurs when one ai is √λ and the rest are 0. Thus:
n∑i=1ai=√λ
Therefore, the minimum value is:
λ2=λ
5. Calculating the Difference:
Now, we can find the difference:
λ1−λ2=nλ−λ=(n−1)λ
Final Answer:
λ1−λ2=(n−1)λ
We know that n∑i=1a2i=λ. We want to find the maximum and minimum values of (n∑i=1ai)2.
By the Cauchy-Schwarz inequality, we have:
(n∑i=1ai)2≤nn∑i=1a2i
Substituting n∑i=1a2i=λ, we get:
(n∑i=1ai)2≤nλ
This gives us the upper bound for (n∑i=1ai)2.
The maximum value of (n∑i=1ai)2 occurs when all ai are equal. If a1=a2=…=an=k, then:
nk2=λ⟹k2=λn⟹k=√λn
Therefore,
n∑i=1ai=n⋅√λn=√nλ
Thus, the maximum value is:
λ1=nλ
The minimum value of (n∑i=1ai)2 occurs when one ai is √λ and the rest are 0. Thus:
n∑i=1ai=√λ
Therefore, the minimum value is:
λ2=λ
Now, we can find the difference:
λ1−λ2=nλ−λ=(n−1)λ
λ1−λ2=(n−1)λ
Topper's Solved these Questions
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise For Session 1
5 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise For Session 2
11 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
21 Videos
- SETS, RELATIONS AND FUNCTIONS BOOK - ARIHANT MATHS ENGLISHCHAPTER - SETS, RELATIONS AND FUNCTIONS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
12 Videos
Similar Questions
If a1,a2,a3,a4 and a5 are in AP with common difference ≠0, find the value of 5∑i=1ai when a3=2.
View SolutionIf λ1andλ2 are the wavelength of the first members of the Lyman and Paschen series, respectively , then λ1:λ2 is
View SolutionA dye absorbs a photon of wavelength λ and re− emits the same energy into two phorons of wavelengths λ1 and λ2 respectively. The wavelength λ is related with λ1 and λ2 as :
View SolutionShow that if λ1,λ2,....,lamndan are n eigenvalues of a square matrix a of order n, then the eigenvalues of the matric A2 are λ21,λ22,...,λ2n.
View SolutionTwo particle are moving perpendicular to each with de-Broglie wave length λ1 and λ2. If they collide and stick then the de-Broglie wave length of system after collision is : (A) λ=λ1λ2√λ21+λ22 (B) λ=λ1√λ21+λ22 (C) λ=√λ21+λ22λ2 (D) λ=λ1λ2√λ1+λ2
View SolutionFor any n positive numbers a1,a2,…,an such that
n∑i=1ai=α, the least value of n∑i=1a−1i, is
View SolutionLet αandβ be the values of x obtained form the equation λ2(x2−x)+2λx+3=0andifλ1,λ2 be the two values of λ for which αandβ are connected by the relation αβ+βα=43 . then find the value of λ21λ2+λ22λ1andλ21λ22+λ22λ21
View SolutionTransition between three energy energy levels in a particular atom give rise to three Spectral line of wevelength , in increasing magnitudes. λ1,λ2 and λ3. Which one of the following equations correctly ralates λ1,λ2 and λ3?
λ1=λ2−λ3
λ1=λ3−λ2
1λ1=1λ2+1λ3
1λ2=1λ3+1λ1
View SolutionIn the figure shown a sinusoidal wave is generated at the end A the wave travels along positive x-axis and during its motion it encounter another string BD at the junction 'B'atx=0.The density of string AB and BC are ρand9ρrespectively and their radii of cross sections are 2randr. The wave function amplitude and wavelength of incident wave are respectively yiAiandλi Similarly for reflected and transmitted wave these peremeters are yr,Ar,λrandyt,At,λt
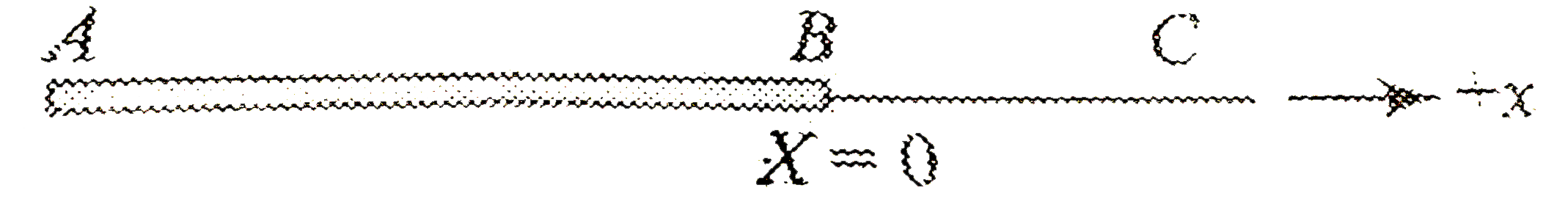
The ratio of wavelengths λrtoλi(i.e.λr:λt) will be
View SolutionTwo sound waves of wavelengths λ1 and λ2(λ2>λ1) produces nbeats/s , the speed of sound is
View Solution