A, B, C are respectively the points (1,2), (4, 2), (4, 5). If are the points of trisection of the line segment BC, the area of the Triangle is
1
2
The correct Answer is:B
To find the area of triangle AT1T2 where A,B,C are the points (1,2),(4,2),(4,5) and T1,T2 are the points of trisection of line segment BC, we will follow these steps:
Step 1: Identify Points
The points are given as:
- A(1,2)
- B(4,2)
- C(4,5)
Step 2: Find Points of Trisection T1 and T2
To find the points of trisection T1 and T2 of the line segment BC:
- The coordinates of B are (4,2) and C are (4,5).
- The distance between B and C is 5−2=3.
- Since T1 and T2 trisect the segment, they divide it into three equal parts of 1 unit each.
Thus:
- T1 is at (4,2+1)=(4,3)
- T2 is at (4,2+2)=(4,4)
Step 3: Calculate the Area of Triangle AT1T2
The area of a triangle given vertices (x1,y1),(x2,y2),(x3,y3) can be calculated using the formula:
Area=12|x1(y2−y3)+x2(y3−y1)+x3(y1−y2)|
Substituting the coordinates:
- A(1,2)
- T1(4,3)
- T2(4,4)
The area becomes:
Area=12|1(3−4)+4(4−2)+4(2−3)|
Calculating each term:
=12|1(−1)+4(2)+4(−1)|
=12|−1+8−4|
=12|3|=32
Step 4: Final Area Calculation
Thus, the area of triangle AT1T2 is 32 square units.
Summary
The area of triangle AT1T2 is 32 square units.
The points are given as:
- A(1,2)
- B(4,2)
- C(4,5)
To find the points of trisection T1 and T2 of the line segment BC:
- The coordinates of B are (4,2) and C are (4,5).
- The distance between B and C is 5−2=3.
- Since T1 and T2 trisect the segment, they divide it into three equal parts of 1 unit each.
- T1 is at (4,2+1)=(4,3)
- T2 is at (4,2+2)=(4,4)
The area of a triangle given vertices (x1,y1),(x2,y2),(x3,y3) can be calculated using the formula:
Area=12|x1(y2−y3)+x2(y3−y1)+x3(y1−y2)|
- A(1,2)
- T1(4,3)
- T2(4,4)
Area=12|1(3−4)+4(4−2)+4(2−3)|
Calculating each term:
=12|1(−1)+4(2)+4(−1)|
=12|−1+8−4|
=12|3|=32
Thus, the area of triangle AT1T2 is 32 square units.
The area of triangle AT1T2 is 32 square units.
Topper's Solved these Questions
- COORDINATE SYSTEM AND COORDINATES Book:ARIHANT MATHS ENGLISHChapter:COORDINATE SYSTEM AND COORDINATES Exercise:Exercise (More Than One Correct Option Type Questions)
Explore 7 Videos
- COORDINATE SYSTEM AND COORDINATES Book:ARIHANT MATHS ENGLISHChapter:COORDINATE SYSTEM AND COORDINATES Exercise:Exercise (Passage Based Questions)
Explore 5 Videos
- COORDINATE SYSTEM AND COORDINATES Book:ARIHANT MATHS ENGLISHChapter:COORDINATE SYSTEM AND COORDINATES Exercise:Exercise For Session 4
Explore 17 Videos
- CONTINUITY AND DIFFERENTIABILITYBook:ARIHANT MATHS ENGLISHChapter:CONTINUITY AND DIFFERENTIABILITYExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 20 Videos
- DEFINITE INTEGRALBook:ARIHANT MATHS ENGLISHChapter:DEFINITE INTEGRALExercise:Exercise (Questions Asked In Previous 13 Years Exam)
Explore 38 Videos
Similar Questions
The point of intersection of the tangents at t1 and t2 to the parabola y2=12x is
View SolutionThe reflection of the point (t−1,2t+2) in a line is (2t+1,t), then the equation of the line has slope equals to
View SolutionShow that P (3,m -5) is a point of trisection of the line segment joining the points A (4,-2) and B (1, 4). Hence, find the value of 'm'.
View Solutionlet the point B be the reflection of the point A(2,3) with respect to the line 8x-6y-23=0. let TA and TB be circles of radii 2 and 1 with centres A and B respectively. Let T be a common tangent to the circles TA and TB such that both the circles are on the same side of T. if C is the point of intersection of T and the line passing through A and B then the length of the line segment AC is
View Solution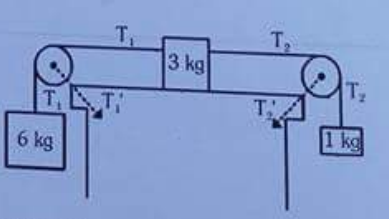
Calculate a, T1,T2,T1'&T2'.
View Solutiont1,t2,t3 are lengths of tangents drawn from a point (h,k) to the circles x2+y2=4,x2+y2−4x=0andx2+y2−4y=0 respectively further, t41=t22 t23+16. Locus of the point (h,k) consist of a straight line L1 and a circle C1 passing through origin. A circle C2 , which is equal to circle C1 is drawn touching the line L1 and the circle C1 externally.
Equation of C1 is
View Solutiont1,t2,t3 are lengths of tangents drawn from a point (h,k) to the circles x2+y2=4,x2+y2−4=0andx2+y2−4y=0 respectively further, t41=t22 t23+16. Locus of the point (h,k) consist of a straight line L1 and a circle C1 passing through origin. A circle C2 , which is equal to circle C1 is drawn touching the line L1 and the circle C1 externally.
Equation of L1 is
View SolutionABCD is a square with side AB = 2. A point P moves such that its distance from A equals its distance from the line BD. The locus of P meets the line AC at T1 and the line through A parallel to BD at T2 and T3. The area of the triangle T1T2T3 is :
View SolutionABCD is a square with side AB = 2. A point P moves such that its distance from A equals its distance from the line BD. The locus of P meets the line AC at T1 and the line through A parallel to BD at T2 and T3. The area of the triangle T1T2T3 is :
View SolutionThe points (at21,2at1),(at22,2at2)and(a,0) will be collinear, if
View Solution
