A right circular cone with radius R and height H contains a liquid which evaporates at a rate proportional to its surface area in contact with air (proportionality constant =k>0). Find the time after which the cone will be empty.
Step by step video solution for A right circular cone with radius R and height H contains a liquid which evaporates at a rate proportional to its surface area in contact with air (proportionality constant =k gt 0). Find the time after which the cone will be empty. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- DIFFERENTIAL EQUATION BOOK - ARIHANT MATHS ENGLISHCHAPTER - DIFFERENTIAL EQUATION EXERCISE - Solved Examples
1 Videos
- DIFFERENTIAL EQUATION BOOK - ARIHANT MATHS ENGLISHCHAPTER - DIFFERENTIAL EQUATION EXERCISE - Subjective Type Question
1 Videos
- DETERMINANTS BOOK - ARIHANT MATHS ENGLISHCHAPTER - DETERMINANTS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
18 Videos
- DIFFERENTIATION BOOK - ARIHANT MATHS ENGLISHCHAPTER - DIFFERENTIATION EXERCISE - Exercise For Session 10
4 Videos
Similar Questions
A right circular cone with radius R and height H contains a liquid which evaporates at a rate proportional to its surface area in contact with air (proportionality constant k is positive). Suppose that r(t) is the radius of the liquid cone at time t. The time after which the cone is empty is
View SolutionAn inverted cone of height H, and radius R is pointed at bottom. It is completely filled with a volatile liquid. If the rate of evaporation is directly proportional to the surface area of the liquid in contact with air (constant of proportionality k > 0). Find the time in which whole liquid evaporates.
View Solution
Knowledge Check
If a right circular cone has a lateral surface area of 6π and a slant height of 6, what is the radius of the base ?
A0.50B0.75C1.00D1.25
Spherical rain drop evaporates at a rate proportional to its surface area. The differential equation corresponding to the rate of change of the radius of the rain drop if the constant of proportionality is K>0 is
View SolutionFind the total surface area of a right circular cone with radius 6cm and height 8cm.
View Solution
A right circular cone, with radius and height 8 and 6 respectively , is cut parallel at the middle of the height to get a smaller cone and a frustum. By what percentage, to the nearest integer, is the combined total surface area of the smaller cone less than of the frustum?
View SolutionThe radius and slant height of a cone are in the ratio of 4:7. If its curved surface area is 792cm2, find its radius. (Useπ=227)
View SolutionA cone of radius R and height H, is hanging inside a liquid of density ρ by means of a string as shown in figure. The force due to the liquid acting on the slant surface of the cone is
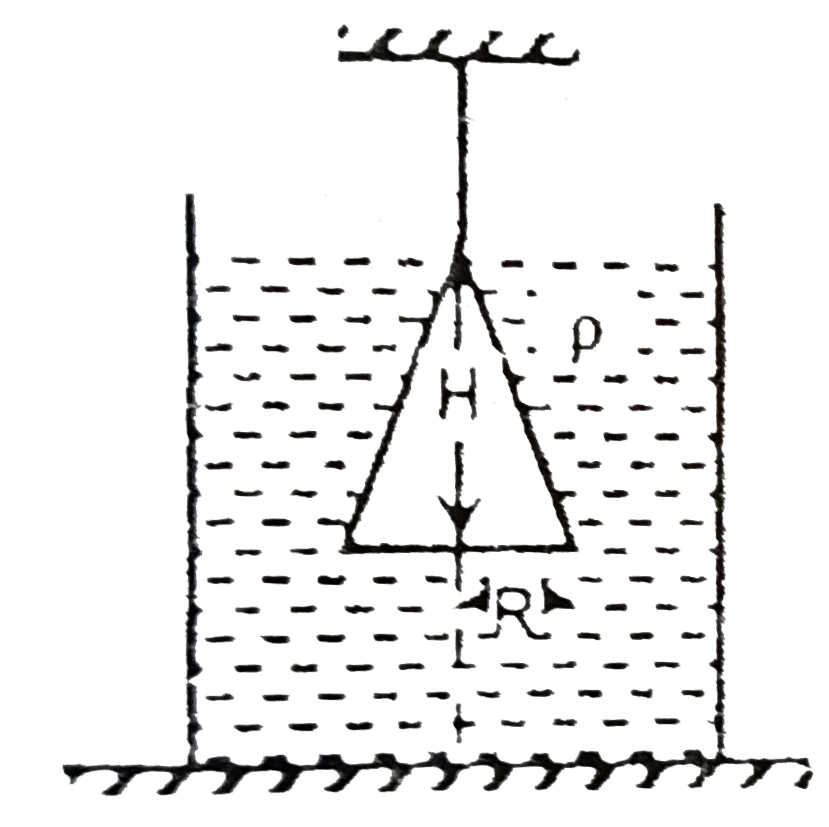
View SolutionThe surface area of a spherical balloon being inflated, changes at a rate proportional to time t. If initially its radius is 1 unit and after 3 secons it is 2 units, find the radius after t seconds.
View SolutionA right circular cone is 3.6 cm high and radius of its base is 1.6 cm. It is melted and recast into a right circular cone with radius of its base as 1.2 cm. Find its height.
View Solution
