Prove that a2+b2+c2+2abc<2, where a,b,c are the sides of triangle ABC such that a+b+c=2.
To prove that a2+b2+c2+2abc<2 where a,b,c are the sides of triangle ABC such that a+b+c=2, we can follow these steps:
Step 1: Express a,b,c in terms of new variables
Given a+b+c=2, we can define new variables:
x=1−a,y=1−b,z=1−c
This implies:
x+y+z=1
Step 2: Rewrite a,b,c
From the definitions of x,y,z, we can express a,b,c as:
a=1−x,b=1−y,c=1−z
Step 3: Substitute into the expression
Now substitute a,b,c into the left-hand side of the inequality:
LHS=(1−x)2+(1−y)2+(1−z)2+2(1−x)(1−y)(1−z)
Step 4: Expand the squares
Expanding the squares:
(1−x)2=1−2x+x2,(1−y)2=1−2y+y2,(1−z)2=1−2z+z2
Thus,
LHS=(1−2x+x2)+(1−2y+y2)+(1−2z+z2)+2(1−x)(1−y)(1−z)
Combining the constant terms:
LHS=3−2(x+y+z)+(x2+y2+z2)+2(1−x−y+xy−xz−yz)
Step 5: Simplify the expression
Since x+y+z=1:
LHS=3−2(1)+(x2+y2+z2)+2(1−1+xy+xz+yz)
This simplifies to:
LHS=1+(x2+y2+z2)+2(xy+xz+yz)
Step 6: Use the identity for squares
Using the identity x2+y2+z2=(x+y+z)2−2(xy+xz+yz):
x2+y2+z2=1−2(xy+xz+yz)
Substituting this back into the expression gives:
LHS=1+(1−2(xy+xz+yz))+2(xy+xz+yz)
This simplifies to:
LHS=2+0=2−2xyz
Step 7: Conclude the proof
Since xyz is non-negative and less than or equal to 1 (as x,y,z are all between 0 and 1), we have:
LHS<2
Thus, we conclude that:
a2+b2+c2+2abc<2
Given a+b+c=2, we can define new variables:
x=1−a,y=1−b,z=1−c
This implies:
x+y+z=1
From the definitions of x,y,z, we can express a,b,c as:
a=1−x,b=1−y,c=1−z
Now substitute a,b,c into the left-hand side of the inequality:
LHS=(1−x)2+(1−y)2+(1−z)2+2(1−x)(1−y)(1−z)
Expanding the squares:
(1−x)2=1−2x+x2,(1−y)2=1−2y+y2,(1−z)2=1−2z+z2
Thus,
LHS=(1−2x+x2)+(1−2y+y2)+(1−2z+z2)+2(1−x)(1−y)(1−z)
Combining the constant terms:
LHS=3−2(x+y+z)+(x2+y2+z2)+2(1−x−y+xy−xz−yz)
Since x+y+z=1:
LHS=3−2(1)+(x2+y2+z2)+2(1−1+xy+xz+yz)
This simplifies to:
LHS=1+(x2+y2+z2)+2(xy+xz+yz)
Using the identity x2+y2+z2=(x+y+z)2−2(xy+xz+yz):
x2+y2+z2=1−2(xy+xz+yz)
Substituting this back into the expression gives:
LHS=1+(1−2(xy+xz+yz))+2(xy+xz+yz)
This simplifies to:
LHS=2+0=2−2xyz
Since xyz is non-negative and less than or equal to 1 (as x,y,z are all between 0 and 1), we have:
LHS<2
Thus, we conclude that:
a2+b2+c2+2abc<2
Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
21 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Subjective Type Questions)
21 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHS ENGLISHCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
51 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHS ENGLISHCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
An equilateral triangle PQR is circumscribed about a given ΔABC. Prove that the maximum area of ΔPQR is 2Δ+a2+b2+c22√3). Where a,b,c are the sides of ΔABCanΔ is its area.
View SolutionLet P be the point inside that ΔABC. Such that ∠APB=∠BPC=∠CPA. Prove that
PA+PB+PC=√a2+b2+c22+2√3Δ,wherea,b,cΔ are the sides and the area of ΔABC.
View SolutionProve that b2c2+c2a2+a2b2>abc(a+b+c), where a,b,c>0 .
View SolutionIf sides of triangle ABC are a, b, and c such that 2b=a+c, then
View SolutionLet a,b,c be the sides of a triangle ABC, a=2c,cos(A-C)+cos B=1. then the value of C is
View SolutionIf angle C of triangle ABC is 900, then prove that tanA+tanB=c2ab (where, a,b,c, are sides opposite to angles A,B,C, respectively).
View SolutionIf a,b and c are sides of a ritht triangle where c is the hypotenuse, prove that the radius of the circle which touches the sides of the triangle is r=a+b−c2.
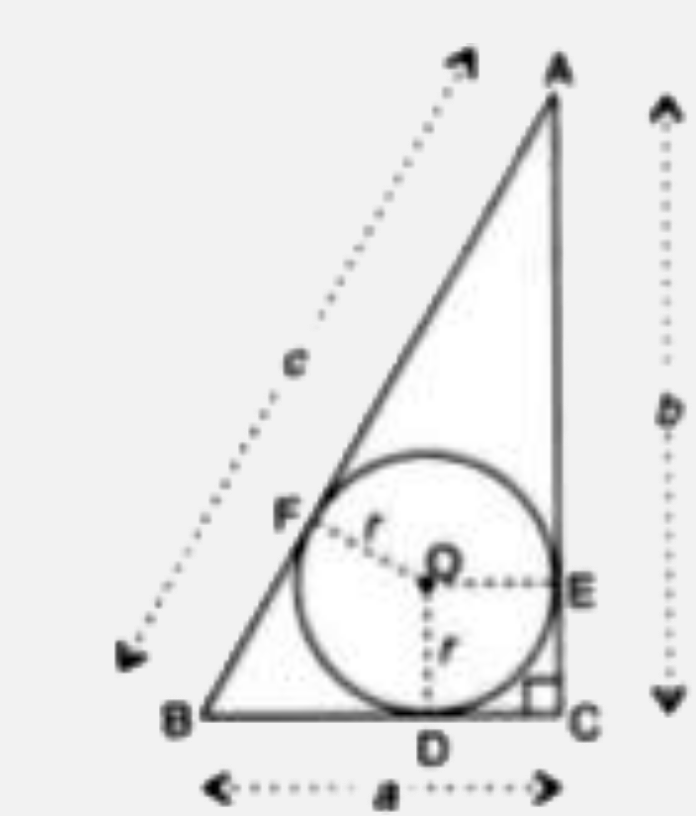
View SolutionIf cosθ=ab+c,cosϕ=ba+c and cosψ=ca+b where θ,ϕ,ψ∈(0,π) and a,b,c are sides of triangle ABC then tan2(θ2)+tan2(ϕ2)+tan2(ψ2)=
View SolutionProve that the area of an equilateral triangle is equal to √34a2, where a is the side of the triangle. GIVEN : An equilateral triangle ABC such that AB=BC=CA=a. TO PROVE : ar(△ABC)=√34a2. CONSTRUCTION : Draw AD⊥BC.
View SolutionIf in a triangle ABC,bc2cosA=b2+c2−2bccosA then prove that the triangle must be isosceles.
View Solution
