The largest area of a rectangle which has one side on the x-axis and the two vertices on the curve y=e−x2 is
√2e−1/2
2e−1/2
e−1/2
None of these
The correct Answer is:A
To find the largest area of a rectangle that has one side on the x-axis and two vertices on the curve y=e−x2, we can follow these steps:
Step 1: Define the Area of the Rectangle
Let the rectangle have vertices at (a,0) and (−a,0) on the x-axis, and the top vertices at (a,e−a2) and (−a,e−a2) on the curve. The width of the rectangle is 2a and the height is e−a2.
The area A of the rectangle can be expressed as:
A=width×height=2a⋅e−a2
Step 2: Differentiate the Area Function
To find the maximum area, we need to differentiate A with respect to a and set the derivative equal to zero.
The area function is:
A(a)=2ae−a2
Using the product rule for differentiation:
dAda=2e−a2+2a⋅dda(e−a2)
Using the chain rule, we have:
dda(e−a2)=e−a2⋅(−2a)
Thus,
dAda=2e−a2−4a2e−a2
Factoring out 2e−a2:
dAda=2e−a2(1−2a2)
Step 3: Set the Derivative Equal to Zero
Setting the derivative equal to zero to find critical points:
2e−a2(1−2a2)=0
Since e−a2 is never zero, we can set:
1−2a2=0
Solving for a2:
2a2=1⟹a2=12⟹a=1√2 (taking the positive root)
Step 4: Calculate the Maximum Area
Now we substitute a=1√2 back into the area formula:
A=2ae−a2=2⋅1√2⋅e−(1√2)2
Calculating e−(1√2)2=e−12:
A=2⋅1√2⋅e−12=√2e−12
Final Answer
The largest area of the rectangle is:
A=√2e−12
---
Let the rectangle have vertices at (a,0) and (−a,0) on the x-axis, and the top vertices at (a,e−a2) and (−a,e−a2) on the curve. The width of the rectangle is 2a and the height is e−a2.
A=width×height=2a⋅e−a2
To find the maximum area, we need to differentiate A with respect to a and set the derivative equal to zero.
A(a)=2ae−a2
dAda=2e−a2+2a⋅dda(e−a2)
Using the chain rule, we have:
dda(e−a2)=e−a2⋅(−2a)
Thus,
dAda=2e−a2−4a2e−a2
Factoring out 2e−a2:
dAda=2e−a2(1−2a2)
Setting the derivative equal to zero to find critical points:
2e−a2(1−2a2)=0
Since e−a2 is never zero, we can set:
1−2a2=0
Solving for a2:
2a2=1⟹a2=12⟹a=1√2 (taking the positive root)
Now we substitute a=1√2 back into the area formula:
A=2ae−a2=2⋅1√2⋅e−(1√2)2
Calculating e−(1√2)2=e−12:
A=2⋅1√2⋅e−12=√2e−12
The largest area of the rectangle is:
A=√2e−12
Topper's Solved these Questions
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHS ENGLISHCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - Exercise For Session 5
8 Videos
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHS ENGLISHCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - Exercise (Single Option Correct Type Questions)
27 Videos
- MONOTONICITY MAXIMA AND MINIMABOOK - ARIHANT MATHS ENGLISHCHAPTER - MONOTONICITY MAXIMA AND MINIMAEXERCISE - Exercise For Session 3
5 Videos
- MATRICES BOOK - ARIHANT MATHS ENGLISHCHAPTER - MATRICES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
49 Videos
- PAIR OF STRAIGHT LINES BOOK - ARIHANT MATHS ENGLISHCHAPTER - PAIR OF STRAIGHT LINES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
2 Videos
Similar Questions
Find the area of the largest rectangle with lower base on the x-axis and upper vertices on the curve y=12−x2.
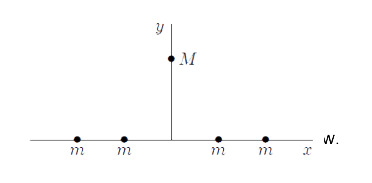
View SolutionFour particles, each with mass m are arranged symmetrically about the origin on the x axis. A fifth particle, with mass M, is on the y axis. The direction of the gravitational force on M is:
View SolutionFind the area of the largest rectangle in the first quadrant with two sides on x-axis and y-axis and one vertex on the curve y=12−x2
View SolutionThe area bounded by the curve y=4−x2 and X-axis is
View SolutionThe area bounded by the X-axis and the curve y=4x−x2−3 is
View SolutionThe area contained between the x-axis and one area of the curve y=cos3x, is
View SolutionTwo vertices of a rectangle are on the positive x-axis. The other two vertices lie on the lines y=4xandy=−5x+6. Then the maximum area of the rectangle is: 43 (b) 35 (c) 45 (d) 34
View SolutionThe area bounded by the x-axis and the curve y=4x−x2−3 is
View SolutionFind the area bounded by the curve |y|+12≤e−|x|.
View SolutionThe area bounded by y=ex, Y-axis and the line y = e is
View Solution
