Relative permittivity of water is 81. If and are permitivities of water and vacuum respectively, then :
To find the relation between the permittivity of water (e_w) and the permittivity of vacuum (e_0), we can use the concept of relative permittivity. Here’s a step-by-step solution:
Step 1: Understand the Concept of Relative Permittivity
Relative permittivity (also known as the dielectric constant) is defined as the ratio of the permittivity of a substance to the permittivity of vacuum.
Step 2: Write the Formula for Relative Permittivity
The formula for relative permittivity (ε_r) is given by:
ϵr=ϵwϵ0
where:
- ε_r is the relative permittivity,
- ε_w is the permittivity of water,
- ε_0 is the permittivity of vacuum.
Step 3: Substitute the Given Value
From the problem, we know that the relative permittivity of water (ε_r) is 81. Therefore, we can write:
81=ϵwϵ0
Step 4: Rearrange the Equation
To find the relationship between ε_w and ε_0, we can rearrange the equation:
ϵw=81⋅ϵ0
Step 5: Conclusion
Thus, the relationship between the permittivity of water and the permittivity of vacuum is:
ϵw=81ϵ0
Final Answer
The answer is that the permittivity of water (ε_w) is 81 times the permittivity of vacuum (ε_0).
---
Relative permittivity (also known as the dielectric constant) is defined as the ratio of the permittivity of a substance to the permittivity of vacuum.
The formula for relative permittivity (ε_r) is given by:
ϵr=ϵwϵ0
where:
- ε_r is the relative permittivity,
- ε_w is the permittivity of water,
- ε_0 is the permittivity of vacuum.
From the problem, we know that the relative permittivity of water (ε_r) is 81. Therefore, we can write:
81=ϵwϵ0
To find the relationship between ε_w and ε_0, we can rearrange the equation:
ϵw=81⋅ϵ0
Thus, the relationship between the permittivity of water and the permittivity of vacuum is:
ϵw=81ϵ0
The answer is that the permittivity of water (ε_w) is 81 times the permittivity of vacuum (ε_0).
Similar Questions
An EM wave from air enters a medium. The electric fields are
→E1=E01ˆxcos[2πv(zc−t)] in air and
→E=E02ˆxcos[k(2z−ct)] in medium, where the wave number k and frequency v refer to their values in air. The medium is non-magnetic. If ∈r1 and ∈r2 refer to relative permittivities of air and medium respectively, which of the following options is correct ?
View SolutionIf relative permittivity and relative permeability of a medium are 3 and 43 respectively. the critical angle for this medium is.
View SolutionIf relative permittivity and relative permeability of a medium are 3 and 43 respectively. the critical angle for this medium is.
View SolutionIf the relative permitivity and relative permeability of a medium are 2 and 1.28 respectively, then refractive index of that material is:
View SolutionRelative permitivity and permeability of a material εrandμr, respectively . Which of the following values of these quantities are allowed for a diamagnetic material?
View SolutionLet [ε0] denote the dimensional formula of the permittivity of the vacuum, and [μ0] that of the permeability of the vacuum. If M = mass ,L = length, T = time and I = electric current,
View SolutionLet [ε0] denote the dimensional formula of the permittivity of the vacuum, and [μ0] that of the permeability of the vacuum. If M=mass,L=≤n>h,T=timeandI=e≤ctriccurrent,
View SolutionLet [ε0] denote the dimensional formula of the permittivity of the vacuum, and [μ0] that of the permeability of the vacuum. If M=mass,L=≤n>h,T=timeandI=e≤ctriccurrent,
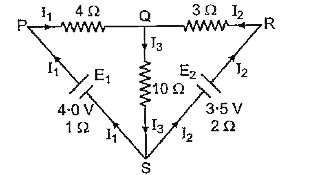
View SolutionIn the circuit shown in figure below, E1 and E2 are batteries having emfs 4.0 V and 3.5 V respectively and internal resistance 1Ω and 2Ω respectively. Using Kirchhoff.s laws, calculate currents : I1,I2 and I3.
View SolutionWhat is the relation between the refractive index of water with respect to air (aμw) and the refractive index of air with respect to water (wμa).
View Solution
