If relative permittivity and relative permeability of a medium are 3 and 43 respectively. the critical angle for this medium is.
30∘
60∘
45∘
15∘
The correct Answer is:1
Step by step video & image solution for If relative permittivity and relative permeability of a medium are 3 and 4/3 respectively. the critical angle for this medium is. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
The refractive index and the permeability of a medium are respectively 1.5 and 5×10−7Hm−1. The relative permitivity of the medium is nearly
A25B15C10D6
What is the relative permeability of air ?
View SolutionDo permeability and relative permeability have the same dimensions?
View SolutionWavelength of given light waves in air and in a medium are 6000 Å and 4000 Å respectively . The critical angle for the medium is given by
View SolutionWavelength of given light waves in air and in a medium are 6000 Å and 3000 Å, respectively. The critical angle is
View SolutionRelative permitivity and permeability of a material εrandμr, respectively . Which of the following values of these quantities are allowed for a diamagnetic material?
View SolutionIf ε0 and μ0 are respectively the electric permittivity and the magnetic permeability of free space and ε and μ the corresponding quantities in a medium, the refractive index of the medium is
View SolutionIn figure, the reflected ray B makes an angle 90∘ with the ray C. If I,r1andr2 are the angles of incidence, reflection and refraction, respectively. Then, the critical angle of the medium is
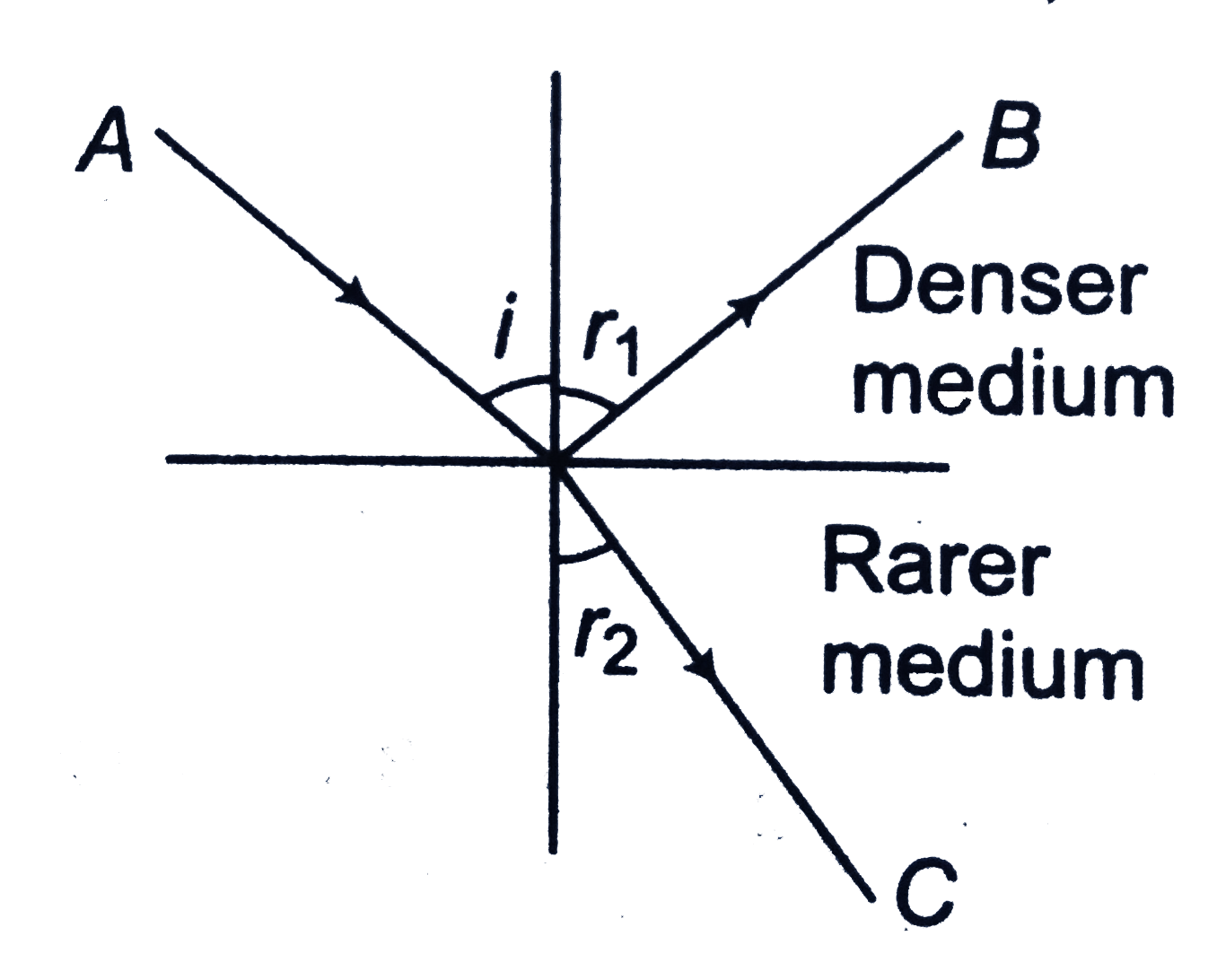 View Solution
View Solution
