A highly rigid cubical block A of mass M and side L is fixed rigidly on the another cubical block of same dimensions and of modulus of rigidity ρ such that the lower face of A completely covers the upper face of B. The lower face of B is rigidly held on a horizontal surface. A small force F is applied perpendicular to one of the side faces of A. After the force is withdrawn, block A executes small oscillations, the time period of which is given by
2π√MρL
2π√(Mρ/L)
2π√(ML/ρ)
2π√(M/ρL)
The correct Answer is:d
To solve the problem, we need to determine the time period of the oscillations of block A after the force F is withdrawn. The key parameters involved are the mass of the block (M), the side length of the block (L), and the modulus of rigidity (ρ).
1. Identify the System:
- We have two cubical blocks, A and B, both with mass M and side length L. Block A is fixed on top of block B, which is held rigidly on a horizontal surface.
2. Force Application:
- A small force F is applied perpendicular to one of the side faces of block A. This force causes a displacement in block A.
3. Restoring Force:
- Once the force F is removed, block A will experience a restoring force due to the deformation of block B. The restoring force is proportional to the displacement and is governed by the modulus of rigidity (ρ).
4. Determine the Effective Spring Constant (k):
- The effective spring constant k can be expressed in terms of the modulus of rigidity (ρ) and the dimensions of the blocks. The relationship can be derived from the definition of shear modulus:
k=ρAL
- Here, A is the area of the face of block A that is in contact with block B, which is L2. Thus, we can write:
k=ρL2L=ρL
5. Using the Formula for Time Period (T):
- The time period T of oscillation for a mass-spring system is given by:
T=2π√mk
- Substituting our expression for k:
T=2π√MρL
6. Final Expression for Time Period:
- Therefore, the time period of oscillation of block A is:
T=2π√MρL
Conclusion:
The time period of the oscillations of block A after the force is withdrawn is given by:
T=2π√MρL
- We have two cubical blocks, A and B, both with mass M and side length L. Block A is fixed on top of block B, which is held rigidly on a horizontal surface.
- A small force F is applied perpendicular to one of the side faces of block A. This force causes a displacement in block A.
- Once the force F is removed, block A will experience a restoring force due to the deformation of block B. The restoring force is proportional to the displacement and is governed by the modulus of rigidity (ρ).
- The effective spring constant k can be expressed in terms of the modulus of rigidity (ρ) and the dimensions of the blocks. The relationship can be derived from the definition of shear modulus:
k=ρAL
- Here, A is the area of the face of block A that is in contact with block B, which is L2. Thus, we can write:
k=ρL2L=ρL
- The time period T of oscillation for a mass-spring system is given by:
T=2π√mk
- Substituting our expression for k:
T=2π√MρL
- Therefore, the time period of oscillation of block A is:
T=2π√MρL
The time period of the oscillations of block A after the force is withdrawn is given by:
T=2π√MρL
Topper's Solved these Questions
- BOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - VECTORS AND SCALARS [Selected from Previoius Years Engg. & Med. & IIT Exam Qns]35videos
COMPETITION CARE UNIT
- BOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - Dynamics (UNIFORMLY ACCELERATED MOTION)58videos
COMPETITION CARE UNIT
- BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)24videos
CIRCULAR MOTION
- BOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)9videos
DIMENSIONS
Similar Questions
A highly rigid cubical block A of small mass M and side L is fixed rigidly on the other cubical block of same dimensions and of modulus of rigidity η such that the lower face of A completely covers the upper face of B. The lower face of B is rigidly held on a horizontal surface . A small force F is applied perpendicular to one of the side faces of A. After the force is withdrawn , block A executes faces of A. After the force is withdrawn , block A exceutes small oscillations , the time period of which is given by
View SolutionA force Fis tangentially applied on the upper face of a cube of side b and whose lower face is fixed. If modulus of rigidity is n then upper surface of cube is shifted to
View SolutionOne of the square faces of a metal slab of side 50 cm and thickness 20 cm is rigidly fixed on a horizontal surface. If a tangential force of 30 N is applied to the top face and it is known that the shear modulus of the material is 4×1010N/m2, then the displacement (in m) of the top face is
View SolutionA cubical block of side 10 cm floats at the interface of an oil and water. The pressure above that of atmosphere at the lower face of the block is
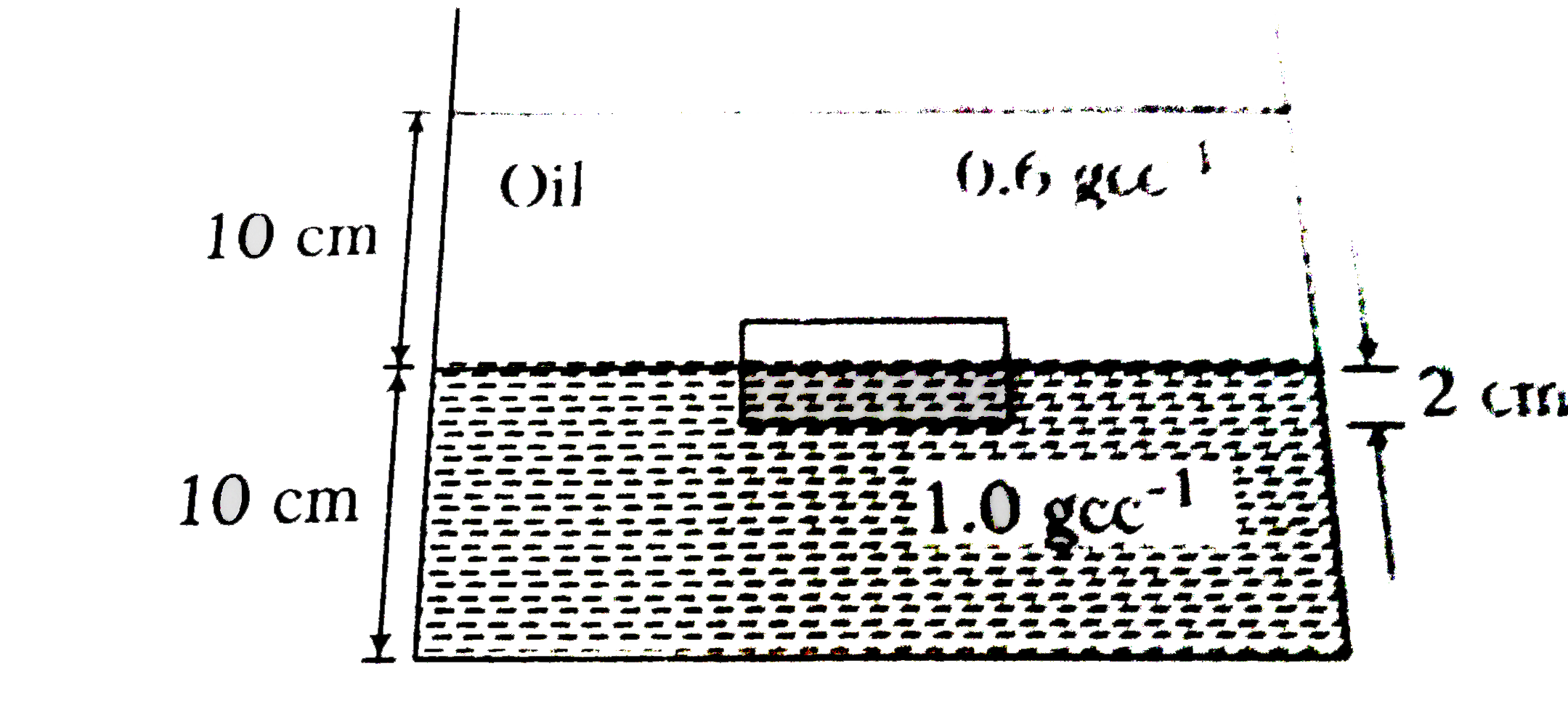 View Solution
View SolutionA cubical block of side 10cm floats at the interface of an oil and water as shown in the figure. The density of oil is 0.6gcm−3 and the lower face of ice cube is 2cm below the interface. The pressure above that of the atomosphere at the lower face of the block is
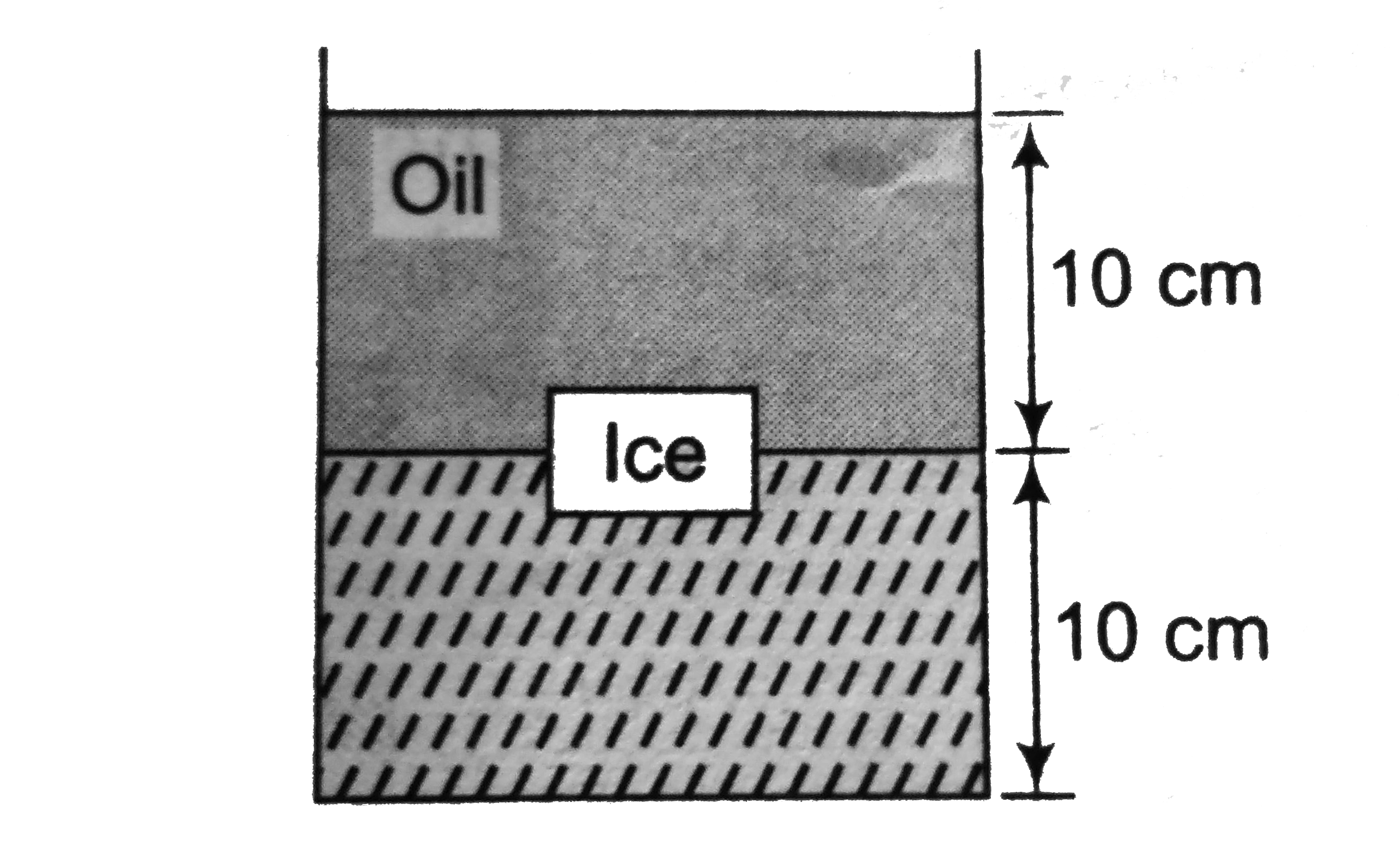 View Solution
View SolutionA block of mass m is kept over another block of mass 2m. Coefficient of friction between two blocks is μ and that of between lower blood from the horizontal surface is zero. A horizontal force F=43μmg is applied on the block of mass 2m. Acceleration of lower block is
View SolutionA small block B of mass m2 is placed on another block A of mass m1 A constant horizontal force F is applied to the block A. All the surfaces are assumed to be frictionless. Find the acceleration of center of mass of the system
View SolutionA block B of mass 0.6kg slides down the smooth face PR of a wedge A of mass 1.7kg which can move freely on a smooth horizontal surface.
The inclination of the face PR to the horizontal is 45∘ . Then :
 View Solution
View SolutionA uniform cube of side a and mass m rests on a rough horizontal table. A horizontal force F is applied normal to one of the faces at a point directly above the centre of the face, at a height 3a4 above the base. What is the minimum value of F for which the cube begins to tip about an edge?
View SolutionA block of massm is placed on another block of mass M which itself is lying on a horizontal surface .The coefficient of friction between two blocks is μ1and that between the block of mass M and horizontal surfece is μ2 What maximum horizontal force can be applied to the lower block move without separation?
 View Solution
View Solution

