A particle 'P' is moving in a circle of radius 'a' with a uniform speed 'u' 'C' is the centre of the circle and AB is a diameter. The angular velocity of P about A and C are in the ratio
1:1
1:2
2:1
4:1
The correct Answer is:b
To solve the problem, we need to find the ratio of the angular velocities of a particle P moving in a circle about two points: point A (one end of the diameter) and point C (the center of the circle).
1. Understand the Setup:
- The particle P is moving in a circle of radius a with a uniform speed u.
- Point C is the center of the circle, and A and B are the endpoints of the diameter.
2. Define Angular Velocity:
- Angular velocity ω is defined as the rate of change of angular displacement with respect to time.
- For point A, angular velocity ωA=dθ1dt.
- For point C, angular velocity ωC=dθ2dt.
3. Relate Angles:
- The angle θ2 at the center C is related to the angle θ1 at point A by the relationship:
θ2=2θ1
- This is due to the fact that the angle subtended at the center of the circle is twice the angle subtended at any point on the circumference.
4. Differentiate the Relationship:
- Taking the derivative of both sides with respect to time gives:
dθ2dt=2dθ1dt
- This implies:
ωC=2ωA
5. Find the Ratio of Angular Velocities:
- From the relationship ωC=2ωA, we can express the ratio of the angular velocities:
ωCωA=2
- Therefore, the ratio of angular velocities ωC:ωA=2:1.
Final Answer:
The ratio of the angular velocities of particle P about points A and C is 2:1.
- The particle P is moving in a circle of radius a with a uniform speed u.
- Point C is the center of the circle, and A and B are the endpoints of the diameter.
- Angular velocity ω is defined as the rate of change of angular displacement with respect to time.
- For point A, angular velocity ωA=dθ1dt.
- For point C, angular velocity ωC=dθ2dt.
- The angle θ2 at the center C is related to the angle θ1 at point A by the relationship:
θ2=2θ1
- This is due to the fact that the angle subtended at the center of the circle is twice the angle subtended at any point on the circumference.
- Taking the derivative of both sides with respect to time gives:
dθ2dt=2dθ1dt
- This implies:
ωC=2ωA
- From the relationship ωC=2ωA, we can express the ratio of the angular velocities:
ωCωA=2
- Therefore, the ratio of angular velocities ωC:ωA=2:1.
The ratio of the angular velocities of particle P about points A and C is 2:1.
Topper's Solved these Questions
- BOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - UNIFORM CIRCULAR MOTION (ROTATIONAL MOTION AND MOMENT OF INERTIA )25videos
COMPETITION CARE UNIT
- BOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - GRAVITATION25videos
COMPETITION CARE UNIT
- BOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - MOTION IN FLUIDS25videos
COMPETITION CARE UNIT
- BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)24videos
CIRCULAR MOTION
- BOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)9videos
DIMENSIONS
Similar Questions
A particle P is moving in a circle of radius 'a' with a uniform speed v. C is the centre of the circle and AB is a diameter. When passing through B the angular velocity of P about A and C are in the ratio
View SolutionThe acceleration of an object moving in a circle of radius R with uniform speed v is
View Solution
Knowledge Check
- Question 1 - Select One
A particle is moving in a circle with uniform speed. IT has constant
AvelocityBAccelerationCkinetic energyDdisplacement
When a particle moves in a circle with a uniform speed
View SolutionA particle is moving in a circle with uniform speed its motion is
View SolutionA particle is moving in a circle of radius R with constant speed. The time period of the particle is T. In a time t=T6
Average velocity of the particle is…..View SolutionA article moves in a circle of radius 20 cm with linear speed of 10 m/s. Find the angular velocity
View SolutionA particle is rotating in circle with uniform speed as shown. The angular momentum of the particle w.r.t. origin is :-
 View Solution
View SolutionA particle is moving in a circle of radius r with speed v as shown in the figure. The magnitude of change in velocity in moving from P to Q is
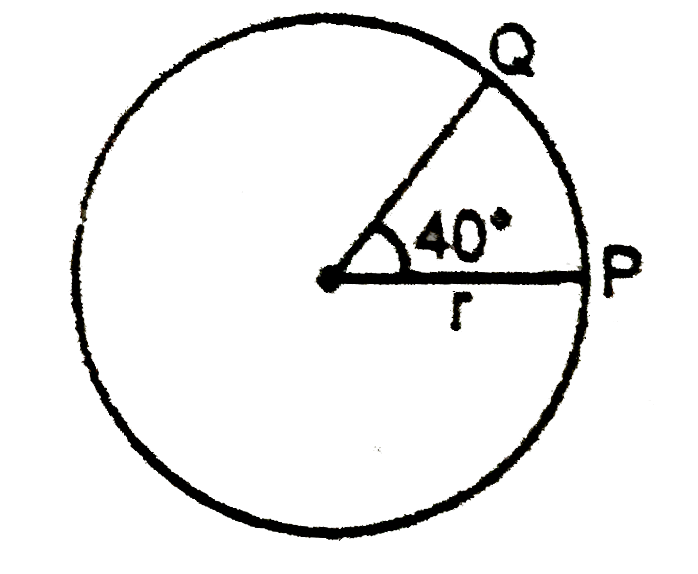 View Solution
View SolutionA particle is moving in a circle of radius r with speed v as shown in the figure. The magnitude of change in velocity in moving from P to Q is
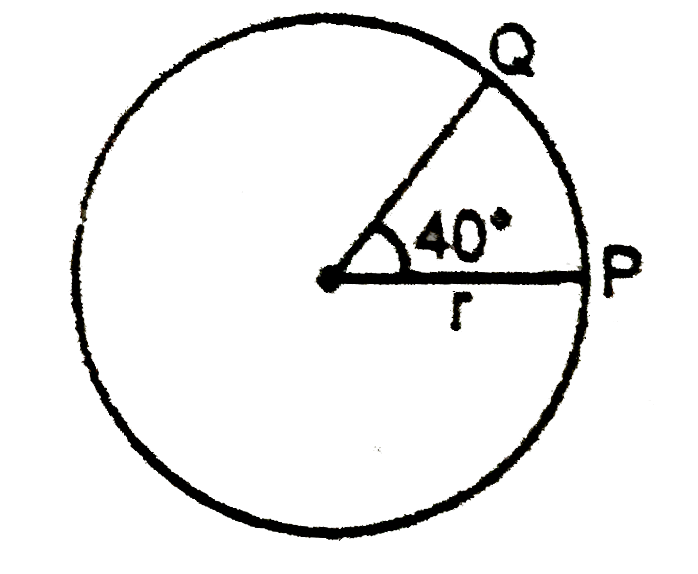 View Solution
View Solution

