A particle starts with uniform acceleration. Draw a graph taking the displacement(s) of the particle along y-axis and time(t) along x-axis. What is the curve known as?
To solve the problem of drawing a graph of displacement versus time for a particle starting with uniform acceleration, we can follow these steps:
Step 1: Understand the Motion
A particle starting with uniform acceleration means that its initial velocity (u) is zero, and it accelerates at a constant rate (a). The second equation of motion relates displacement (s), initial velocity (u), acceleration (a), and time (t):
s=ut+12at2
Step 2: Substitute Initial Conditions
Since the particle starts from rest, we have:
- Initial velocity, u=0
- The equation simplifies to:
s=12at2
Step 3: Identify the Variables for the Graph
- Displacement (s) will be plotted on the y-axis.
- Time (t) will be plotted on the x-axis.
Step 4: Determine the Shape of the Graph
The equation s=12at2 indicates that displacement is proportional to the square of time. This means that as time increases, displacement increases with the square of time.
Step 5: Draw the Graph
1. Choose a value for acceleration a (for example, a=1 m/s² for simplicity).
2. Calculate displacement for various time intervals:
- For t=0: s=0
- For t=1: s=12×1×(1)2=0.5
- For t=2: s=12×1×(2)2=2
- For t=3: s=12×1×(3)2=4.5
- For t=4: s=12×1×(4)2=8
3. Plot these points on the graph:
- (0, 0)
- (1, 0.5)
- (2, 2)
- (3, 4.5)
- (4, 8)
4. Connect the points with a smooth curve.
Step 6: Identify the Curve
The resulting graph will be a parabola opening upwards, indicating that the displacement increases quadratically with time.
Conclusion
The curve is known as a parabola.
---
A particle starting with uniform acceleration means that its initial velocity (u) is zero, and it accelerates at a constant rate (a). The second equation of motion relates displacement (s), initial velocity (u), acceleration (a), and time (t):
s=ut+12at2
Since the particle starts from rest, we have:
- Initial velocity, u=0
- The equation simplifies to:
s=12at2
- Displacement (s) will be plotted on the y-axis.
- Time (t) will be plotted on the x-axis.
The equation s=12at2 indicates that displacement is proportional to the square of time. This means that as time increases, displacement increases with the square of time.
1. Choose a value for acceleration a (for example, a=1 m/s² for simplicity).
2. Calculate displacement for various time intervals:
- For t=0: s=0
- For t=1: s=12×1×(1)2=0.5
- For t=2: s=12×1×(2)2=2
- For t=3: s=12×1×(3)2=4.5
- For t=4: s=12×1×(4)2=8
- (0, 0)
- (1, 0.5)
- (2, 2)
- (3, 4.5)
- (4, 8)
The resulting graph will be a parabola opening upwards, indicating that the displacement increases quadratically with time.
The curve is known as a parabola.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Solved Examples9videos
BASIC MATHEMATICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.12videos
BASIC MATHEMATICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 2 VOLUME 6EXERCISE - Integer4videos
ARCHIVES 2 VOLUME 6
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example13videos
CALORIMETRY
Similar Questions
Figure shows the displacement time graph of a particle moving on the X-axis
 View Solution
View SolutionFigure shows the displacement-time graph of a particle moving on the X-axis
 View Solution
View SolutionDraw the acceleration-time graphs for the given velocity-time graphs of a particle moving along x-axis.
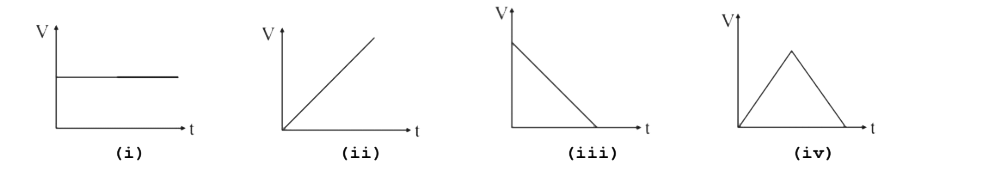 View Solution
View SolutionThe graph between displacement and time for a particle moving with uniform acceleration is a
View SolutionA particle starts with some initial velocity with an acceleration along the direction of motion. Draw a graph depicting the variation of velocity (v) along y-axis with the variation of displacement(s) along x-axis.
View SolutionIf a graph is plotted by taking spectral emissive power along y-axis and wavelength along X-axis then the area below the graph above wavelength axis is
View SolutionA particle moves along the X-axis as x=u(t−2s)+a(t−2s)2.
View SolutionThe displacement x of a particle moving along x-axis at time t is given by x2=2t2+6t. The velocity at any time t is
View SolutionFigures (i) and (ii) below show the displacement - time graphs of two particles moving along the x-axis. We can say that
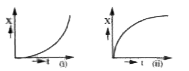 View Solution
View SolutionFor a particle moving along the x-axis, mark the correct statement(s).
View Solution

