If a graph is plotted by taking spectral emissive power along y-axis and wavelength along X-axis then the area below the graph above wavelength axis is
A. Emissivity
B. Total intensity of radiation
C. Diffusivity
D. Solar constant
To solve the question, we need to analyze the relationship between spectral emissive power and wavelength, and understand what the area under the curve represents.
1. Understanding Spectral Emissive Power:
- Spectral emissive power (E(λ, T)) is defined as the power emitted per unit area per unit wavelength by a black body at a given temperature T. It varies with both wavelength (λ) and temperature (T).
2. Graph Representation:
- In the given graph, the y-axis represents the spectral emissive power, while the x-axis represents the wavelength. The area under the curve in this graph corresponds to the total emissive power emitted by the body across all wavelengths.
3. Planck's Law:
- According to Planck's law, the spectral emissive power is given by:
E(λ,T)=c1λ5⋅1ec2λT−1
- Here, c1 and c2 are constants, and this equation describes how emissive power varies with wavelength at a specific temperature.
4. Area Under the Curve:
- The area under the spectral emissive power curve (above the wavelength axis) represents the total power emitted per unit area by the black body across all wavelengths. This is often referred to as the total emissive power (E).
5. Relation to Total Emissive Power:
- According to the Stefan-Boltzmann law, the total emissive power (E) is related to the temperature (T) of the black body:
E=σT4
- Here, σ is the Stefan-Boltzmann constant. The total emissive power is the integral of the spectral emissive power over all wavelengths.
6. Conclusion:
- Therefore, the area below the graph (above the wavelength axis) represents the total emissive power of the body, which is a measure of the total energy emitted per unit area.
Final Answer:
The area below the graph above the wavelength axis is the total emissive power of the body.
- Spectral emissive power (E(λ, T)) is defined as the power emitted per unit area per unit wavelength by a black body at a given temperature T. It varies with both wavelength (λ) and temperature (T).
- In the given graph, the y-axis represents the spectral emissive power, while the x-axis represents the wavelength. The area under the curve in this graph corresponds to the total emissive power emitted by the body across all wavelengths.
- According to Planck's law, the spectral emissive power is given by:
E(λ,T)=c1λ5⋅1ec2λT−1
- Here, c1 and c2 are constants, and this equation describes how emissive power varies with wavelength at a specific temperature.
- The area under the spectral emissive power curve (above the wavelength axis) represents the total power emitted per unit area by the black body across all wavelengths. This is often referred to as the total emissive power (E).
- According to the Stefan-Boltzmann law, the total emissive power (E) is related to the temperature (T) of the black body:
E=σT4
- Here, σ is the Stefan-Boltzmann constant. The total emissive power is the integral of the spectral emissive power over all wavelengths.
- Therefore, the area below the graph (above the wavelength axis) represents the total emissive power of the body, which is a measure of the total energy emitted per unit area.
The area below the graph above the wavelength axis is the total emissive power of the body.
Similar Questions
A graph is plotted by taking pressure along y-axis and centigrade temperature along X-axis for an ideal gas at constant volume. x intercept of the graph is
View SolutionA graph is plotted between PVm along Y-axis and P along X-axis whre Vm is the molar volume of a real gas Find the intercept along Y-axis .
View SolutionA graph is plotted between PVm along y-axis and P along x-axis, where Vm is the molar volume of a real gas. Find the intercept .
View SolutionA graph is plotted with PV/T on y-axis and mass of the gas along x-axis for different gases. The graph is
View SolutionA particle starts with uniform acceleration. Draw a graph taking the displacement(s) of the particle along y-axis and time(t) along x-axis. What is the curve known as?
View SolutionA graph is drawn with 1u along x-axis and 1v along the y-axis. If the intercept on the x-axis is 0.5m−1, the focal length of the mirror is (in meter).
View SolutionFor a particle moving along x−axis, speed must be increasing for the following graph :
View SolutionA graph is plotted for gas 'C' , by putting its weight (in gm) on X-axis and the Volume (L) of gas at NTP on Y-axis. Select the correct statement :
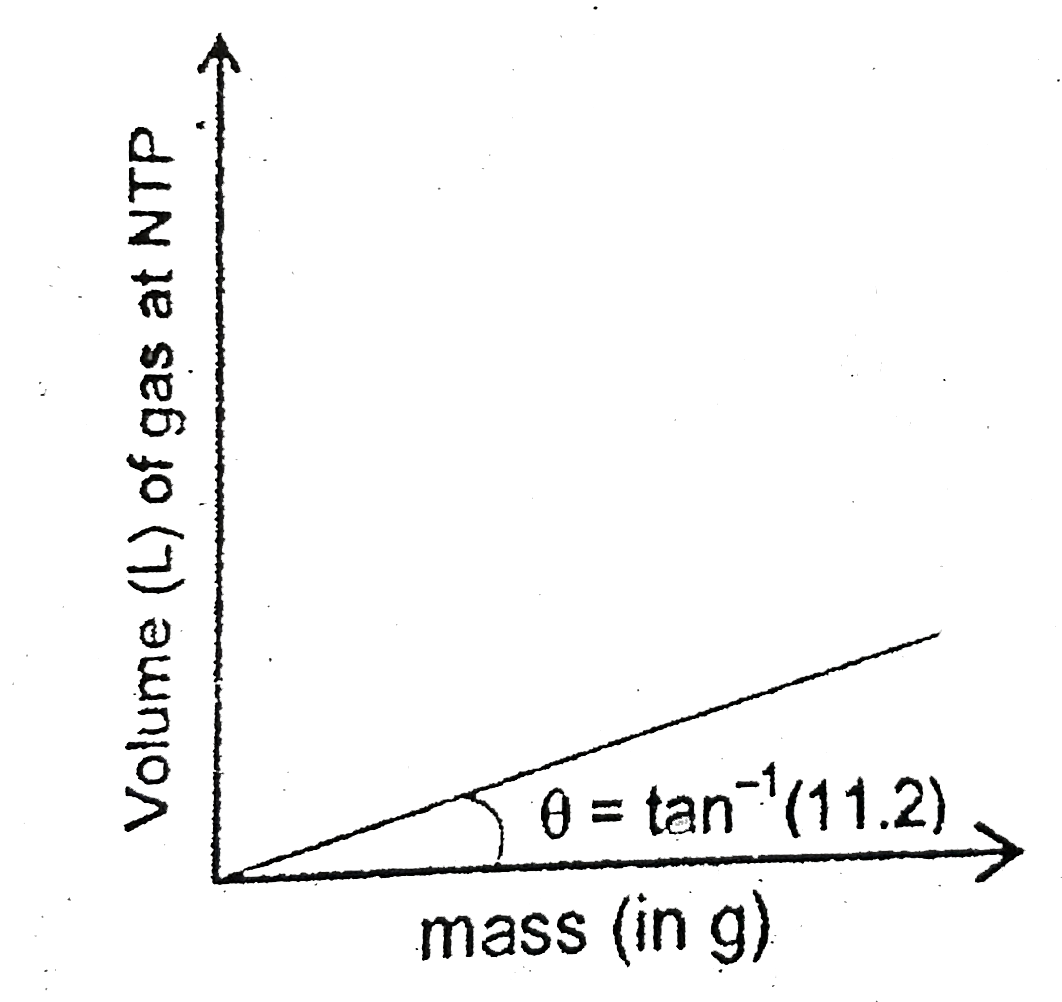
View SolutionAn electron is projected along positive z-axis, magnetic field is present along y-axis then direction of initial acceleration of the particle is
View SolutionWe plot the graph having temperature in ∘C on x - axis and in ∘F on y - axis. If the graph is straight line, then the correct statement is
View Solution