Forces X,Y and Z have magnitudes 10N5(√3−1)N and 5(√3+1)N, respectively. The forces Y and Z act in the same direction as shown in (figure) The resultant of X and Y and the resultant of X and Z have the same magnitudes. Find θ, the angle between X and Y.

The correct Answer is:150∘
Resultant of →X+→Y=→X+→Z
(10)2+(5√3−1)2)+2.10.(5√3−1)cosθ
=(10)2+(5√3+1)2+2.10.(5√3+1)cosθ
or (5√3−1)2−(5√3+1)2=2.10cosθ[(5√3+1)−(5√3−1)]
(2.5.√3)(−2)−2.10cosθ[2]=−510√3=cosθ
−√32=cosθ⇒θ=150∘
Topper's Solved these Questions
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Single Correct
51 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise 3.2
13 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Integer
9 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (integer)
4 Videos
Similar Questions
The two forces 2√2N and xN are acting at a point their resultant is perpendicular to ˆxN and having magnitude of →6N. The angle between the two forces and magnitude of x are
View SolutionTwo horizontal forces of magnitudes of 10N and PN act on a particle. The force of magnitude 10N acts due west and the force of magnitude PN acts on a bearing of 30∘ east of north as shown in (figure) The resultant of these two force acts due north. Find the magnitude of the resultant.
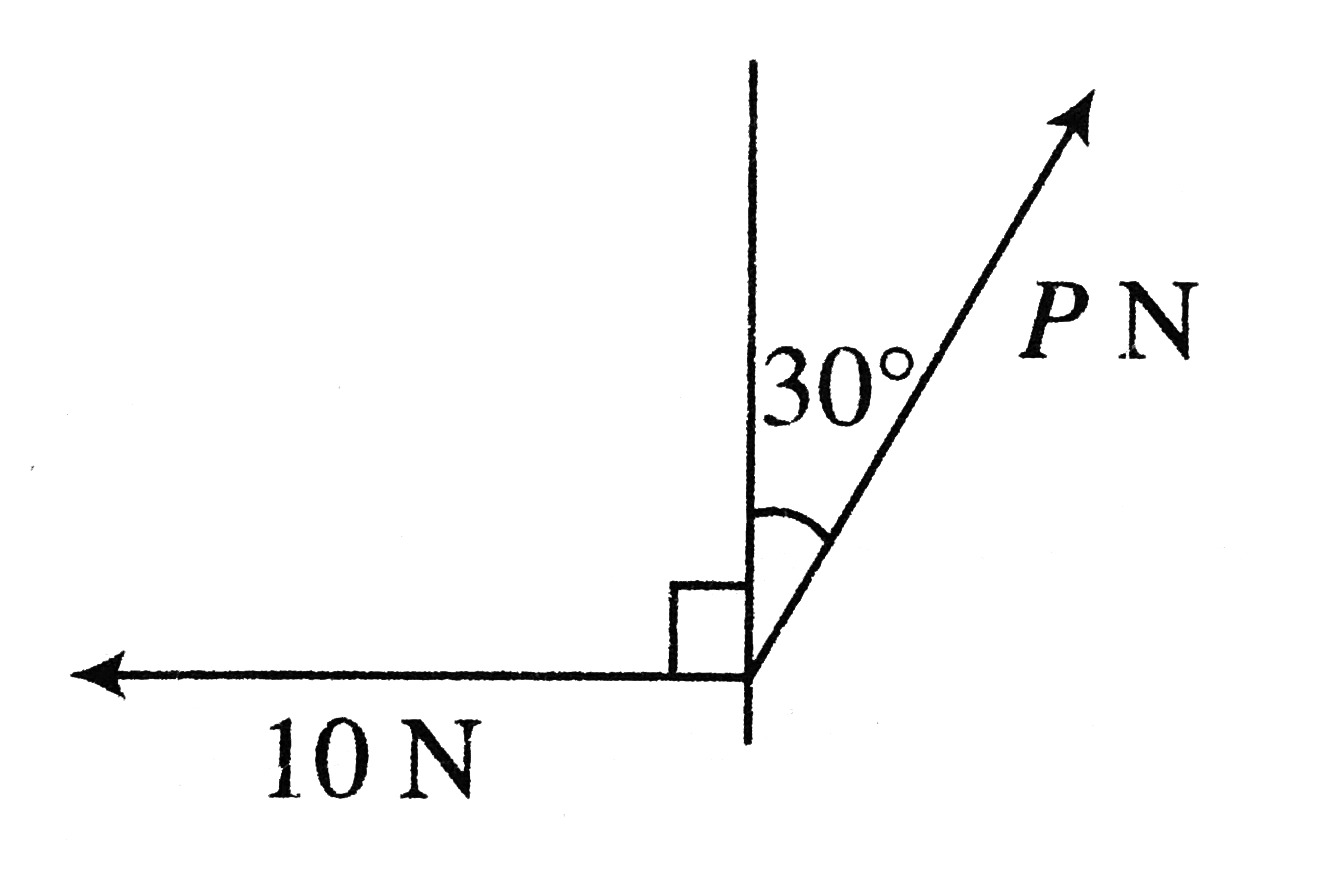
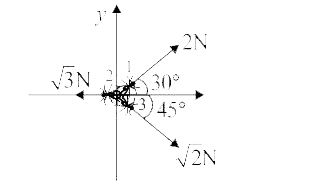
View SolutionThree ants 1, 2 and 3 are pulling a grain with forces of magnitudes 2N√3N and √2N and as shown in the figure. Find the resultant force acting on the grain.
View SolutionFind the angle between the planes x+y+2z=9adn2x−y+z=15.
View SolutionFind the angle between the plane: x−y+z=5 and x+2y+z=9
View SolutionFind the angle between the plane: 2x−3y+4z=1 and−x+y=4.
View SolutionA uniform plank is supporeted by two equal 120 N forces at x and y as shown in figure. If the support at x is moved to z, then the supporting forces at y and z are
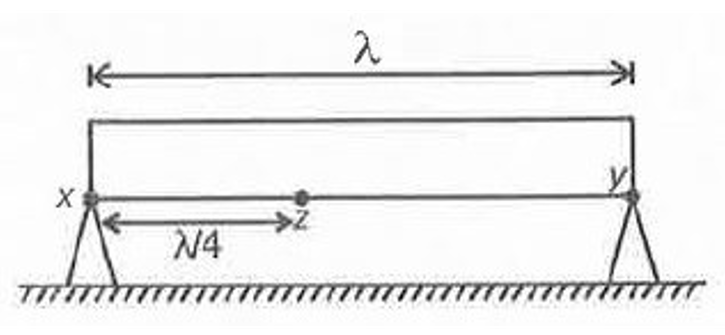
View SolutionFind the angle between the plane: 2x−y+z=4 and x+y+2z=3
View SolutionFind the angle between the planes 3x+y+2z=1 and 2x−y+z+3=0.
View Solutionthere are two force each having same magnitude 10 N .one is incilined at an angle of 30∘ and other at an angle of 135∘ to the positive direction of x-axis .The x and y componenents of the resultant are
View Solution
