Three boys are pulling a heavy trolled by means of three ropes. The boy in the middle is exerting a pull of 100N. The other two boys ,whose ropes both make an angle 30∘ with the central rope, are pulling with forces of 50√3N and 100√3N. Find the magnitudes of the resultant pull on the trolltey.
The correct Answer is:327.9
To solve the problem of finding the resultant pull on the trolley by the three boys, we will break down the forces into their components and then combine them to find the resultant.
Step 1: Identify the Forces
- The middle boy exerts a force of Fm=100N in the positive x-direction.
- The left boy exerts a force of Fl=50√3N at an angle of 30∘ to the left of the middle boy.
- The right boy exerts a force of Fr=100√3N at an angle of 30∘ to the right of the middle boy.
Step 2: Break the Forces into Components
We will break down the forces exerted by the left and right boys into their x and y components.
Left Boy's Force:
- Flx=Flcos(30∘)=50√3⋅√32=75N (to the left, which we consider negative)
- Fly=Flsin(30∘)=50√3⋅12=25√3N (upwards)
Right Boy's Force:
- Frx=Frcos(30∘)=100√3⋅√32=150N (to the right, which we consider positive)
- Fry=Frsin(30∘)=100√3⋅12=50√3N (upwards)
Step 3: Calculate the Total Components
Now, we will sum up the x and y components of all the forces.
Total x-component:
FRx=Fm+Flx+Frx=100+(−75)+150=175N
Total y-component:
FRy=Fly+Fry=25√3+50√3=75√3N
Step 4: Calculate the Magnitude of the Resultant Force
The magnitude of the resultant force R can be calculated using the Pythagorean theorem:
R=√(FRx)2+(FRy)2=√(175)2+(75√3)2
Calculating (75√3)2:
(75√3)2=752⋅3=5625⋅3=16875
Now substituting back:
R=√30625+16875=√47500
Calculating the square root:
R≈218.2N
Final Result
The magnitude of the resultant pull on the trolley is approximately 218.2N.
---
- The middle boy exerts a force of Fm=100N in the positive x-direction.
- The left boy exerts a force of Fl=50√3N at an angle of 30∘ to the left of the middle boy.
- The right boy exerts a force of Fr=100√3N at an angle of 30∘ to the right of the middle boy.
We will break down the forces exerted by the left and right boys into their x and y components.
- Flx=Flcos(30∘)=50√3⋅√32=75N (to the left, which we consider negative)
- Fly=Flsin(30∘)=50√3⋅12=25√3N (upwards)
- Frx=Frcos(30∘)=100√3⋅√32=150N (to the right, which we consider positive)
- Fry=Frsin(30∘)=100√3⋅12=50√3N (upwards)
Now, we will sum up the x and y components of all the forces.
FRx=Fm+Flx+Frx=100+(−75)+150=175N
FRy=Fly+Fry=25√3+50√3=75√3N
The magnitude of the resultant force R can be calculated using the Pythagorean theorem:
R=√(FRx)2+(FRy)2=√(175)2+(75√3)2
Calculating (75√3)2:
(75√3)2=752⋅3=5625⋅3=16875
Now substituting back:
R=√30625+16875=√47500
Calculating the square root:
R≈218.2N
The magnitude of the resultant pull on the trolley is approximately 218.2N.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Single Correct51videos
VECTORS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct5videos
VECTORS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise 3.213videos
VECTORS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Integer9videos
TRAVELLING WAVES
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (integer)4videos
WORK, POWER & ENERGY
Similar Questions
Three ants 1, 2 and 3 are pulling a grain with forces of magnitudes 2N√3N and √2N and as shown in the figure. Find the resultant force acting on the grain.
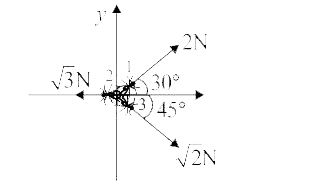 View Solution
View SolutionTwo equal forces are acting at a point with an angle 60∘ between them. If the resultant force is equal to 4√3N, the magnitude of each force is
View Solution
Knowledge Check
- Question 1 - Select One
A rope is wound round a hollow cylinder of mass 3kgandradius40cm. If the rope is pulled with a force of 30N, what is the angualr acceleration of the cylinder ?
A15rads−2B20rads−2C25rads−2D30rads−2
Two equal forces are acting at a point with an angle of 60∘ between them. If the resultant force is equal to 40√3N, The magnitude of each force is :-
View SolutionThe resultant of two forces has magnitude 20N. One of the forces is of magnitude 20√3N and makes an angle of 30∘ with the resultant. Then what is the magnitude of the other force?
View SolutionTwo forces of magnitudes 3N and 4 N act together on an object making an angle 60∘ with each other. Find the resultant force acting on the object.
View SolutionA rope AB of length 5 m and mass 2kg is atteched to a 6kg block kept on a smooth surface ad is pulled by a force of 40N.

Find the force applied by the rope on the blockView SolutionThree boys are pushing horizontally a box placed on horizontal table. One is pushing towards north with a 5√3N force. The second is pushing towards east and third pushes with a force 10 N such that the box is in equilibrium. Find the magnitude of the force, second by is applying in newton.
View SolutionA body of mass √3 kg is suspended by a string to a rigid support. The body is pulled horizontally by a force F until the string makes an angle of 30° with the vertical. The value of F and tension in the string are.
View SolutionTwo forces whose magnitudes are in the ratio 3:5 give a resultant of 28N. If the angle of their inclination is 60∘, find the magnitude of each force.
View Solution

