The friction of the air causes a vertical retardation equal to 10% of the acceleration due to gravity (takeg=10ms−2) The maximum height will be decreased by:
To solve the problem, we need to determine how the maximum height of a projectile is affected by the air friction, which causes a vertical retardation equal to 10% of the acceleration due to gravity.
1. Understanding the Problem:
- The maximum height h of a projectile without air resistance is given by the formula:
h=u2sin2θ2g
- Here, u is the initial velocity, θ is the angle of projection, and g is the acceleration due to gravity.
2. Calculating the Effect of Air Friction:
- The air friction causes a vertical retardation of 10% of g. Thus, the retardation due to air friction is:
Retardation=0.1g
- Therefore, the effective acceleration due to gravity when considering air friction becomes:
g′=g+0.1g=1.1g
3. Finding the New Maximum Height:
- The new maximum height h′ with the effective gravity g′ is given by:
h′=u2sin2θ2g′
- Substituting g′:
h′=u2sin2θ2(1.1g)=u2sin2θ2g⋅11.1=h1.1
4. Finding the Decrease in Maximum Height:
- The decrease in maximum height can be calculated as:
Δh=h−h′=h−h1.1=h(1−11.1)
- Simplifying this:
Δh=h(1.1−11.1)=h(0.11.1)=h11
5. Calculating the Percentage Decrease:
- The percentage decrease in maximum height is given by:
Percentage Decrease=(Δhh)×100=(h/11h)×100=10011≈9.09%
Final Answer:
The maximum height will decrease by approximately 9%.
---
- The maximum height h of a projectile without air resistance is given by the formula:
h=u2sin2θ2g
- Here, u is the initial velocity, θ is the angle of projection, and g is the acceleration due to gravity.
- The air friction causes a vertical retardation of 10% of g. Thus, the retardation due to air friction is:
Retardation=0.1g
- Therefore, the effective acceleration due to gravity when considering air friction becomes:
g′=g+0.1g=1.1g
- The new maximum height h′ with the effective gravity g′ is given by:
h′=u2sin2θ2g′
- Substituting g′:
h′=u2sin2θ2(1.1g)=u2sin2θ2g⋅11.1=h1.1
- The decrease in maximum height can be calculated as:
Δh=h−h′=h−h1.1=h(1−11.1)
- Simplifying this:
Δh=h(1.1−11.1)=h(0.11.1)=h11
- The percentage decrease in maximum height is given by:
Percentage Decrease=(Δhh)×100=(h/11h)×100=10011≈9.09%
The maximum height will decrease by approximately 9%.
Topper's Solved these Questions
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.2
25 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise 5.3
12 Videos
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Solved Examples
7 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
Similar Questions
Knowledge Check
If the friction of air causes a vertical retardation equal to 10% of the acceleration due to gravity then the maximum height and time to reach the maximum height will be decreased by (g=10ms−2)
A10%, 10%B9%, 10%C9%, 9%D10%, 9%
If retardation produced by air resistances to projectile is one-tenth of acceleration due to gravity, the time to reach maximum height approximately-
View SolutionA rocket is fired upwards such that its engine takes 10 s to explods fully. Its velocity- time graph is as shown in the figure. Acceleration due to gravity is 10m/s2.
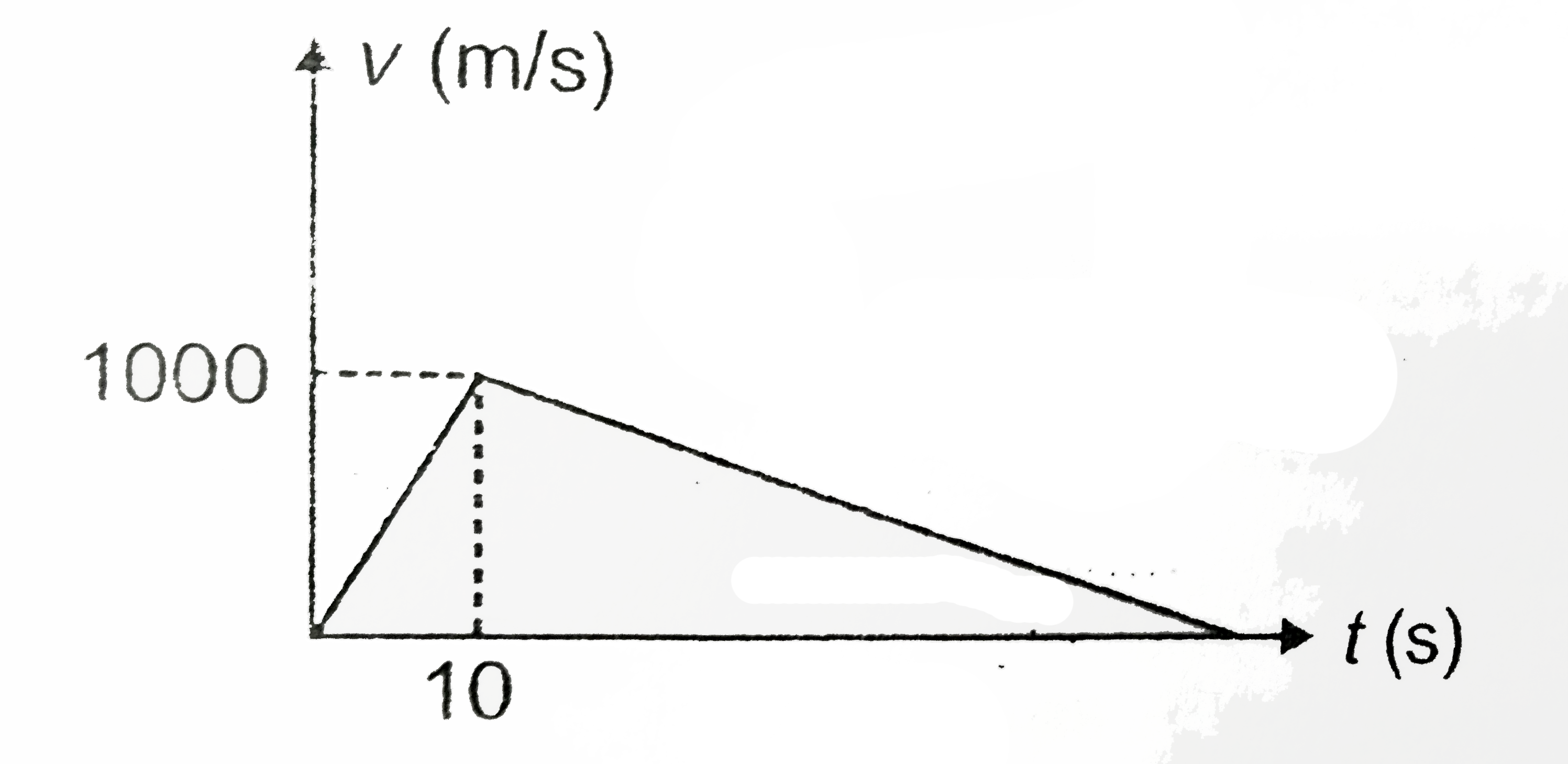
The maximum height attained by the rocket is
View SolutionIf the mass of a planet is 10% less than that of the earth and the radius is 20% greater than that of the earth, the acceleration due to gravity on the planet will be
View SolutionIf the radius of the earth decreases by 10%, the mass remaining unchanged, what will happen to the acceleration due to gravity?
View SolutionA small spherical ball (obeying Stoke's law for viscous force) is thrown up vertically with a speed 20ms−1 and is received back by the thrower at the point of projection with a speed 10ms−1. Neglecting the buoyant force on the ball, assuming the speed of the ball during its flight to be never equal to its terminal speed and taking the acceleration due to gravity g=10ms−2, find the time of flight of the ball in seconds.
View SolutionA small spherical ball (obeying Stoke's law for viscous force) is thrown up vertically with a speed 20ms−1 and is received back by the thrower at the point of projection with a speed 10ms−1. Neglecting the buoyant force on the ball, assuming the speed of the ball during its flight to be never equal to its terminal speed and taking the acceleration due to gravity g=10ms−2, find the time of flight of the ball in seconds.
View SolutionA man of mass 60 kg climbed down using an elevator . The elevator had an acceleration of 4ms−2 . If the acceleration due to gravity is 10ms−2 , the man's apparent weight on his way down is
View Solution

