A body is thrown with the velocity v0 at an angle of θ to the horizon. Determine v0inms−1 if the maximum height attained by the body is 5m and at the highest point of its trajectory the radius of curvature is r=3m. Neglect air resistance. [Use√80as9].
The correct Answer is:(9)
To solve the problem, we need to determine the initial velocity v0 of a projectile thrown at an angle θ given the maximum height h=5m and the radius of curvature r=3m at the highest point of its trajectory. We will neglect air resistance.
1. Understanding the Radius of Curvature:
The radius of curvature r at the highest point of the projectile's trajectory can be expressed as:
r=v20cos2θg
where g is the acceleration due to gravity (approximately 10m/s2).
2. Understanding Maximum Height:
The maximum height h attained by the projectile can be expressed as:
h=v20sin2θ2g
3. Relating the Two Equations:
From the two equations, we can express v20 in terms of r and h:
- From the radius of curvature equation:
v20=rg
- From the maximum height equation:
v20=2hg⋅1sin2θ
4. Adding the Two Expressions:
We can add the two expressions for v20:
rg+2hg=v20(sin2θ+cos2θ)
Since sin2θ+cos2θ=1, we have:
v20=rg+2hg
5. Substituting Values:
Now, substituting the known values:
- r=3m
- h=5m
- g=10m/s2
v20=(3)(10)+2(5)(10)=30+100=130
6. Calculating v0:
Taking the square root to find v0:
v0=√130
Since √80≈9, we can express √130 as:
√130=√(80+50)≈√80+√50≈9+7≈16
However, for exact calculations:
v0=√130≈11.4m/s
Final Answer:
The initial velocity v0 is approximately 11.4m/s.
The radius of curvature r at the highest point of the projectile's trajectory can be expressed as:
r=v20cos2θg
where g is the acceleration due to gravity (approximately 10m/s2).
The maximum height h attained by the projectile can be expressed as:
h=v20sin2θ2g
From the two equations, we can express v20 in terms of r and h:
- From the radius of curvature equation:
v20=rg
- From the maximum height equation:
v20=2hg⋅1sin2θ
We can add the two expressions for v20:
rg+2hg=v20(sin2θ+cos2θ)
Since sin2θ+cos2θ=1, we have:
v20=rg+2hg
Now, substituting the known values:
- r=3m
- h=5m
- g=10m/s2
v20=(3)(10)+2(5)(10)=30+100=130
Taking the square root to find v0:
v0=√130
Since √80≈9, we can express √130 as:
√130=√(80+50)≈√80+√50≈9+7≈16
However, for exact calculations:
v0=√130≈11.4m/s
The initial velocity v0 is approximately 11.4m/s.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Comprehension21videos
KINEMATICS-2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Integer9videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression2videos
KINETIC THEORY OF GASES
Similar Questions
A body is thrown with the velocity v0 at an angle of θ to the horizontal. Determine v0inms−1 if the maximum height attained by the body is 5m and at the highest point of its trajectory the radius of curvature is r=3m. Neglect air resistance. [Use√80=9].
View SolutionA particle of mass m is projected with velocity v at an angle θ with the horizontal. Find its angular momentum about the point of projection when it is at the highest point of its trajectory.
View Solution
Knowledge Check
- Question 1 - Select One
A body is thrown with a velocity of 9.8 m/s making an angle of 30∘ with the horizontal. It will hit the ground after a time
A3 sB2sC1.5 sD1 s
A body of mass m is projected with a velocity u at an angle θ with the horizontal. The angular momentum of the body, about the point of projection, when it at highest point on its trajectory is
View SolutionTwo bodies are thrown with the same initial velocity of 30 m/s. One at 17°, other at 73° to the horizontal. The sum of the maximum heights reached by them is [g = 10 m/s2]
View SolutionA particle is projected at angle θ with horizontal with velocity v0att=0. Find
(a) tangential and normal acceleration of the particle at t=0 and at highest point of its trajectory.
(b) the radius of curvature a=0 and highest point.
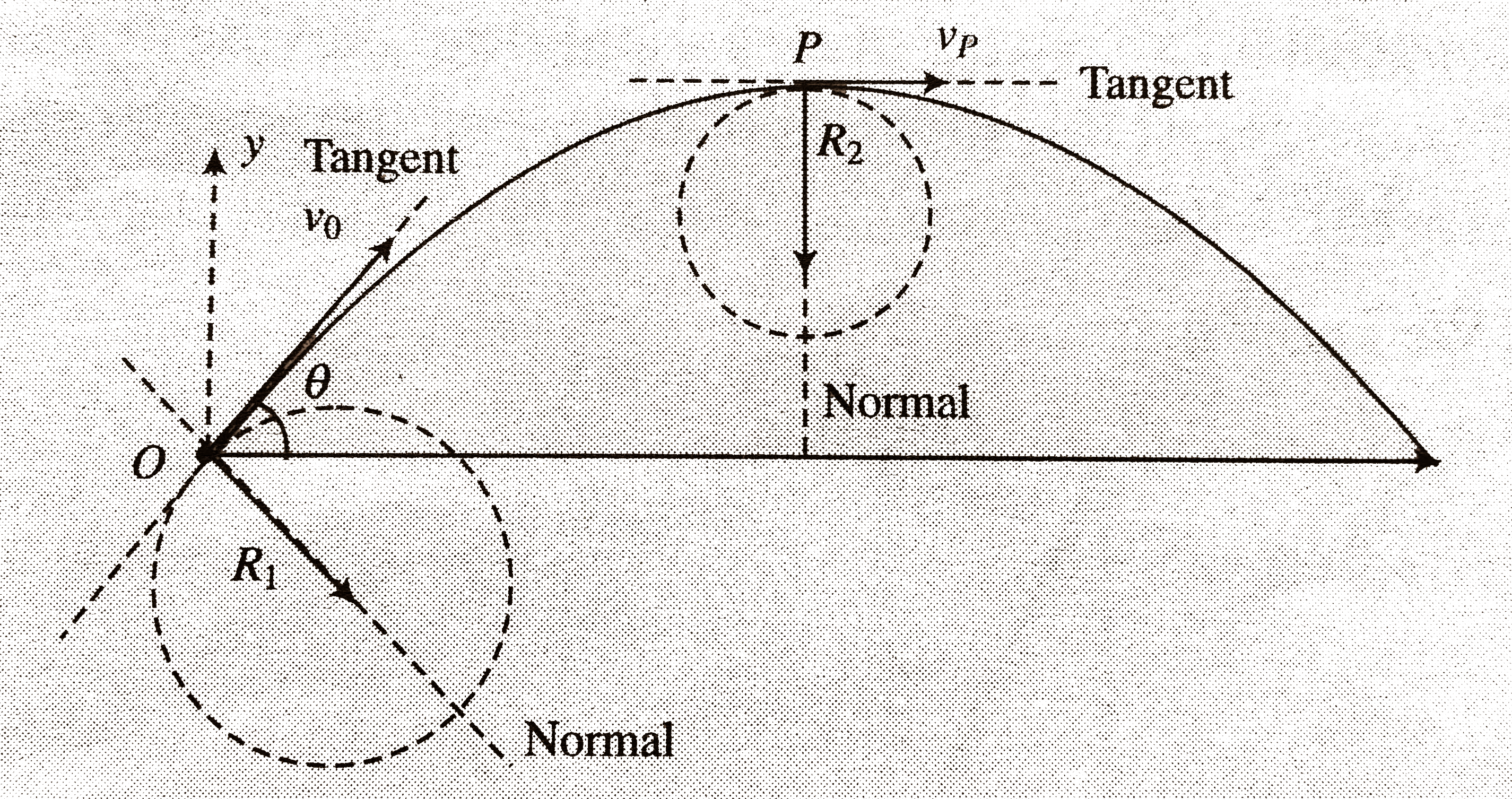 .View Solution
.View SolutionA particle is thrown vertically upwards. If its velocity is half of the maximum height is 20m/s, then maximum height attained by it is
View SolutionA particle is thrown vertically upward. Its velocity at half of the height is 10 m/s. Then the maximum height attained by it : -
(g=10m/s2)View SolutionA body is projected with a certain speed at angles of projection of θ and 90−θ The maximum height attained in the two cases are 20m and 10m respectively. The range for angle of projection θ is
View SolutionA particle of mass m is projected with a velocity v at an angle of θ with horizontal. The angular momentum of the particle at the highest point of its trajectory is equal to :
View Solution

