A block of mass m is placed on the inclined sufrace of a wedge as shown in fig. Calculate the acceleration of the wedge and the block when the block is released. Assume all surfaces are frictionless.

Method 1: Analysis form ground frame: Let the acceleration of wedge be A and that of block be a (w.r.t wedge).
Then, acceleration of m w.r.t ground is
→am=→am,M+→aM=(acosθˆi−asinθˆj)−Aˆi
=(acosθ−A)ˆi−asinθˆj...(i)
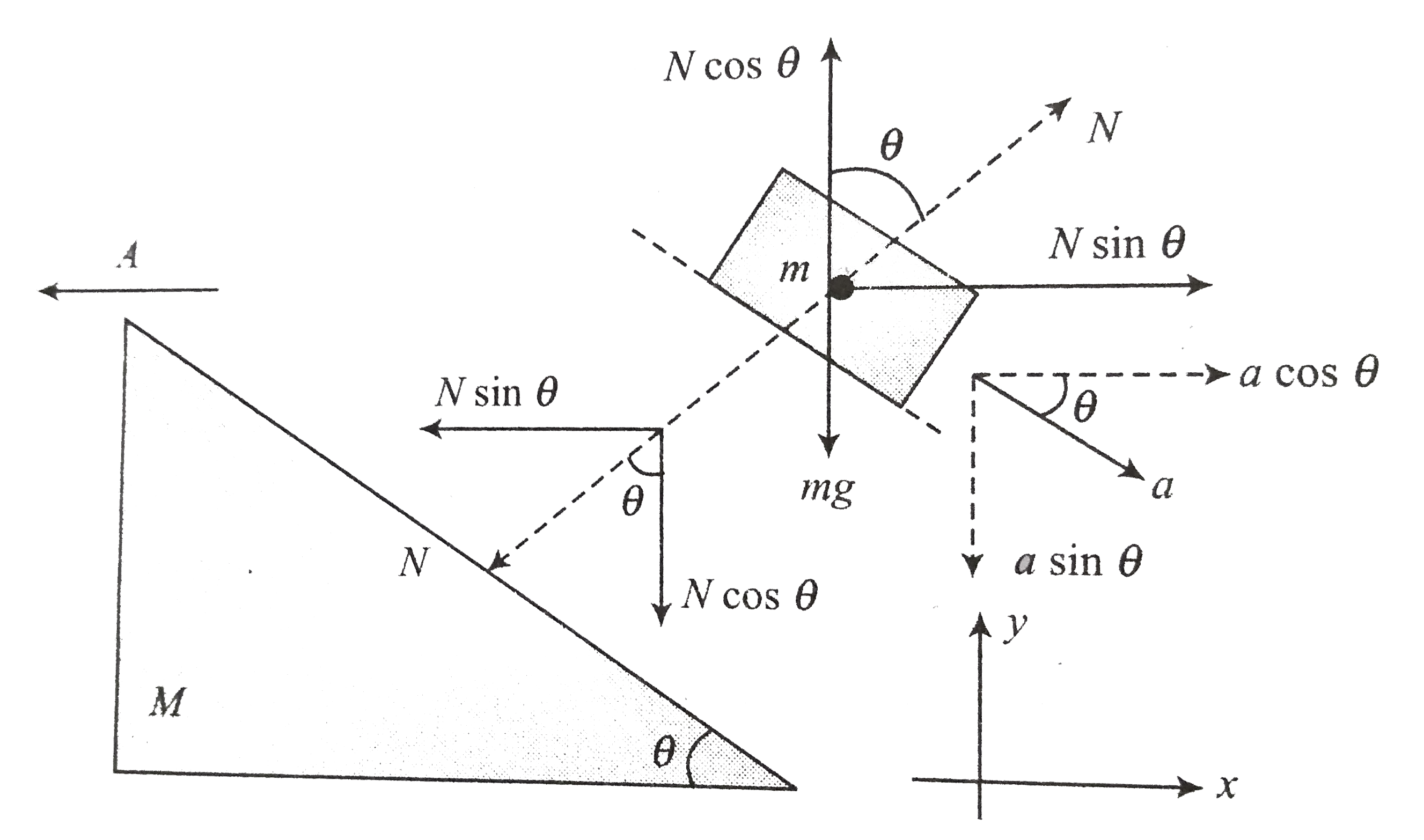
For M: Nsinθ=MA ...(ii)
For m: mg−Ncosθ=masinθ ...(iii)
Nsinθ=m(acosθ−A)...(iv)
Solve (ii), (iii) and (iv) to get
A=mgsinθcosθM+msin2θ and (M+m)gsinθM+msin2θ
Now acceleration of block can be found by putting the values of a in (i)
Method 2: If we consider the motion of block parallel and perpendicular to sloping surface w.r.t. ground, acceleration of m can also be written as :
→am=→am,M_→aM=−aˆi+(Acosθˆi+Asinθˆj)
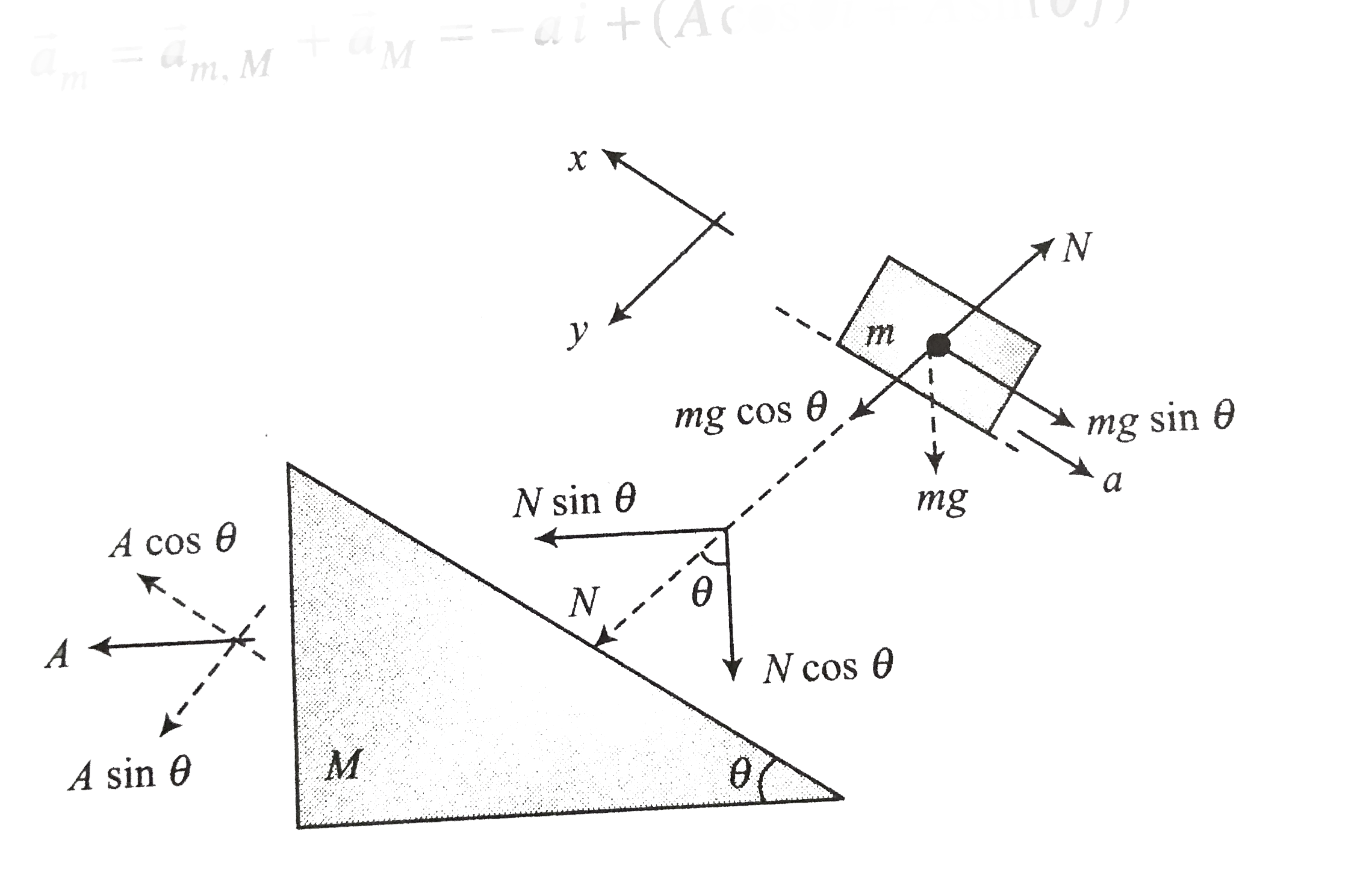
→am=(Acosθ−a)ˆi+Asinθˆj ...(v)
For M: Nsinθ=MA ...(vi)
For m: −mgsinθ=m(Acosθ−a) ...(vii)
mgcosθ−N=mAsinθ ...(viii)
From above equation we can get the valuve of
A=mgsinθcosθM+msin2θ and (M+m)gsinθM+msin2θ
Method 3: Analysis from non-inertial frame: With the help of pseudo force. If we consider the motion of block parallel and perpendicular to ground.

let us make teh FBD of m w.r.t. M.
For M: Nsinθ=MA...(ix)
For m: Nsinθ+mA=macosθ ...(x)
mg−Ncosθ=masinθ ...(xi)
From above equations, we can get the value of
A=mgsinθcosθM+msin2θ and (M+m)gsinθM+msin2θ
Method 4: Analyses from non-inertial frame: With the help of pseudo force.

When the forces are resolved in directions along the plane and perpendicular to the plene:
For M: Nsinθ=MA
for m: mgsinθ+mAcosθ=ma
mgcosθ=N+mAsinθ
From above equations, we can get the value of
A=mgsinθcosθM+msin2θ and (M+m)gsinθM+msin2θ
Method 5: Taking system together:
Considering wedge and block together as a system as shown in fig.

When the system is released, the wedge will start moving towards left with acceleration A and the block will starts sliding on the sloping surface of the wedge with acceleration a with respects to wedge as shown in fig.
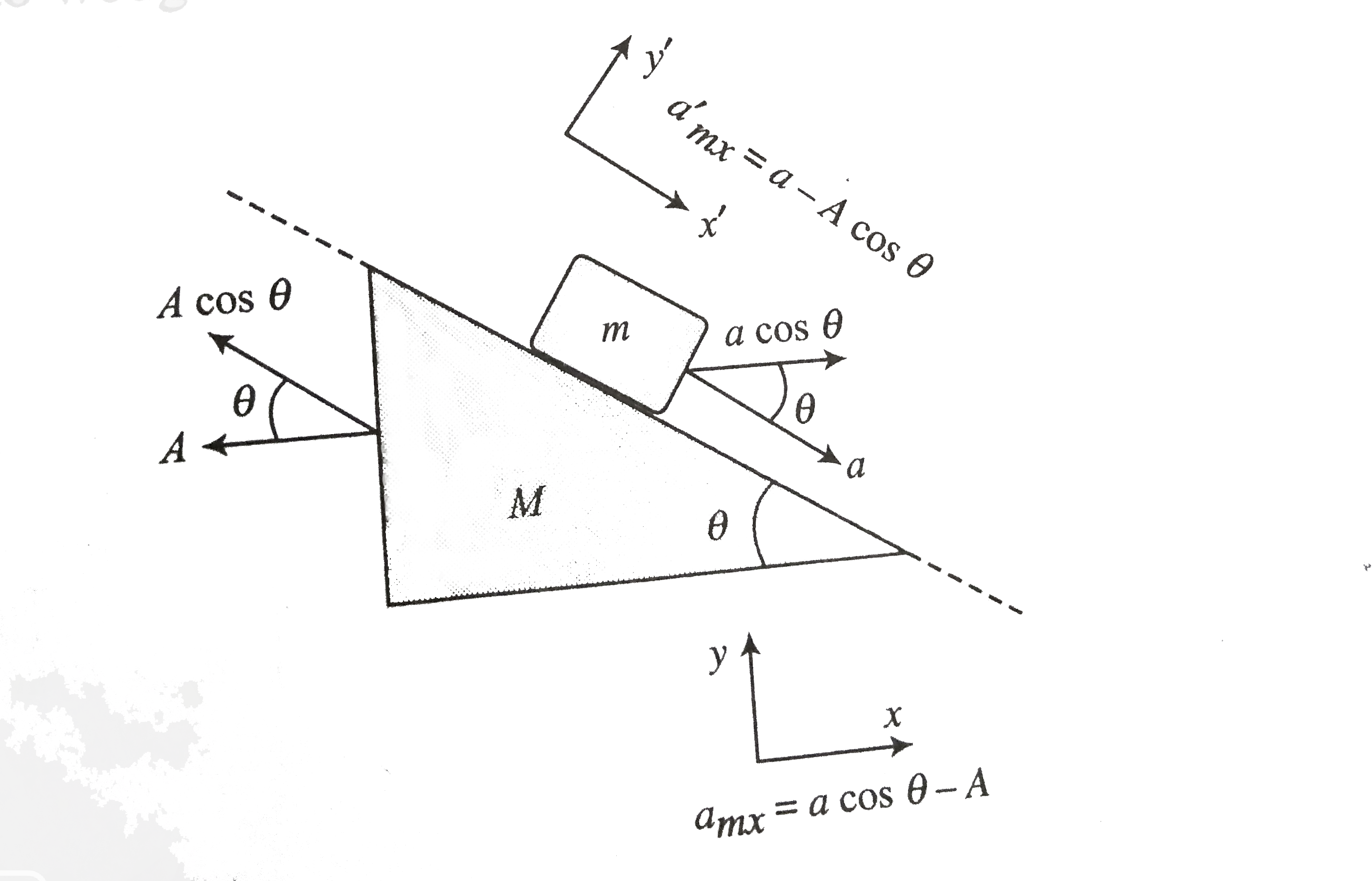
Now analyzing the system in the horizontal directon, the system is not having any external force in horizontal direction. So we can apply
Σ→Fx=Σmi→ai=m→am+M→aM
Σ→Fx=0⇒−MA+m(acosθ−A)=0
⇒a=(m+M)Amcosθ ..(i)
Now considering the motion of block parallel to the sloping side. Writing the equation of motion with respect to ground.
Σ→Fx'=Σm→ax'
⇒mgsinθ=ma'x
=m(a−Acosθ) ...(ii)
Solve (i) and (ii) to get
A=mgsinθcosθM+msin2θ and (M+m)gsinθM+msin2θ
Topper's Solved these Questions
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.1
11 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.2
35 Videos
- NEWTON'S LAWS OF MOTION 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer
5 Videos
- MISCELLANEOUS VOLUME 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE
10 Videos
- NEWTON'S LAWS OF MOTION 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type
1 Videos
Similar Questions
A block of mass m is placed on a smooth wedge of mass M, as shown in the figure . Just after placiing block m over wedge M.
View SolutionFind the minimum acceleration of the wedge, so that the block is under free fall (assume all surfaces tivate v are smooth).
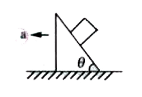
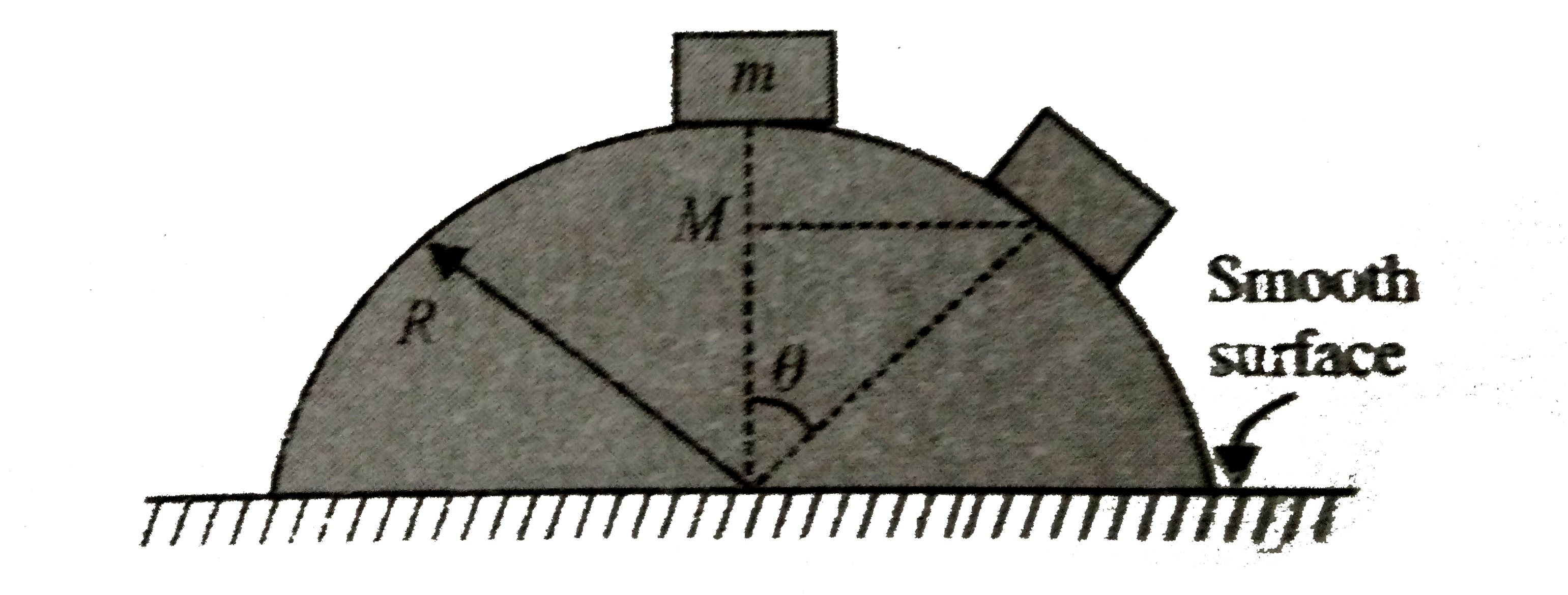
View SolutionA block is released on the convex surface of a hemispherical wedge as shown in Fig. Determine the displacement of the wedge when the block reaches the angular position θ
View SolutionA block of mass m is at rest on a rought wedge as shown in figure. What is the force exerted by the wedge on the block?

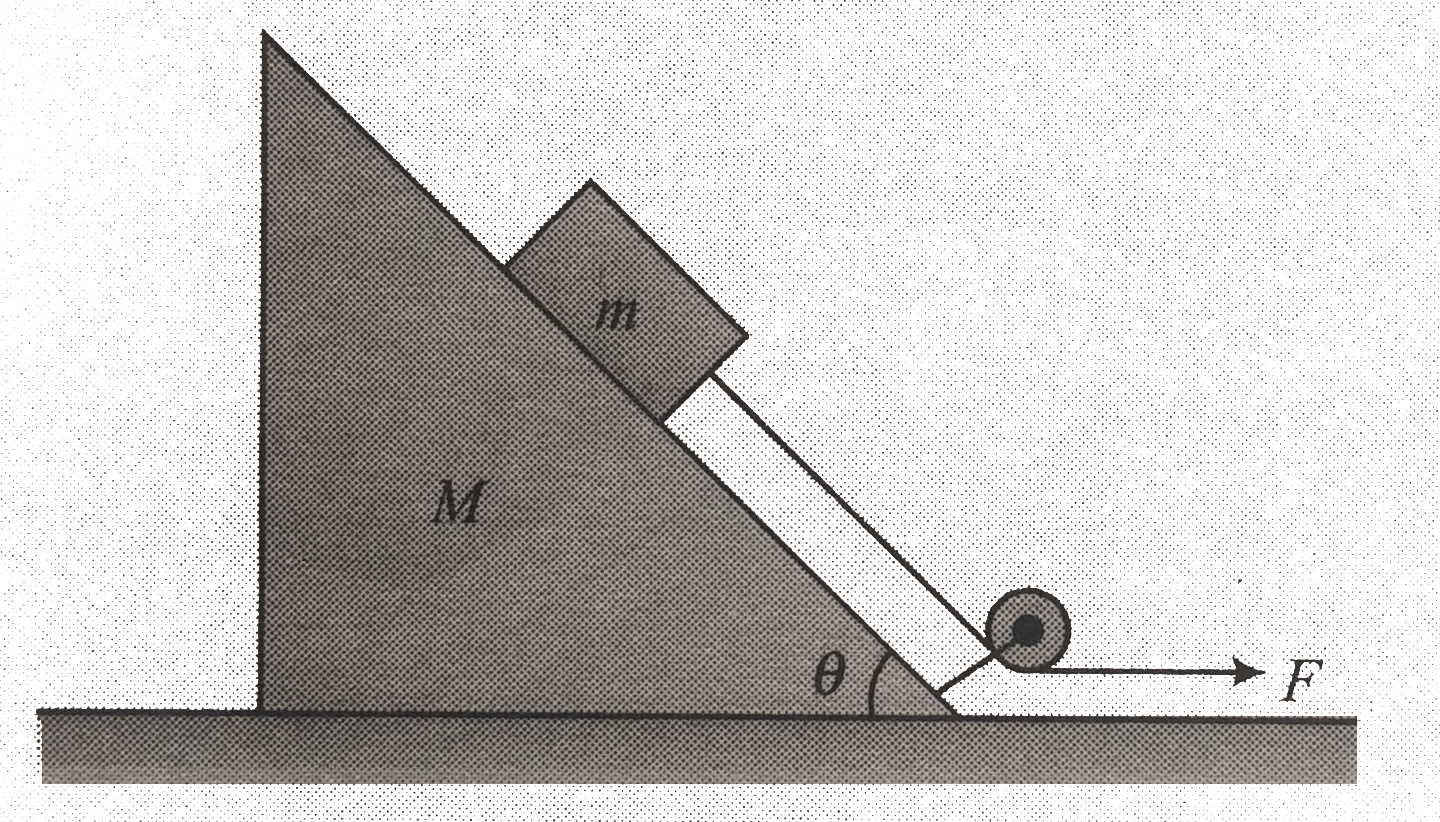
View SolutionIn fig. mass m is being pulled on the incline of a wedge of mass M. All the surfaces are smooth. Find the acceleration of the wedge.
View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?

View SolutionA block of mass m is at rest on a rough wedge as shown in figure. What is the force exerted by the wedge on the block?

View SolutionA block of mass 'm' is released on the top of a frictionless incline as shown in the figure. The time period of the oscillation of the block is

View SolutionA small block of mass m is kept on the inclined surface of a wedge of mass M. The inclined surface of the wedge makes an angle θ with the horizontal. The wedge is kept on a frictionless rigid horizontal floor. You can neglect friction between the block and the wedge also. An external agent applies a horizontal force F on the wedge.
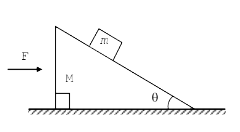
The value of the force F so that the wedge remains at rest is F1. Which of the options is incorrect?
View SolutionA small block of mass m is kept on the inclined surface of a wedge of mass M. The inclined surface of the wedge makes an angle θ with the horizontal. The wedge is kept on a frictionless rigid horizontal floor. You can neglect friction between the block and the wedge also. An external agent applies a horizontal force F on the wedge.

The value of the force F so that the wedge remains at rest is F1. Which of the options is incorrect?
View Solution
