A system of two blocks is placed on a rought horizontal surface as shown in figure below The coefficient of staic and kinetic friction at two surfaces are shown A force F is horizontal applied on the upper block as shown .Let f1,f2 represent the frictional force between upper and lower surface of contact respectively and a1,a2 represent the acceleration of 3kgand2kg block respectively

For F=12N mark the correct option
For a particular value of F(<F0) there is no motion at any of the contact surface
The value of F0is 10N
The F increases beyond F0,f1 increases and contitaes to increase unit it limiting value
All of the above
The correct Answer is:d
From the data given we can find the limiting friction force for the two surface
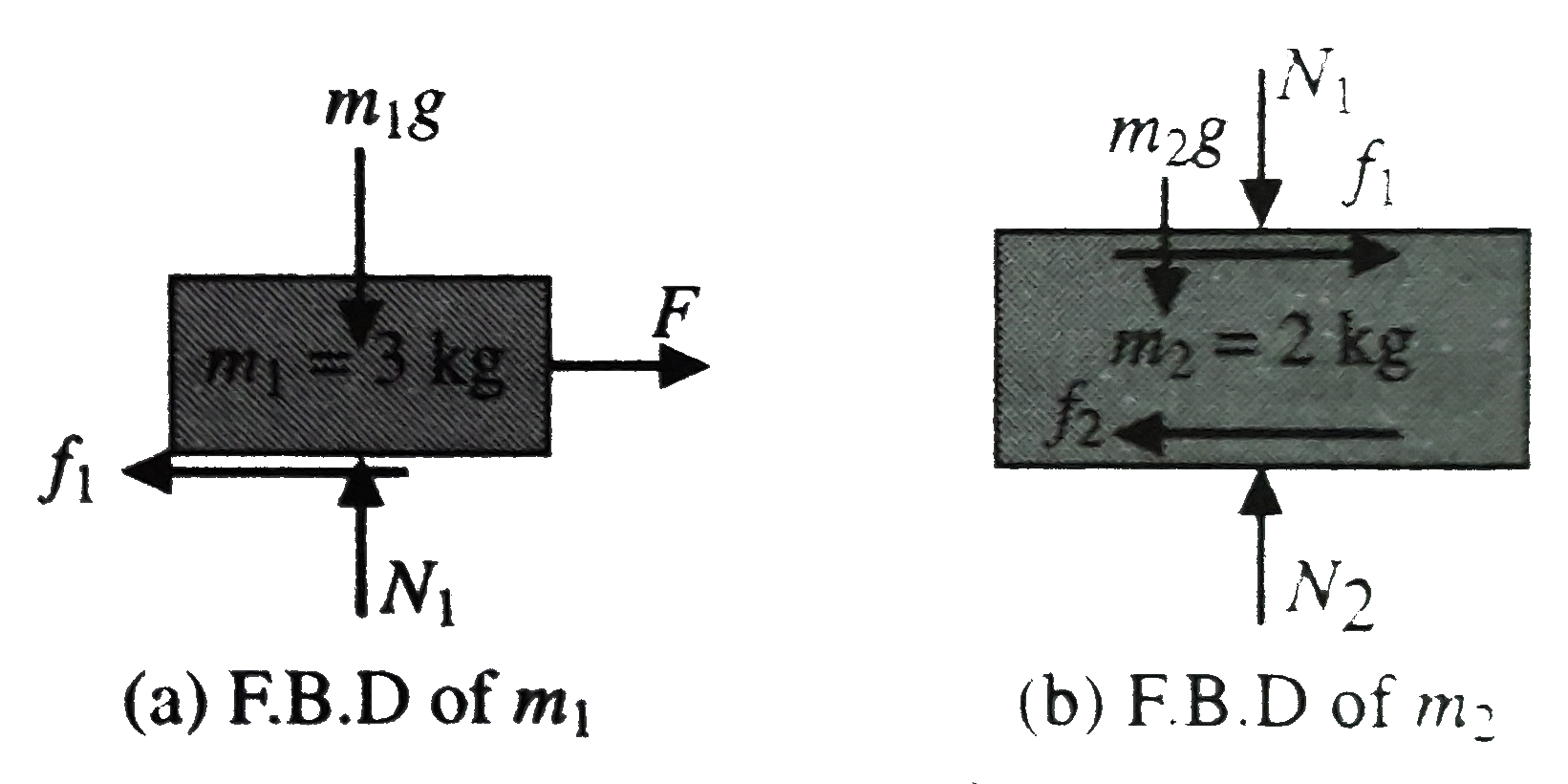
fL1=0.5×3×10=15N
fL2=0.2×5×10=10N
F<fL3
Both the blocks remain at rest and f1=F,f1=f1 and a1 and a2 For F>ft2 and F is less than a correct value anyF1 the motion stars at lower surface but both the blocks surface continue to move with starts same acceleration The friction on surface becomes kinetic in nature
Here a=a1=a2=F−ft25ms−2
F−f1=3a and f1−fk=2a
All these equation give
f1=2F−3ft25
for relative motion to start between two blocks f1>ft2F>30Nso minimum value to F is relative motion between blocks is 30N for f=12N,f1=7.8N
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer6videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - True And False4videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Multiple Correct2videos
NEWTON'S LAWS OF MOTION 2
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Integer5videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
Similar Questions
A system of two blocks is placed on a rought horizontal surface as shown in figure below The coefficient of staic and kinetic friction at two surfaces are shown A force F is horizontal applied on the upper block as shown .Let f1,f2 represent the frictional force between upper and lower surface of contact respectively and a1,a2 represent the acceleration of 3kgand2kg block respectively

For relative motion to be there between two blocks the minimum value of F should beView SolutionA block is kept on a rough horizontal surface as shown. Its mass is 2 kg and coefficient of friction between block and surface (μ)=0.5. A horizontal force F is acting on the block. When
 View Solution
View SolutionA block is kept on a rough surface and applied with a horizontal force as shown which is gradually increasing for zero. The coefficient of static and kinetic friction are 1/√3 then
 View Solution
View SolutionA block of mass m is moving on a rough horizontal surface. μ is the coefficient of kinetic friction between the block and the surface. What is the net force exerted by the surface on the block?
View SolutionA block is sliding on a rough horizontal surface. If the contact force on the block is √2 times the frictional force, the coefficient of friction is
View SolutionA force shown in the F−x graph is applied to a 2kg block horizontal as shown in figure. The change in kinetic energy is
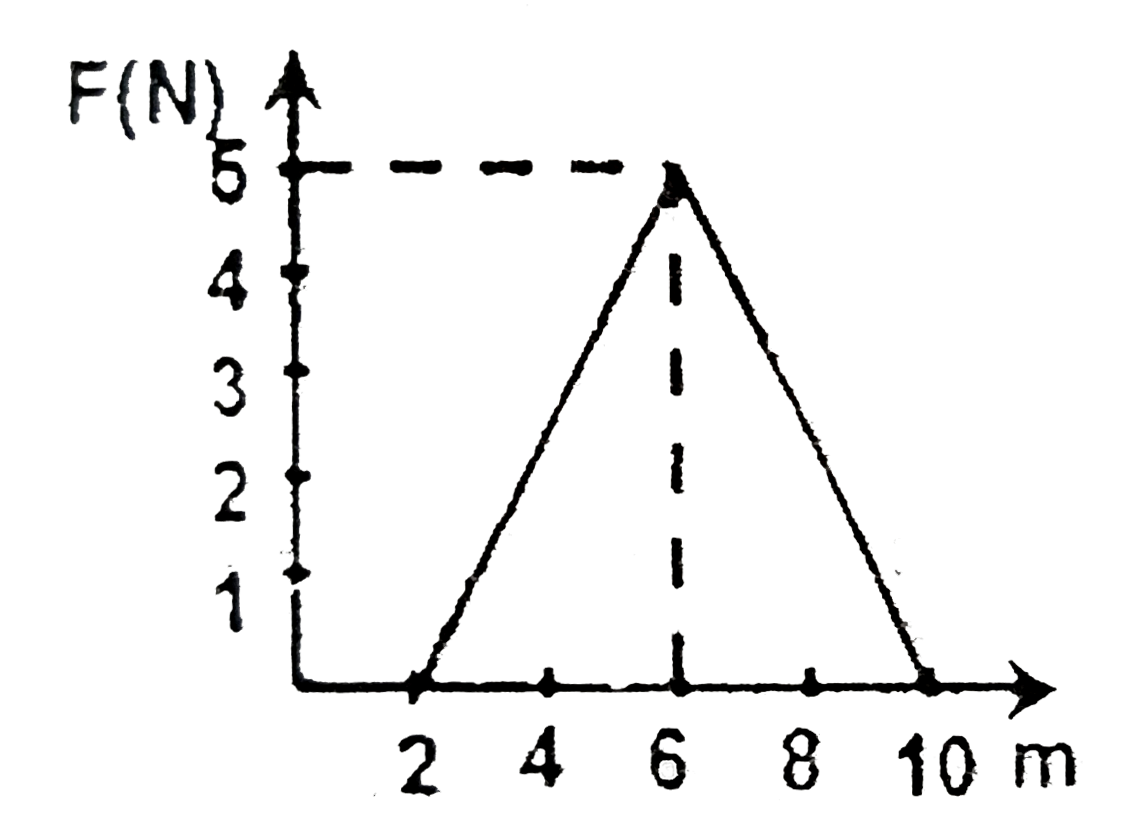 View Solution
View SolutionA block of mass 1kg is at rest on a rough horizontal surface having coefficient of static friction 0.2 and kinetic friciton 0.15, find the frictional forces if a horizontal force,
(a) F=1N (b) F=1.96N (c) F=2.5N is applied on a block
 View Solution
View SolutionA force of 100 N is applied on a block of mass 3 kg as shown in figure. The coefficient of friction between the surface and the block is 14 .Then the friction force acting on the block is
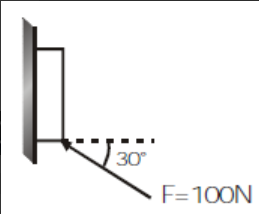 View Solution
View SolutionA force of 100N is applied on a block of mass 3kg as shown below. The coefficient of friction between wall and the block is 1/4. The friction force acting on the block is :
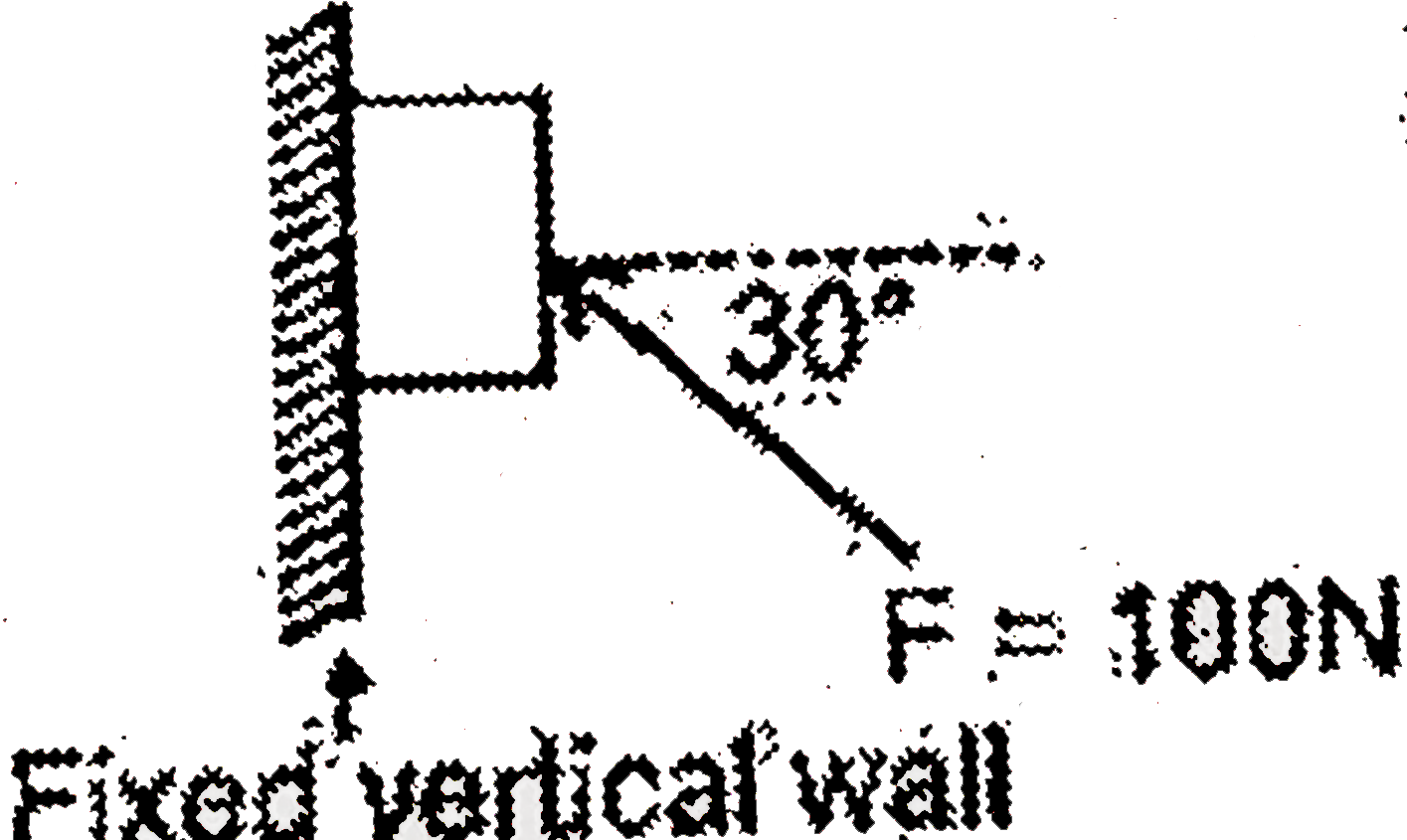 View Solution
View SolutionA block of mass 5 kg is placed on a rough horizontal plane . The coefficients of stastic and kinetic friction between the block and surface are 0⋅5and0⋅3 respectively. A force of 100 N is applied at an angle 30∘ with the horizontal as shown in the figure . The acceleration of the block will be [ g = 10m/s2]
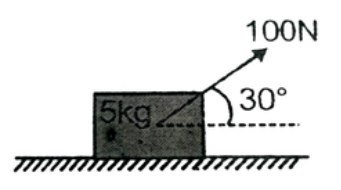 View Solution
View Solution

