A continuous stream of particles, of mass m and velocity r, is emitted from a source at a rate of n per second. The particles travel along a straight line, collide with a body of mass M and get embedded in the body. If the mass M was originally at rest, its velocity when it has received N particles will be
mnNNm+M
mvNNM+M
mvNm+M
Nm+MNM
The correct Answer is:B
To solve the problem, we will use the principle of conservation of momentum. Here's the step-by-step solution:
Step 1: Understand the system
We have a continuous stream of particles, each with mass m and traveling at velocity r. These particles collide with a stationary body of mass M and get embedded in it. We need to find the velocity of the combined mass after N particles have been embedded.
Step 2: Calculate the total mass after N particles are embedded
When N particles, each of mass m, collide with the body of mass M, the total mass of the system after the collision will be:
\(
\text{Total mass} = M + N \cdot m
\)
Step 3: Apply the conservation of momentum
Before the collision, the momentum of the system is due to the particles only, as the body of mass M is at rest. The momentum of N particles is given by:
\(
\text{Initial momentum} = N \cdot m \cdot r
\)
After the collision, the total momentum of the combined mass (body + embedded particles) moving with velocity V is:
\(
\text{Final momentum} = (M + N \cdot m) \cdot V
\)
According to the conservation of momentum:
\(
\text{Initial momentum} = \text{Final momentum}
\)
Thus, we can write:
\(
N \cdot m \cdot r = (M + N \cdot m) \cdot V
\)
Step 4: Solve for the final velocity V
Rearranging the equation to solve for V gives:
\(
V = \frac{N \cdot m \cdot r}{M + N \cdot m}
\)
Conclusion
The velocity of the body after it has received N particles is:
\(
V = \frac{N \cdot m \cdot r}{M + N \cdot m}
\)
We have a continuous stream of particles, each with mass m and traveling at velocity r. These particles collide with a stationary body of mass M and get embedded in it. We need to find the velocity of the combined mass after N particles have been embedded.
When N particles, each of mass m, collide with the body of mass M, the total mass of the system after the collision will be:
\(
\text{Total mass} = M + N \cdot m
\)
Before the collision, the momentum of the system is due to the particles only, as the body of mass M is at rest. The momentum of N particles is given by:
\(
\text{Initial momentum} = N \cdot m \cdot r
\)
After the collision, the total momentum of the combined mass (body + embedded particles) moving with velocity V is:
\(
\text{Final momentum} = (M + N \cdot m) \cdot V
\)
According to the conservation of momentum:
\(
\text{Initial momentum} = \text{Final momentum}
\)
Thus, we can write:
\(
N \cdot m \cdot r = (M + N \cdot m) \cdot V
\)
Rearranging the equation to solve for V gives:
\(
V = \frac{N \cdot m \cdot r}{M + N \cdot m}
\)
The velocity of the body after it has received N particles is:
\(
V = \frac{N \cdot m \cdot r}{M + N \cdot m}
\)
Topper's Solved these Questions
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Multiple Correct
25 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion - Reasoning
2 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Subjective
23 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example
13 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
Similar Questions
Knowledge Check
The escape velocity of a particle of mass 'm'
Avaries as m2Bvaries as mCvaries as m−1Dis independent of mass
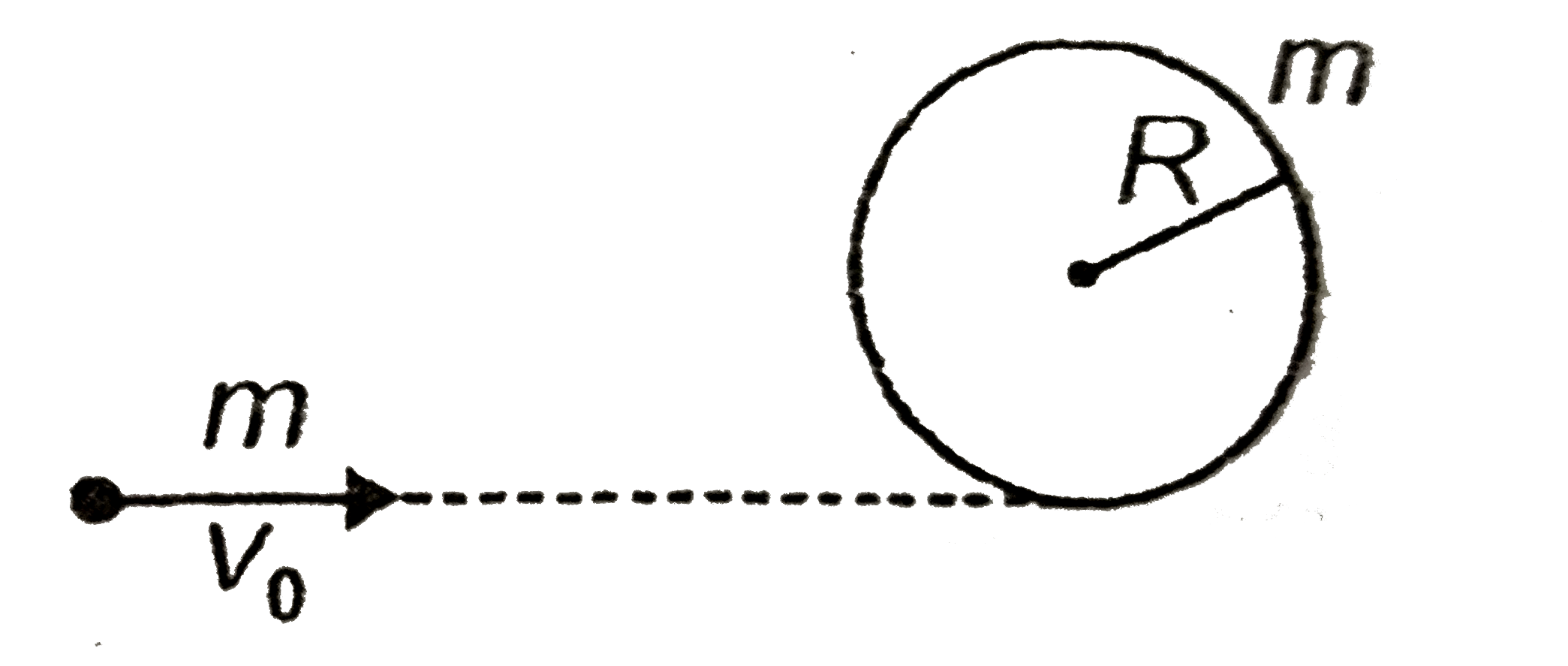
A disc of mass m and radius R is kept on a smooth horizontal surface with its plane parallel to the surface. A particle of same mass m travelling with speed v0 collides with the stationery disc and gets embedded into it as shown in the figure. Then
View SolutionA particle of mass m moves along line PC with velocity v as shown. What is the angular momentum of the particle about O?

View SolutionThe momentum of a particle of mass of m moving with a velocity v is p= mv. What is the rate of change of momentum ? Assume that m is constant .
View SolutionA particle of mass 2m moving with velocity v strikes a stationary particle of mass 3m and sticks to it . The speed of the system will be
View SolutionA particle of mass m moving with a velocity (3ˆi+2ˆj)ms−1 collides with another body of mass M and finally moves with velocity (−2ˆi+ˆj)ms−1. Then during the collision
View SolutionForce acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K/V K is constant then speed of the particle in time t
View SolutionA machine gun fires a steady stream of bullets at the rate of n per minute into a stationary target in which the bullets get embedded. If each bullet has a mass m and arrives at the target with a velocity v, the average force on the target is
View Solution
