Force acting on a particle of mass m moving in straight line varies with the velocity of the particle as F=K/V K is constant then speed of the particle in time t
√ktm
√2ktm
2√ktm
none of these
The correct Answer is:B
To solve the problem, we need to find the speed of a particle of mass m as a function of time t, given that the force acting on it varies with its velocity v as F=Kv, where K is a constant.
1. Start with Newton's Second Law:
According to Newton's second law, the force acting on a particle is equal to the mass of the particle multiplied by its acceleration:
F=mdvdt
2. Substitute the Given Force:
We know that the force F is given by F=Kv. Therefore, we can equate the two expressions for force:
mdvdt=Kv
3. Rearranging the Equation:
To separate the variables, we can multiply both sides by v and rearrange:
vdv=Kmdt
4. Integrate Both Sides:
Now we integrate both sides. The left side will be integrated with respect to v from 0 to v, and the right side will be integrated with respect to t from 0 to t:
∫v0vdv=∫t0Kmdt
5. Calculate the Integrals:
The integral on the left side is:
12v2∣∣∣v0=12v2
The integral on the right side is:
Kmt∣∣∣t0=Kmt
6. Set the Results Equal:
Setting the results of the integrals equal gives us:
12v2=Kmt
7. Solve for Velocity v:
To find v, we multiply both sides by 2:
v2=2Kmt
Taking the square root of both sides gives:
v=√2Kmt
Final Result:
The speed of the particle at time t is:
v(t)=√2Kmt
According to Newton's second law, the force acting on a particle is equal to the mass of the particle multiplied by its acceleration:
F=mdvdt
We know that the force F is given by F=Kv. Therefore, we can equate the two expressions for force:
mdvdt=Kv
To separate the variables, we can multiply both sides by v and rearrange:
vdv=Kmdt
Now we integrate both sides. The left side will be integrated with respect to v from 0 to v, and the right side will be integrated with respect to t from 0 to t:
∫v0vdv=∫t0Kmdt
The integral on the left side is:
12v2∣∣∣v0=12v2
The integral on the right side is:
Kmt∣∣∣t0=Kmt
Setting the results of the integrals equal gives us:
12v2=Kmt
To find v, we multiply both sides by 2:
v2=2Kmt
Taking the square root of both sides gives:
v=√2Kmt
The speed of the particle at time t is:
v(t)=√2Kmt
Similar Questions
Forces acting on a particle moving in a straight line varies with the velocity of the particle as F=αυ where α is constant. The work done by this force in time interval Δt is :
View SolutionThe power supplied by a force acting on a particle moving in a straight line is constant. The velocity of the particle varies with displacement as x1K. Find the value of K.
View SolutionA time varying force, F=2t is acting on a particle of mass 2kg moving along x-axis. velocity of the particle is 4m/s along negative x-axis at time t=0. Find the velocity of the particle at the end of 4s.
View SolutionThe power supplied by a force acting on a particle moving in a straight line is constant. The velocity of the particle varies with the displacement x as :
View SolutionThe velocity-time graph of a particle moving in a straight line is shown in figure. The mass of the particle is 2kg. Work done by all the forces acting on the particle in time interval between t=0 to t=10s is

View SolutionThe displacement of a particle of mass 2kg moving in a straight line varies with times as x=(2t3+2)m. Impulse of the force acting on the particle over a time interval between t = 0 and t = 1 s is
View SolutionPower of the only force acting on a particle of mass m=1 kg moving in straight line depends on its velocity as P=v2 where v is in m/s and P is in watt. If initial velocity of the particle is 1m/s, then the displacement of the particle in ln2 second will be :
View SolutionKinetic energy of a particle moving in a straight line varies with time t as K=4t2. The force acting on the particle
View SolutionA particle of mass m initially moving with speed v.A force acts on the particle f=kx where x is the distance travelled by the particle and k is constant. Find the speed of the particle when the work done by the force equals W.
View SolutionThe force F acting on a particle is moving in a straight line as shown in figure. What is the work done by the force on the particle in the 4 m of the trajectory?
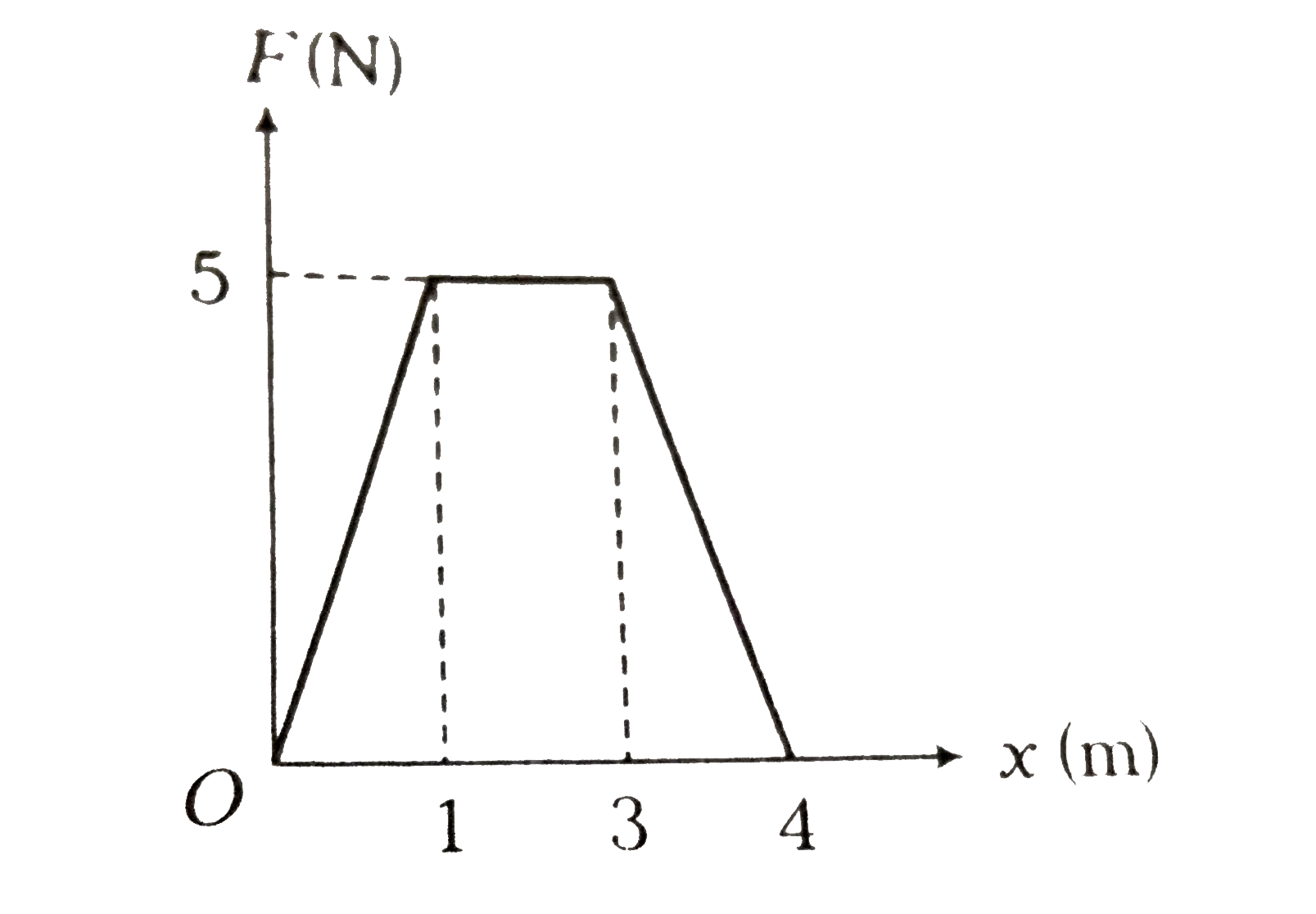
View Solution
