Let l be the moment of inertia of a uniform square plate about an axis AB that passes through its centre and is parallel to two of its sides. CD is a line in the plane of the plate that passes through the centre of the plate and makes an angle θ with AB. The moment of inertia of the plate about the axis CD is then equal to
I
Isin2θ
Icos2θ
Icos2(θ2)
The correct Answer is:A
To find the moment of inertia of a uniform square plate about an axis CD that passes through its center and makes an angle θ with the axis AB, we can follow these steps:
1. Identify the Moment of Inertia about Axis AB:
- The moment of inertia I of a uniform square plate about an axis AB that passes through its center and is parallel to two of its sides is given as I.
2. Use the Perpendicular Axis Theorem:
- According to the perpendicular axis theorem, for a planar object, the moment of inertia about an axis perpendicular to the plane (let's call it axis z) is the sum of the moments of inertia about two perpendicular axes in the plane (x and y).
- Therefore, we can write:
Iz=Ix+Iy
- Since the plate is uniform and symmetric, we have Ix=Iy=I. Thus,
Iz=I+I=2I
3. Consider the Moment of Inertia about Axis CD:
- Now, we need to find the moment of inertia about the axis CD, which makes an angle θ with the axis AB.
- Let’s denote the moment of inertia about the axis CD as I0.
4. Analyze the Axes:
- The axis CD can be resolved into two components: one along AB and one perpendicular to AB.
- By symmetry, if we consider the axis perpendicular to CD (let's call it C'D'), the moment of inertia about C'D' will also be I0.
5. Apply the Perpendicular Axis Theorem Again:
- For the axes CD and C'D', we can apply the perpendicular axis theorem:
Iz=I0+I0
- This implies:
Iz=2I0
6. Relate the Moments of Inertia:
- From the previous step, we know that Iz=2I. Therefore, we can set the equations equal to each other:
2I=2I0
- Dividing both sides by 2 gives:
I=I0
7. Conclusion:
- Thus, the moment of inertia of the plate about the axis CD, which makes an angle θ with the axis AB, is:
I0=I
Final Answer:
The moment of inertia of the plate about the axis CD is equal to I.
- The moment of inertia I of a uniform square plate about an axis AB that passes through its center and is parallel to two of its sides is given as I.
- According to the perpendicular axis theorem, for a planar object, the moment of inertia about an axis perpendicular to the plane (let's call it axis z) is the sum of the moments of inertia about two perpendicular axes in the plane (x and y).
- Therefore, we can write:
Iz=Ix+Iy
- Since the plate is uniform and symmetric, we have Ix=Iy=I. Thus,
Iz=I+I=2I
- Now, we need to find the moment of inertia about the axis CD, which makes an angle θ with the axis AB.
- Let’s denote the moment of inertia about the axis CD as I0.
- The axis CD can be resolved into two components: one along AB and one perpendicular to AB.
- By symmetry, if we consider the axis perpendicular to CD (let's call it C'D'), the moment of inertia about C'D' will also be I0.
- For the axes CD and C'D', we can apply the perpendicular axis theorem:
Iz=I0+I0
- This implies:
Iz=2I0
- From the previous step, we know that Iz=2I. Therefore, we can set the equations equal to each other:
2I=2I0
- Dividing both sides by 2 gives:
I=I0
- Thus, the moment of inertia of the plate about the axis CD, which makes an angle θ with the axis AB, is:
I0=I
The moment of inertia of the plate about the axis CD is equal to I.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Multiple Correct9videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Linked Comprehension28videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Subjective13videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger2videos
RIGID BODY DYNAMICS 2
Similar Questions
The moment of inertia of a copper disc, rotating about an axis passing through its centre and perpendicular to its plane
View SolutionFind the moment of inertia of a uniform square plate of mass M and edge a about one of its diagonals.
View SolutionFind the moment of inertia of a uniform square plate of mass M and edge a about one of its diagonals.
View SolutionThe moment of inertia of a uniform rod of length 2l and mass m about an axis xy passing through its centre and inclined at an enable α is
 View Solution
View SolutionThe moment of inertia of a cube of mass M and edge length a about an axis passing through one of its edge is
View SolutionThe moment of inertia of a thin square plate ABCD of uniform thickness about an axis passing through the centre O and perpendicular to plate is
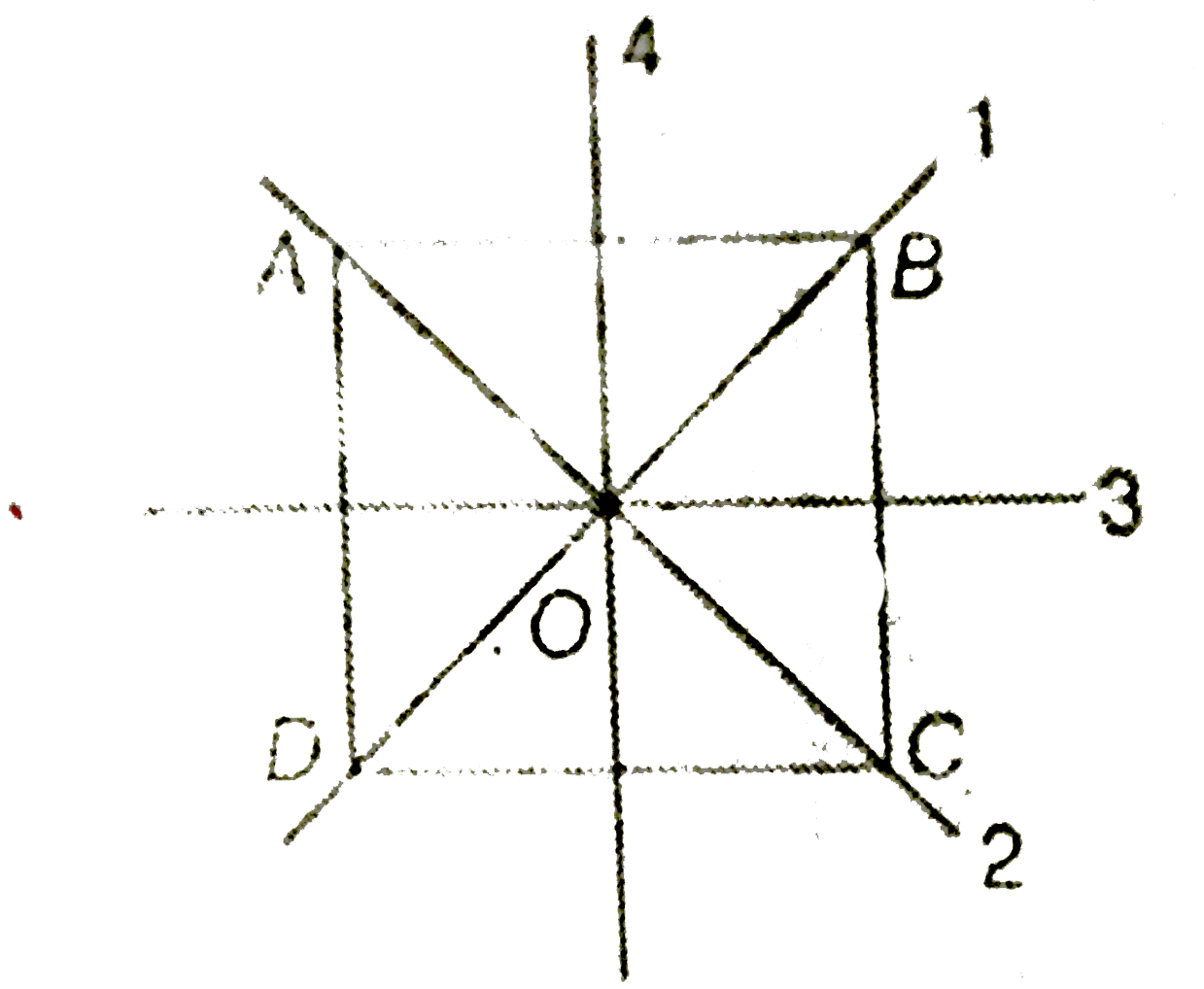 View Solution
View SolutionMoment of inertia of a ring of mass M and radius R about an axis passing through the centre and perpendicular to the plane is
View SolutionCalculate the moment of inertia of a ring of mass 2kg and radius 2cm about an axis passing through its centre and perpendicular to its surface.
View SolutionCalculate the moment of inertia of a ring of mass 2kg and radius 2cm about an axis passing through its centre and perpendicular to its surface.
View SolutionMoment of inertia of a uniform quarter disc of radius R and mass M about an axis through its centre of mass and perpendicular to its plane is :
View Solution

