A cubical block of wood 10cm along each side floats at the interface between an oil and water with its lowest surface 2cm below the interface. If the height of oil and water columns are 10cm each and ρoil=0.8gcc, find a the mass of the block, b. the liquid pressure at the lower surface of the block.
To solve the problem, we will break it down into two parts: finding the mass of the block and calculating the liquid pressure at the lower surface of the block.
Part (a): Finding the Mass of the Block
1. Identify the dimensions and submerged volume:
- The block is a cube with a side length of 10 cm.
- The lowest surface of the block is submerged 2 cm in water and 8 cm in oil.
- The area of the base of the block (cross-section) is:
A=side2=(10cm)2=100cm2
2. Calculate the volume submerged in water and oil:
- Volume submerged in water:
Vwater=A×height submerged in water=100cm2×2cm=200cm3
- Volume submerged in oil:
Voil=A×height submerged in oil=100cm2×8cm=800cm3
3. Calculate the buoyant force from water and oil:
- Density of water (ρwater=1g/cm3):
Fbuoyant, water=ρwater×Vwater×g=1g/cm3×200cm3×g=200g⋅g
- Density of oil (ρoil=0.8g/cm3):
Fbuoyant, oil=ρoil×Voil×g=0.8g/cm3×800cm3×g=640g⋅g
4. Total buoyant force:
Fbuoyant, total=Fbuoyant, water+Fbuoyant, oil=200g⋅g+640g⋅g=840g⋅g
5. Equate buoyant force to weight of the block:
Fbuoyant, total=m⋅g⟹840g⋅g=m⋅g
- Thus, the mass of the block is:
m=840g
Part (b): Finding the Liquid Pressure at the Lower Surface of the Block
1. Identify the pressure contributions:
- The pressure at the lower surface of the block is due to the oil and the water above it.
- The depth of the oil above the lower surface of the block is 10 cm (height of the oil) and the depth of the water above the lower surface is 2 cm (height submerged in water).
2. Calculate the pressure due to oil:
Poil=ρoil×g×hoil=0.8g/cm3×g×10cm
3. Calculate the pressure due to water:
Pwater=ρwater×g×hwater=1g/cm3×g×2cm
4. Total pressure at the lower surface:
Ptotal=Poil+Pwater=(0.8×10+1×2)g/cm2⋅g=(8+2)g/cm2⋅g=10g/cm2⋅g
5. Convert to SI units:
- To convert to Pascals, multiply by 980cm/s2:
Ptotal=10g/cm2⋅g×980cm/s2=9800Pa
Final Answers:
- a. The mass of the block is 840g.
- b. The liquid pressure at the lower surface of the block is 9800Pa.
- The block is a cube with a side length of 10 cm.
- The lowest surface of the block is submerged 2 cm in water and 8 cm in oil.
- The area of the base of the block (cross-section) is:
A=side2=(10cm)2=100cm2
- Volume submerged in water:
Vwater=A×height submerged in water=100cm2×2cm=200cm3
- Volume submerged in oil:
Voil=A×height submerged in oil=100cm2×8cm=800cm3
- Density of water (ρwater=1g/cm3):
Fbuoyant, water=ρwater×Vwater×g=1g/cm3×200cm3×g=200g⋅g
- Density of oil (ρoil=0.8g/cm3):
Fbuoyant, oil=ρoil×Voil×g=0.8g/cm3×800cm3×g=640g⋅g
Fbuoyant, total=Fbuoyant, water+Fbuoyant, oil=200g⋅g+640g⋅g=840g⋅g
Fbuoyant, total=m⋅g⟹840g⋅g=m⋅g
- Thus, the mass of the block is:
m=840g
- The pressure at the lower surface of the block is due to the oil and the water above it.
- The depth of the oil above the lower surface of the block is 10 cm (height of the oil) and the depth of the water above the lower surface is 2 cm (height submerged in water).
Poil=ρoil×g×hoil=0.8g/cm3×g×10cm
Pwater=ρwater×g×hwater=1g/cm3×g×2cm
Ptotal=Poil+Pwater=(0.8×10+1×2)g/cm2⋅g=(8+2)g/cm2⋅g=10g/cm2⋅g
- To convert to Pascals, multiply by 980cm/s2:
Ptotal=10g/cm2⋅g×980cm/s2=9800Pa
- a. The mass of the block is 840g.
- b. The liquid pressure at the lower surface of the block is 9800Pa.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Solved Examples
7 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Exercise 4.1
11 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
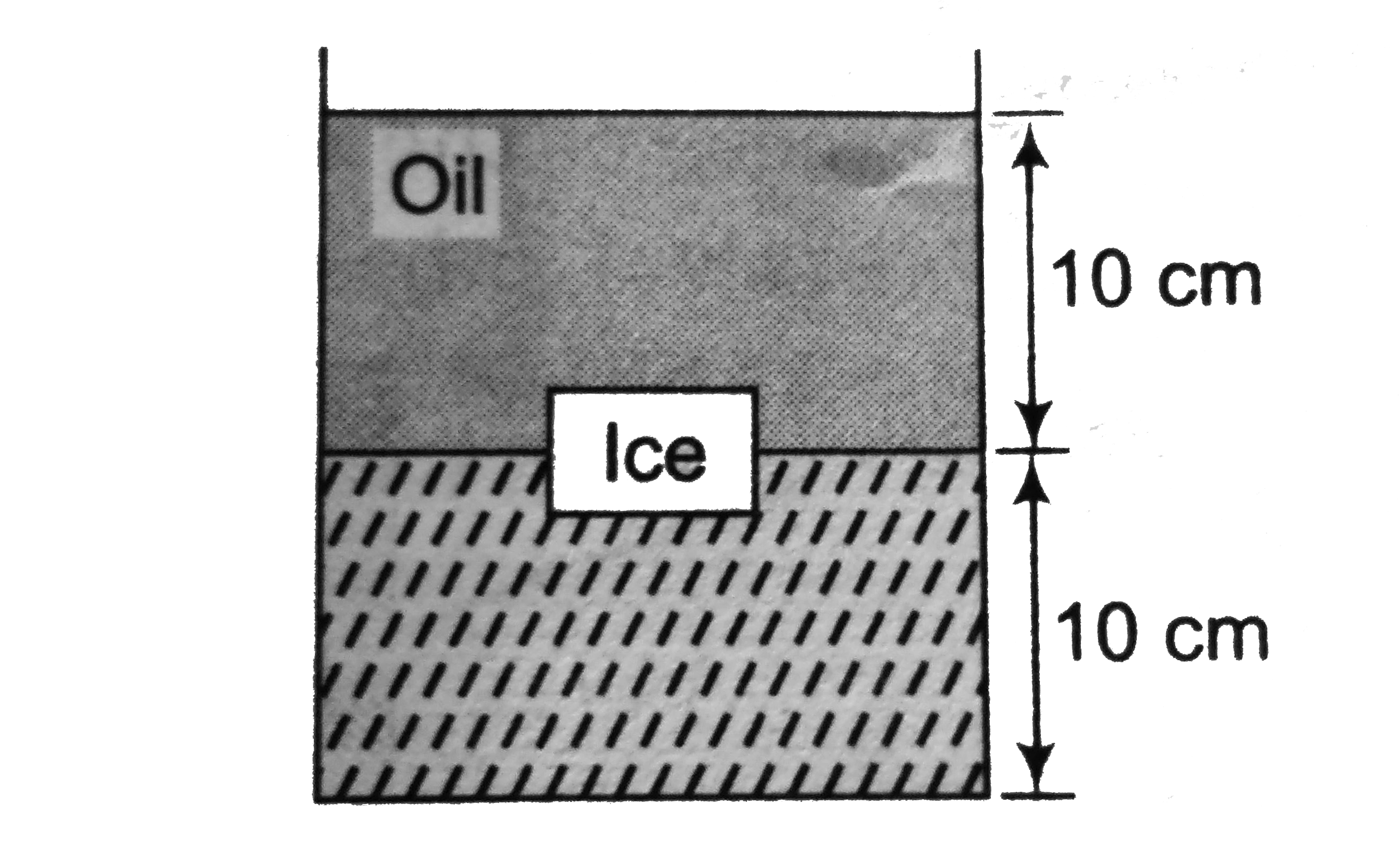
A cubical block of side 10 cm floats at the interface of an oil and water. The pressure above that of atmosphere at the lower face of the block is
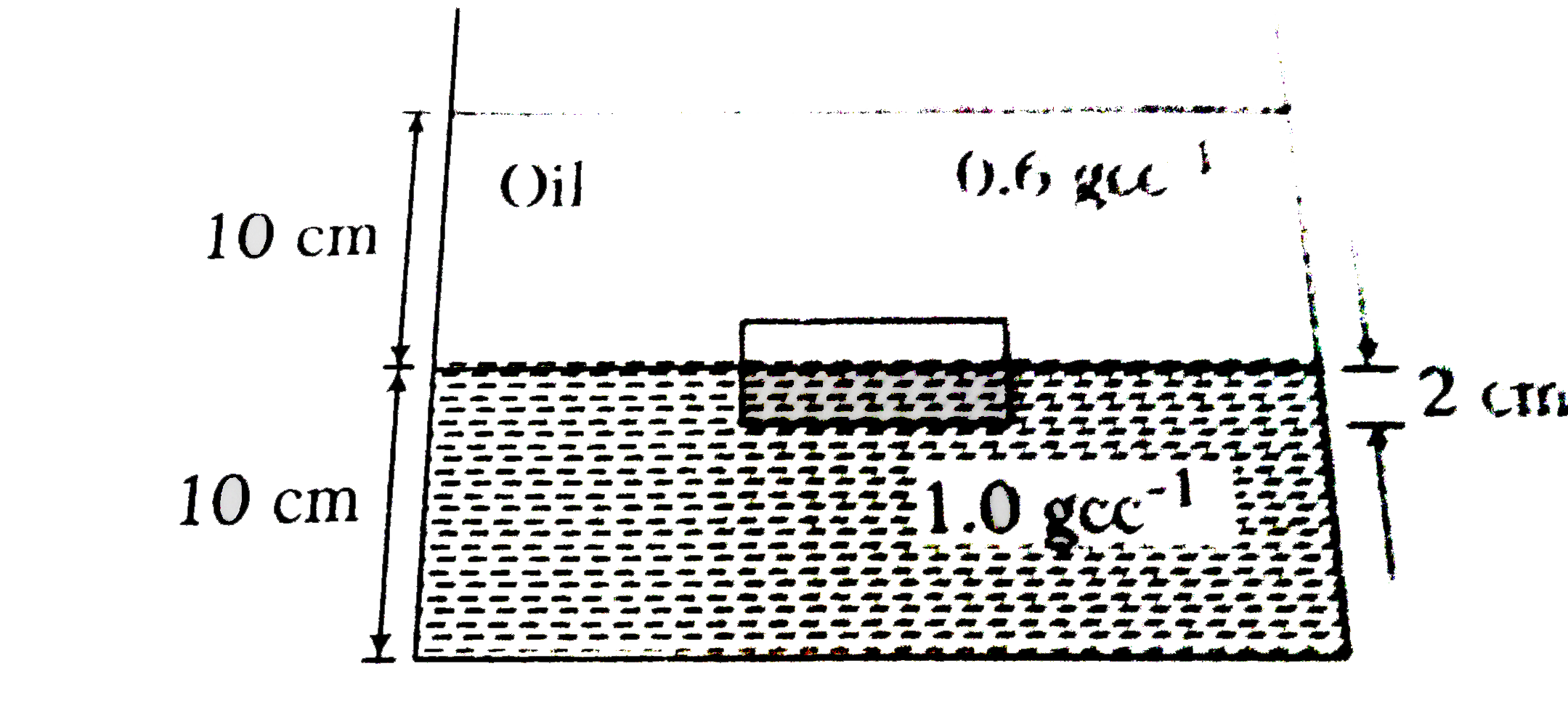
View SolutionA cubical block of side 10cm floats at the interface of an oil and water as shown in the figure. The density of oil is 0.6gcm−3 and the lower face of ice cube is 2cm below the interface. The pressure above that of the atomosphere at the lower face of the block is
View SolutionA cubical block of wood having an edge 10 cm and mass 0.92 kg floats on a tank of water with oil of relative density 0.5 to a height of 4 cm above water. When the block attains equilibrium with four of its edges vertical
View SolutionA block of wood of mass 24 kg floats in water. The volume of wood is 0.032 m. Find the volume of block below the surface of water
View SolutionA block of wood of mass 24 kg floats on water. The volume of wood is 0.032m3 Find
a.the volume of block below the surface of water,
b.the density of wood.
(Denstiy of water =1000kgm−3)
View SolutionA cubical metal block of edge 12 cm floats in mercry with one fifth of the height inside the mercury. Water poured till the surface of the block is just immersed in it. Find the height of the water column to be poured. Specific gravity of mercury =13.6.
View SolutionA cubical block of iron of side13.6cm is floating in mercury in a vessel .Water is poured in the vessel until it just covers the iron block. What is the height of water column? (ρHg=13.6gmcm3, ρFe=7.2gmcm3, ρH_2O=1gmcm3)
View SolutionA cubical block of wood weighing 200 g has a lead piece fastened underneath. Find the mass of the lead piece which will just allow the block of float in water. Specific gravity of wood is 0.8 and that of lead is 11.3.
View SolutionWater and oil are poured into the two limbs of a U-tube containig mercury. The interface of the mercury and the liquids are at the same height in both limbs.
Determine the height of the water columnh1 if that of the oil h2=20cm. The density of the oil is 0.9.
 .
.
View SolutionA cubical box of wood of side 30 cm and mass 21.6 kg floats on water with two faces horizontal. The length of immersed part in water is :
(ρwood=0.8g/cc)
View Solution
