A cubical block of wood having an edge 10 cm and mass 0.92 kg floats on a tank of water with oil of relative density 0.5 to a height of 4 cm above water. When the block attains equilibrium with four of its edges vertical
a. 1 cm of it will be above the free surface of oil
b. 5 cm of it will be under water
c. 1.6 cm of it will be above the comon surface of oil and water
d. 8.4 cm of it will be under water
The correct Answer is:C, D
To solve the problem step by step, we need to analyze the situation involving the cubical block of wood floating in a tank of water with oil on top.
Given Data:
- Edge of the cubical block, a=10cm=0.1m
- Mass of the block, m=0.92kg
- Height of the block above water, hoil=4cm=0.04m
- Relative density of oil, RD=0.5
- Density of water, ρwater=1000kg/m3
- Density of oil, ρoil=RD×ρwater=0.5×1000=500kg/m3
Step 1: Calculate the Volume of the Block
The volume V of the cubical block is given by:
V=a3=(0.1m)3=0.001m3
Step 2: Calculate the Weight of the Block
The weight W of the block is given by:
W=m⋅g=0.92kg⋅9.81m/s2≈9.03N
Step 3: Set Up the Buoyant Force Equation
The buoyant force FB acting on the block is equal to the weight of the fluid displaced. This buoyant force consists of contributions from both the oil and the water.
Let x be the height of the block submerged in oil, and (10cm−x) be the height submerged in water. The total buoyant force can be expressed as:
FB=Foil+Fwater=ρoil⋅g⋅Voil+ρwater⋅g⋅Vwater
Where:
- Voil=A⋅x
- Vwater=A⋅(10cm−x)
Since the area A cancels out, we can simplify the equation to:
FB=ρoil⋅x+ρwater⋅(10cm−x)
Step 4: Substitute Values into the Buoyant Force Equation
Substituting the known values:
FB=(500kg/m3⋅x)+(1000kg/m3⋅(0.1−x))
Setting FB=W:
500x+1000(0.1−x)=9.03
500x+100−1000x=9.03
−500x+100=9.03
−500x=9.03−100
−500x=−90.97
x=90.97500≈0.18194m≈18.19cm
Step 5: Determine the Height of the Block Above the Water Surface
The height of the block submerged in water is:
hwater=10cm−x=10cm−18.19cm=−8.19cm
This indicates that the block is floating with part of it above the water surface.
Step 6: Calculate the Height Above the Common Surface of Water and Oil
Since the block is 4 cm above water, the total height above the common surface of water and oil is:
habove=4cm+(10cm−hwater)
Calculating habove:
habove=4cm+8.19cm=12.19cm
Conclusion
The height of the block above the common surface of the water and oil is approximately 1.6cm.
- Edge of the cubical block, a=10cm=0.1m
- Mass of the block, m=0.92kg
- Height of the block above water, hoil=4cm=0.04m
- Relative density of oil, RD=0.5
- Density of water, ρwater=1000kg/m3
- Density of oil, ρoil=RD×ρwater=0.5×1000=500kg/m3
The volume V of the cubical block is given by:
V=a3=(0.1m)3=0.001m3
The weight W of the block is given by:
W=m⋅g=0.92kg⋅9.81m/s2≈9.03N
The buoyant force FB acting on the block is equal to the weight of the fluid displaced. This buoyant force consists of contributions from both the oil and the water.
FB=Foil+Fwater=ρoil⋅g⋅Voil+ρwater⋅g⋅Vwater
Where:
- Voil=A⋅x
- Vwater=A⋅(10cm−x)
FB=ρoil⋅x+ρwater⋅(10cm−x)
Substituting the known values:
FB=(500kg/m3⋅x)+(1000kg/m3⋅(0.1−x))
Setting FB=W:
500x+1000(0.1−x)=9.03
500x+100−1000x=9.03
−500x+100=9.03
−500x=9.03−100
−500x=−90.97
x=90.97500≈0.18194m≈18.19cm
The height of the block submerged in water is:
hwater=10cm−x=10cm−18.19cm=−8.19cm
This indicates that the block is floating with part of it above the water surface.
Since the block is 4 cm above water, the total height above the common surface of water and oil is:
habove=4cm+(10cm−hwater)
Calculating habove:
habove=4cm+8.19cm=12.19cm
The height of the block above the common surface of the water and oil is approximately 1.6cm.
Topper's Solved these Questions
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - D
2 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - E
8 Videos
- MECHANICAL PROPERTIES OF FLUIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - SECTION - B
23 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
- MECHANICAL PROPERTIES OF SOLIDSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - Assignment(Section-D)
14 Videos
Similar Questions
A cubical box of wood of side 30 cm and mass 21.6 kg floats on water with two faces horizontal. The length of immersed part in water is :
(ρwood=0.8g/cc)
View SolutionThe densit of a block of wood which flots on water with 0.1 of its volume above water is:
View SolutionA block of wood of volume 30cm3 floats it water with 20cm3 of its volume immersed.
Calculate the density.
View SolutionA block of wood of volume 25cm3 floats on water with 20cm3 of its volume immersed. Calculate (i) the density, and (ii) the weight of block of wood.
View SolutionA block of steel of size 5cm×5cm×5cm is weighed in water. If the relative density of steel is 7. its apparent weight is:
View SolutionA rectangular tank having base 15cm×20cm is filled with water (density ρ=1000kg/m3) up to 20cm and force constant K=280N/m is fixed to the bottom of the tank so that the spring remains vertical.
This system is in an elevator moving downwards with acceleration a=2m/s2. A cubical block of side l=10cm and mass m=2kg is gently placed over the spring and released gradually, as shown in the figure.
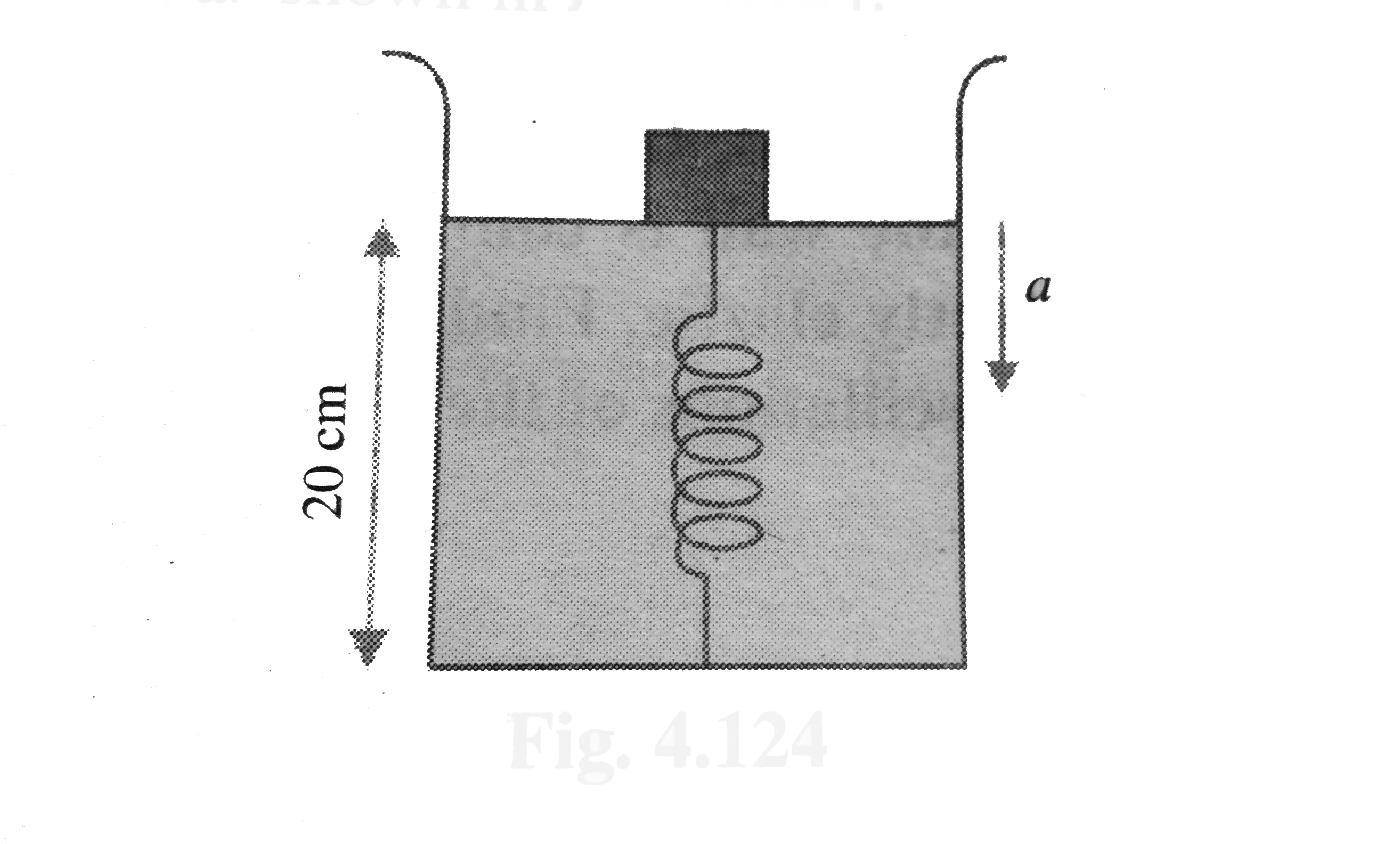
a. Calculate compression of the spring in equilibrium position.
b. If the block is slightly pushed down from equilibrium position and released, calculate frequency of its vertical oscillations.
View SolutionA block of silver of mass 4kg hanging from a string is immersed in a liquid of relative density 0.72. If relative density of silver is 10, then tension in the string will be
View SolutionA block of silver of mass 4kg hanging from a string is immersed in a liquid of relative density 0.72. If relative density of silver is 10, then tension in the string will be
View SolutionA cubical block of side 10 cm floats at the interface of an oil and water. The pressure above that of atmosphere at the lower face of the block is
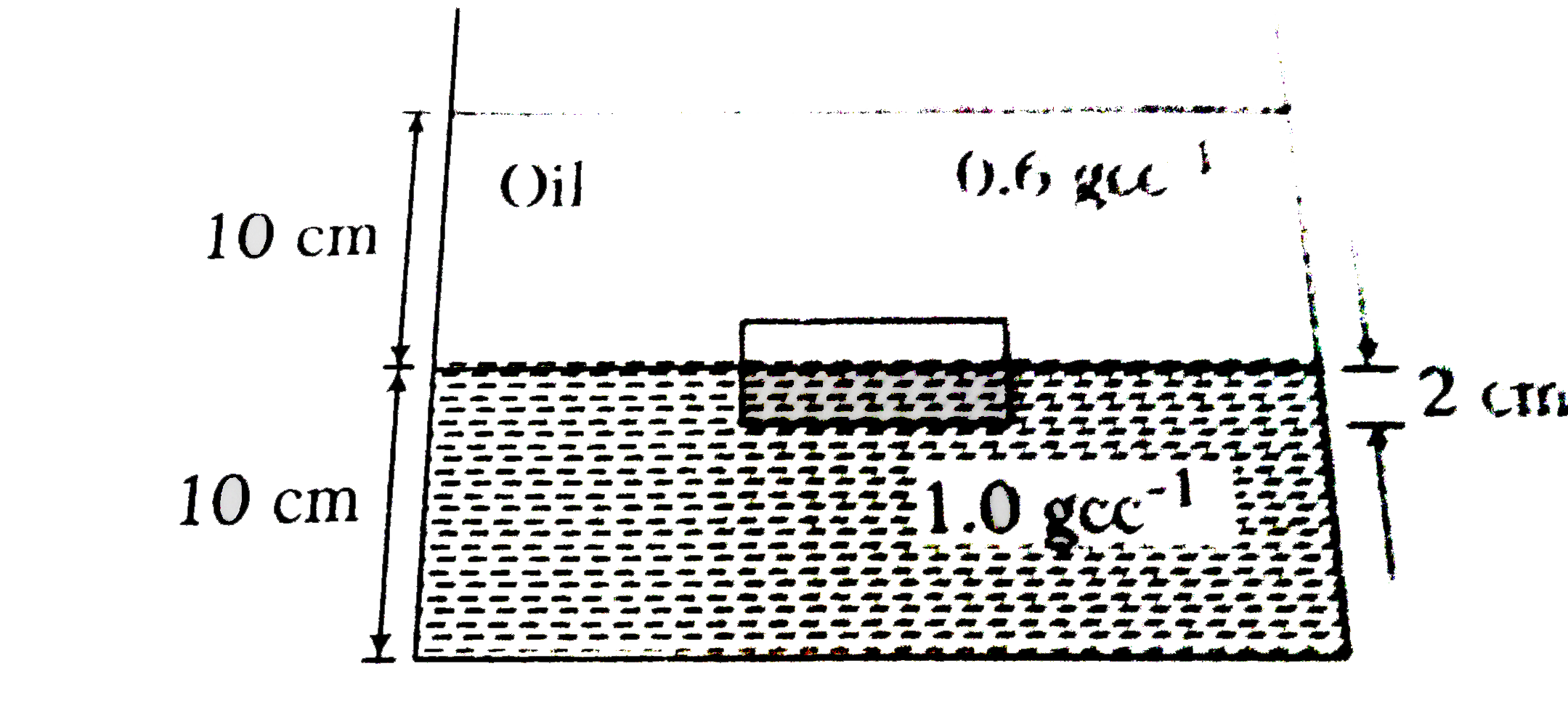
View SolutionA cubical block of wood 10cm along each side floats at the interface between an oil and water with its lowest surface 2cm below the interface. If the height of oil and water columns are 10cm each and ρoil=0.8gcc, find a the mass of the block, b. the liquid pressure at the lower surface of the block.
View Solution
