A cubical box of wood of side 30 cm and mass 21.6 kg floats on water with two faces horizontal. The length of immersed part in water is :
30 cm
12 cm
6 cm
24 cm
The correct Answer is:D
To find the length of the immersed part of a cubical wooden box floating in water, we can follow these steps:
Step 1: Understand the Problem
We have a cubical wooden box with a side length of 30 cm and a mass of 21.6 kg. The box floats on water, and we need to find the length of the part that is immersed in water.
Step 2: Use the Principle of Buoyancy
According to the principle of buoyancy (Archimedes' principle), the weight of the fluid displaced by the submerged part of the object is equal to the weight of the object itself when it is floating.
Step 3: Calculate the Weight of the Wooden Box
The weight (W) of the wooden box can be calculated using the formula:
W=mg
where:
- m=21.6kg
- g=9.81m/s2 (acceleration due to gravity)
Calculating the weight:
W=21.6kg×9.81m/s2=211.6N
Step 4: Calculate the Volume of Water Displaced
Let h be the height of the immersed part of the box in meters. The volume of the displaced water (V) can be expressed as:
V=Area×h
The area of one face of the cube is:
Area=side2=(0.3m)2=0.09m2
Thus, the volume of displaced water becomes:
V=0.09m2×h
Step 5: Relate Weight to Buoyancy Force
The buoyancy force (B) is equal to the weight of the displaced water:
B=ρwater×V×g
where:
- ρwater=1000kg/m3
Substituting for V:
B=1000kg/m3×(0.09m2×h)×9.81m/s2
Step 6: Set Weight Equal to Buoyancy Force
Setting the weight equal to the buoyancy force:
211.6N=1000×0.09×h×9.81
Step 7: Solve for h
Rearranging the equation to solve for h:
h=211.61000×0.09×9.81
Calculating the denominator:
1000×0.09×9.81=882.9
Thus,
h=211.6882.9≈0.239m
Step 8: Convert to Centimeters
To convert h from meters to centimeters:
h≈0.239m×100=23.9cm
Step 9: Final Answer
Rounding to the nearest whole number, the length of the immersed part in water is approximately:
Length of immersed part≈24cm
Conclusion
Thus, the length of the immersed part of the wooden box is 24 cm.
---
We have a cubical wooden box with a side length of 30 cm and a mass of 21.6 kg. The box floats on water, and we need to find the length of the part that is immersed in water.
According to the principle of buoyancy (Archimedes' principle), the weight of the fluid displaced by the submerged part of the object is equal to the weight of the object itself when it is floating.
The weight (W) of the wooden box can be calculated using the formula:
W=mg
where:
- m=21.6kg
- g=9.81m/s2 (acceleration due to gravity)
W=21.6kg×9.81m/s2=211.6N
Let h be the height of the immersed part of the box in meters. The volume of the displaced water (V) can be expressed as:
V=Area×h
The area of one face of the cube is:
Area=side2=(0.3m)2=0.09m2
V=0.09m2×h
The buoyancy force (B) is equal to the weight of the displaced water:
B=ρwater×V×g
where:
- ρwater=1000kg/m3
B=1000kg/m3×(0.09m2×h)×9.81m/s2
Setting the weight equal to the buoyancy force:
211.6N=1000×0.09×h×9.81
Rearranging the equation to solve for h:
h=211.61000×0.09×9.81
Calculating the denominator:
1000×0.09×9.81=882.9
Thus,
h=211.6882.9≈0.239m
To convert h from meters to centimeters:
h≈0.239m×100=23.9cm
Rounding to the nearest whole number, the length of the immersed part in water is approximately:
Length of immersed part≈24cm
Thus, the length of the immersed part of the wooden box is 24 cm.
Topper's Solved these Questions
- RACEBook:ALLENChapter:RACEExercise:Basic Maths (Properties of Matter & Fluid Mechanics)(Surface Tension)
Explore 26 Videos
- RACEBook:ALLENChapter:RACEExercise:Basic Maths (Thermal Physics) (Temperature scales & thermal expansion)
Explore 13 Videos
- RACEBook:ALLENChapter:RACEExercise:Basic Maths (Properties of Matter & Fluid Mechanics)(Fluid Dynamics + Viscosity)
Explore 18 Videos
- NEWTONS LAWS OF MOTIONBook:ALLENChapter:NEWTONS LAWS OF MOTIONExercise:EXERCISE-III
Explore 28 Videos
- SIMPLE HARMONIC MOTIONBook:ALLENChapter:SIMPLE HARMONIC MOTIONExercise:Example
Explore 1 Video
Similar Questions
A cubical block of wood having an edge 10 cm and mass 0.92 kg floats on a tank of water with oil of relative density 0.5 to a height of 4 cm above water. When the block attains equilibrium with four of its edges vertical
View SolutionA block of wood of volume 30cm3 floats in water with 20cm3 of its volume immersed.
Calculate the weight of the block of wood .
View SolutionA block of wood of volume 30cm3 floats it water with 20cm3 of its volume immersed.
Calculate the density.
View SolutionA block of wood of mass 24 kg floats in water. The volume of wood is 0.032 m. Find the volume of block below the surface of water
View SolutionA cubical block of side 10 cm floats at the interface of an oil and water. The pressure above that of atmosphere at the lower face of the block is
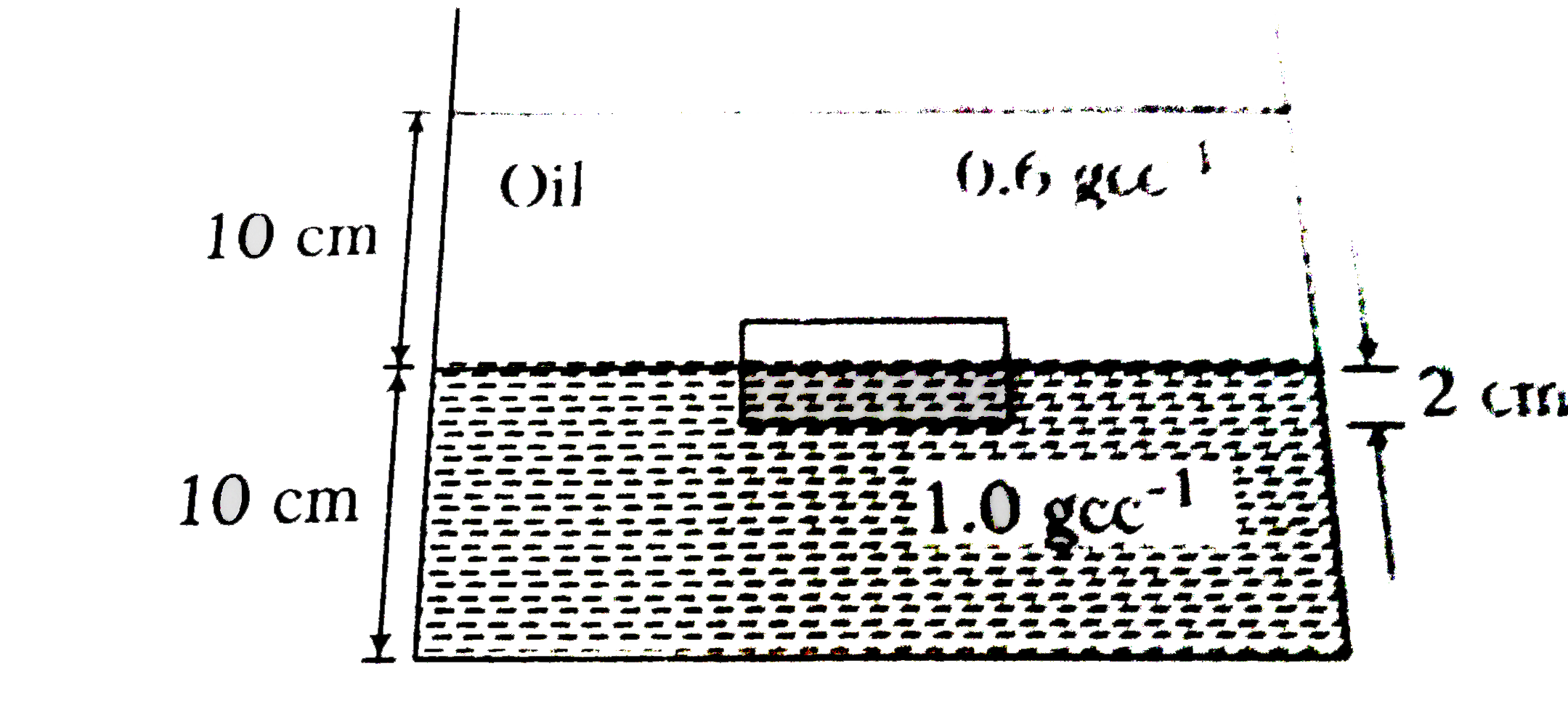
View SolutionA block of wood of mass 24 kg floats in water. The volume of wood is 0.032 m^3. Find the density of wood. (Density of water = 1000kg m−3 )
View SolutionA wooden cube of side 10 cm has mass 700 g. What part of it remains above the water surface while floating vertically on water surface?
View SolutionIf 2 cm3 of wood has a mass 0.6 g, what would be its density?
View SolutionA raft of wood (density=600kg/m3) of mass 120kg floats in water. How much weight can be put on the raft to make it just sink?
View SolutionA log of wood of mass 120 Kg floats in water. The weight that can be put on the raft to make it just sink, should be (density of wood =600Kg/m)
View Solution
