A cylindrical tank of height H is open at the top end and it has a radius r. Water is filled in it up to a height of h. The time taken to empty the tank through a hole of radius r' in its bottom is
√2hgr2r'2
√2Hgr2r'2
√hH
none of these
The correct Answer is:A
To solve the problem of determining the time taken to empty a cylindrical tank through a hole at the bottom, we can follow these steps:
Step 1: Understand the Setup
We have a cylindrical tank of height H and radius r, filled with water up to a height h. There is a hole at the bottom of the tank with radius r′. We need to find the time taken to empty the tank through this hole.
Step 2: Apply the Principle of Continuity
According to the principle of continuity, the flow rate of water out of the hole must equal the rate at which the water level in the tank decreases.
Let:
- A1=πr2 (cross-sectional area of the tank)
- A2=π(r′)2 (cross-sectional area of the hole)
The flow rate out of the hole can be expressed as:
A2v2=π(r′)2v2
where v2 is the velocity of water exiting the hole.
Step 3: Apply Torricelli’s Law
The velocity v2 of water exiting the hole can be derived from Torricelli’s law:
v2=√2gh
where g is the acceleration due to gravity and h is the height of water above the hole.
Step 4: Relate the Change in Height to Time
The rate of change of height of water in the tank can be expressed as:
dhdt=−A2v2A1
Substituting for A1 and A2:
dhdt=−π(r′)2√2ghπr2=−(r′)2√2ghr2
Step 5: Rearranging and Integrating
Rearranging gives:
dt=−r2(r′)2√2gdh√h
Now, we integrate both sides. The limits for h will go from h to 0 and for t from 0 to T (the total time to empty the tank):
T=−r2(r′)2√2g∫0hdh√h
Step 6: Solve the Integral
The integral ∫dh√h evaluates to −2√h. Thus:
T=−r2(r′)2√2g(−2√h)0h=2r2(r′)2√2g√h
Final Result
The time taken to empty the tank is given by:
T=2r2(r′)2√2g√h
We have a cylindrical tank of height H and radius r, filled with water up to a height h. There is a hole at the bottom of the tank with radius r′. We need to find the time taken to empty the tank through this hole.
According to the principle of continuity, the flow rate of water out of the hole must equal the rate at which the water level in the tank decreases.
- A1=πr2 (cross-sectional area of the tank)
- A2=π(r′)2 (cross-sectional area of the hole)
A2v2=π(r′)2v2
where v2 is the velocity of water exiting the hole.
The velocity v2 of water exiting the hole can be derived from Torricelli’s law:
v2=√2gh
where g is the acceleration due to gravity and h is the height of water above the hole.
The rate of change of height of water in the tank can be expressed as:
dhdt=−A2v2A1
Substituting for A1 and A2:
dhdt=−π(r′)2√2ghπr2=−(r′)2√2ghr2
Rearranging gives:
dt=−r2(r′)2√2gdh√h
Now, we integrate both sides. The limits for h will go from h to 0 and for t from 0 to T (the total time to empty the tank):
T=−r2(r′)2√2g∫0hdh√h
The integral ∫dh√h evaluates to −2√h. Thus:
T=−r2(r′)2√2g(−2√h)0h=2r2(r′)2√2g√h
The time taken to empty the tank is given by:
T=2r2(r′)2√2g√h
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Multipe Correct15videos
FLUID MECHANICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Assertion-Reasoning8videos
FLUID MECHANICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective25videos
FLUID MECHANICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer2videos
DIMENSIONS & MEASUREMENT
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE1videos
GRAVITATION
Similar Questions
A cylinderical tank of height 0.4 m is open at the top and has a diameter 0.16m. Water is filled in it up to height of 0.16 m. Find the time taken to empty the tank through a hole of radius 5×10−3m in its bottom.
View SolutionA cylindrical tank of height 2 m is open at the top and has a radius 50 cm. Water is filled in it upto a height 1.75 m. Calculate how long it will take to empty the tank through a hole of radius 0.05 cm in its bottom.
View Solution
Knowledge Check
- Question 1 - Select One
A cylindrical vessel of height 500mm has an orifice (small hole) at its bottom. The orifice is initially closed and water is filled in it up to height H. Now the top is completely sealed with a cap and the orifice at the bottom is opened. Some water comes out from the orifice and the water level in the vessel becomes steady with height of water column being 200mm. Find the fall in height(in mm) of water level due to opening of the orifice.
[Take atmospheric pressure =1.0×105N/m2, density of water=1000kg//m^3andg=10m//s^2. Neglect any effect of surface tension.]A6B4C2D8
T=R2r2√2hg
Suppose an open cylindrical tank has a round drain with radius t in the bottom of the tank. When the tank is filled with water to a depth of h centimeters, the time it takes for all the water to drain from the tank is given by the formula above, where R is the radius of the tank (in centimeters) and g=980cm/s2 is the acceleration due to gravity. Suppose such a tank has a radius of 2 meters and is filled to a depth of 4 meters. About how many minutes does it take to empty the tank if the drain has a radius of 5 centimeters? (1 meter=100 centimeter).View SolutionA body has a weight 72 N. When it is taken to a height h=R= radius of earth, it would weight
View SolutionA cylindrical tank full of water is emptied by a pipe at the rate of 225 litres per minute. How much time will it take to empty half the tank, if the diameter of its base is 3 m and its height is 3.5 m? [Use π=22/7 ]
View SolutionAn observer can see through a pin-hole the top end of a thin rod of height h, placed as shown in the figure. The beaker height is 3h and its radius h. When the beaker is filled with a liquid up to a height 2h, he can see the lower end of the rod. Then the refractive index of the liquid is
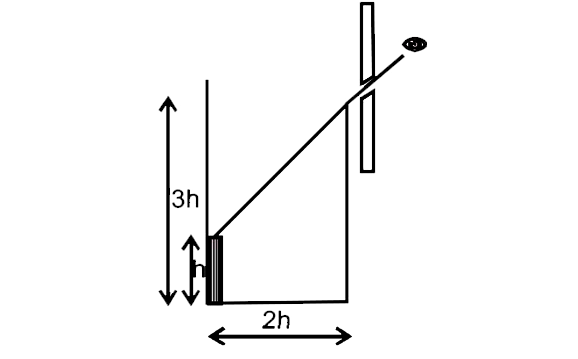 View Solution
View SolutionA tank has a hole at its bottom. The time needed to empty the tank from level h1 to h2 will be proportional to
View SolutionThere is a hole at the bottom of a large open vessel. If water is filled upto a height h, it flows out in time t. if water is filled to a height 4h, it will flow out in time
View SolutionThe flat bottom of cylinder tank is silvered and water (μ=43) is filled in the tank upto a height h. A small bird is hovering at a height 3h from the bottom of the tank. When a small hole is opened near the bottom of the tank, the water level falls at the rate of 1 cm/s. The bird will perceive that his velocity of image is 1/x cm/sec (in downward directions) where x is
View Solution

